Calculating Induced EMF in Moving Wire

[JSGandora]

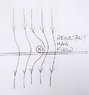
In the classic problem of the induced EMF in a moving conductor (as in the picture above), the calculation for the induced EMF is as follows

E=\frac{d\Phi_B}{dt}=\frac{d(BA)}{dt}=\frac{Bd(A)}{dt}=\frac{Bd(Lx)}{dt}=\frac{BLdx}{dt}=BLv.

The derivation assumes that the magnetic field B is constant throughout the entire time the flux is changing. I am confused because it does not seem like the magnetic field is constant because while there is an induced EMF in the wire, there must also be self induction that occurs (since the induced current in the coil also produces a magnetic field) thereby changing the already present magnetic field. So in fact the magnetic field is a function of time inside the coil of wire. Is the self induction so insignificant that the calculation can assume that the magnetic field does not change?

 

[cabraham]

If the rod and loop have a high resistance value, greater than inductive reactance, then self inductance need not be considered. But if both the loop and rod have a low resistance value, so that X_L is greater than or close in value to R, then we cannot ignore self inductance.

You're thinking the right way. For a more detailed explanation, I would review an electric machines text. Here are my references I used in college/graduate school.

Leander Matsch - "Electromagnetic & Electromechanical Machines"

Fitzgerald, Kingsley, Umans - "Electric Machinery"

Dr. Umans was my professor in spring 2010 when I took his power and machines course. He teaches at MIT, and he was lecturing at Case in Cleveland where I am a Ph.D. student. He's very good.

Incidentally that problem appeared on my Ph.D. qualifier written exam, and I gave the same answer you did. I computed the induction due to the external field, then computed self inductance. I got it right and passed the exam. There were many other problems as well.

Claude

 

[technician]

If the rod and loop have a high resistance value, greater than inductive reactance, then self inductance need not be considered. But if both the loop and rod have a low resistance value, so that X_L is greater than or close in value to R, then we cannot ignore self inductance.

You're thinking the right way. For a more detailed explanation, I would review an electric machines text. Here are my references I used in college/graduate school.

Leander Matsch - "Electromagnetic & Electromechanical Machines"

Fitzgerald, Kingsley, Umans - "Electric Machinery"

Dr. Umans was my professor in spring 2010 when I took his power and machines course. He teaches at MIT, and he was lecturing at Case in Cleveland where I am a Ph.D. student. He's very good.

Incidentally that problem appeared on my Ph.D. qualifier written exam, and I gave the same answer you did. I computed the induction due to the external field, then computed self inductance. I got it right and passed the exam. There were many other problems as well.

Claude

Can you make clear what you mean by X_L and R
What is the value of the induced emf if it is not Blv?...you do realize that this is the standard textbook answer...is it wrong?
What answer did your research come up with?

 

[cabraham]

Can you make clear what you mean by X_L and R
What is the value of the induced emf if it is not Blv?...you do realize that this is the standard textbook answer...is it wrong?
What answer did your research come up with?

X_L = Lω. The value of the "induced emf" is often taken as "Blv", which is the induced emf due to external flux. The general approach is to take the distributed resistance and inductance of the loop and lump them into R & L. The induced emf equals the open circuit voltage, Voc, since the open circuit condition has very little current, hence self inductance is negligible.

When loaded (loop closed), the equivalent circuit is a voltage source of Voc, plus an inductive reactance X_L, plus resistance R. Current I is computed using circuit theory. The impedance Z is just R + jX_L, or its magnitude is |Z| = √R² + X_L². |I| =Voc/|Z|.

As far as "Blv" being the "standard textbook answer" goes, not in the textbooks I referenced is that the answer. Any machines book will affirm my answer. I believe we spoke on this subject recently. Are you familiar with ac circuit theory? Do you know reactance as well as resistance. In dc theory, V = I*R, but with ac we must use V = I*Z, where Z = R + jX. Did I help.

Claude

 

[Philip Wood]

CAB: I agree with the general thrust of your answer, but don't like your use of X_L = \omega L, because this is only applicable when a sinusoidal current is flowing. In the case of an arbitrary movement of the rod on the rails we have to use the more general term E_{back} = - \frac{d\widetilde{\Phi}}{dt} in which \widetilde{\Phi} is the flux due to the induced current, if any. This emf acts in the opposite direction to BLv (in which B is the externally applied field), and doesn't act at all if the circuit is open.

[Original post corrected.]

 

[technician]

The value of the "induced emf" is often taken as "Blv",

Yhe induced emf is always taken to be Blv. If the induced emf = Blv and an induced current arises (because of a complete circuit of resistance R) then the current is Blv/R.
The magnetic field around the moving wire resulting from the induced current does not change the value of the original field. The moving wire with its associated field is moving through the applied field. There is now a Force acting on the wire and this should be apparent on some diagrams I am attaching.
The equations that have been given in an earlier post relate to AC circuit theory, not this aspect of physics...ω has no meaning in this problem.

 

[Philip Wood]

Technician. You're right about the meaningless of \omega of course, and I'd now argue that even L is pretty meaningless, because the circuit's shape keeps changing, but I don't agree that the induced emf is simply BLv, when there is a current flowing - if by B you mean the externally applied field.

 

[technician]

Technician. You're right about the meaningless of \omega of course, and I'd now argue that even L is pretty meaningless, because the circuit's shape keeps changing, but I don't agree that the induced emf is simply BLv, when there is a current flowing - if by B you mean the externally applied field.

First of all, let's be clear...when I say 'I' I mean my references...text books, past exam papers and answers etc...I am not inventing Faradays laws!
The (every !) textbook answer is that E = Blv and yes...B is the externally applied field. If you have some (text book or other) reference that this is not correct then we all need to know and we can disregard the textbooks that state it to be the case.
I think I am correct in saying that the wire does not cut through the lines of force created by the induced current created by the induced emf given by E = Blv... so this has no effect on E.?
We cannot progress in this thread without this being cleared up.

 

[vanhees71]

In this simple case you can (with overwhelming accuracy) neglect self-inductance and just use Farday's Law in integral form, which reads (note that this is not accurate in many textbooks, but it's pretty well described in Wikipedia)
\mathcal{E}=\int_{\partial A} \mathrm{d} \vec{x} \cdot [\vec{E}+\vec{v} \times \vec{B}]=-\frac{\mathrm{d}}{\mathrm{d} t} \int_{A} \mathrm{d} \vec{F} \cdot \vec{B}.
Let's take the plane of the circuit as the xy plane of a Cartesian coordinate system with the moving rod in y direction.

Let's take the area as given by the circuit and let's integrate counter clockwise. Then the area element is \mathrm{d} \vec{F} =\vec{e}_z \mathrm{d} x \mathrm{d} y. Then tha appropriate magnetic flux thus is
\Phi_A=\int_{A} \mathrm{d} \vec{F} \cdot \vec{B}=-B L (x_0+v t).
Here L is the length of the rod and (x_0+v t) the momentary length of the other wires perpendicular to it.

The left-hand side of the equation, i.e., the line integral, only gives a net contribution for integrating along the pieces of the circuit along the y axis. For the piece at rest you have \vec{v}(\vec{x})=0 and the integral over the electric field across the resistor gives R I (with the sign according to the direction of the current given in the figure).

For the moving piece, if assumed as an ideal conductor there must be no net electric field, i.e, you have \vec{E}+\vec{v} \times \vec{B}=0. Note that there is an electric field along the wire such that
\vec{E}=-\vec{v} \times \vec{B}=-v \vec{e}_x \times (-B \vec{e}_z)=-v B \vec{e}_y
such that there is no net electric field in the rest frame of the moving rod!

This finally leads to
\mathcal{E}=R I=-\frac{\mathrm{d} \Phi_A}{\mathrm{d} t}=B L v.
Note that this calculation only analyzes the stationary state, i.e., the rod should have moved with constant velocity through the homogeneous magnetic field for some time before such that initial-state ("transient") effects can be negelected.

 

[cabraham]

CAB: I agree with the general thrust of your answer, but don't like your use of X_L = \omega L, because this is only applicable when a sinusoidal current is flowing. In the case of an arbitrary movement of the rod on the rails we have to use the more general term E_{back} = - \frac{d\widetilde{\Phi}}{dt} in which \widetilde{\Phi} is the flux due to the induced current, if any. This emf acts in the opposite direction to BLv (in which B is the externally applied field), and doesn't act at all if the circuit is open.

[Original post corrected.]

But any waveform can be resolved into a sum of sine and cosine functions. Even a non-periodic waveform has a Fourier transform. This seems to annoy everybody. Induction motors are a prime example of induction taking place in a loop with very low R value.

If induced emf was constant independent of load such as self inductance, then the power would increase w/o limit as R decreases. We already know that as the rotor R value decreases, power in heating said rotor increases until R equals X_L. Then as R drops below X_L, rotor heat goes DOWN. This is due to self inductance.

Thus for ac domain, I = Voc/(√R² + X_L²). For R << X_L, I = Voc/R.

In your example, if open circuit voltage is "Blv", with the loop resistance of R and inductance L, we cannot ignore L unless we know it is << RT, where T is pulse width. We discussed a transient case in another thread where I included time constants. If L is small, and the induced voltage waveform is a rectangular pulse, that is L/R time constant << T (pulse width), we can assume I = Voc/R. But if L/R approaches or exceeds T, then, I = (Voc/R)(1 - e^-Rt/L).

Here L is increasing as loop area increases. If the track was infinite in length, we would need to compute the Voc waveform. A ramp would result for a dc flux value. The ramp is a constant times "t" (time). The slope of this ramp must be compared to R/L. If slope << R/L, then L will influence the value of I. Later tonight after work, I can compute the infinite track case, slope, then compute L/R constant and its influence.

In technician's sketches, please refer to the current in the rod and associated magnetic field. The rod current is part of the loop which includes the track and closed end as well. Using right hand rule, the flux due to current is oriented exactly opposite to external flux. I will draw a sketch for that as well.

Motor/generator textbooks laid this issue to rest in 19th century. The current I is not simply Voc/R. It could be for small L value, but in general L cannot be ignored in loops with very low R. In this example the track and rod are metal such that R is very low. L comes into play in these scenarios. More later.

Claude

 

[cabraham]

First of all, let's be clear...when I say 'I' I mean my references...text books, past exam papers and answers etc...I am not inventing Faradays laws!
The (every !) textbook answer is that E = Blv and yes...B is the externally applied field. If you have some (text book or other) reference that this is not correct then we all need to know and we can disregard the textbooks that state it to be the case.
I think I am correct in saying that the wire does not cut through the lines of force created by the induced current created by the induced emf given by E = Blv... so this has no effect on E.?
We cannot progress in this thread without this being cleared up.

Induction does not require that force lines be cut. The original induction due to external mag field is due to a changing flux due to changing area. B is constant, but ∅ is changing, as ∅=AB. The loop current is I, and N=1 since the loop has but 1 turn. So ∅ = Li. Then d∅/dt = d(Li)/dt = idL/dt + Ldi/dt, since L changes it must be included in the derivative. So we get a 1st order diff eq in i, with time varying coefficient. I will solve and post tonight. This is not a trivial problem.

Do not make the assumption that L can be ignored. Depending on the values of the parameters, sometimes L can be ignored. But I prefer to compute the solution accounting for L, then if the numbers allow, I can drop L from further consideration since it changes the answer very little. But it's always better to take it into account, because you can see how much error is incurred by dropping it. BR.

"Every textbook" does not say what you say, all of mine do not. Again, I've said this a lot, but machine texts are the horse's mouth on this subject. Study synchronous and induction ac motors, and inductance is always included, both stator and rotor. To compute current, the open circuit voltage is divided by the total impedance, R + jX_L. The texts I mentioned are a good starting point.

Claude

 

[Jackson Lee]

Technician. You're right about the meaningless of \omega of course, and I'd now argue that even L is pretty meaningless, because the circuit's shape keeps changing, but I don't agree that the induced emf is simply BLv, when there is a current flowing - if by B you mean the externally applied field.

Admittedly the shape of the loop is changing, but the inductance reactance always exist. I think it is meaningful.