- #1
Mutaja
- 239
- 0
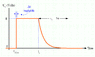
Charging/discharging of capacitor.
Attached to this post, I have two circuits. The left, circuit 1. The right, circuit 2.
For circuit 1 I'm supposed to compute T (tau). Find the expression for Uc1(t) and IR1(t). This is regarding discharging of a capacitor.
For circuit 2, I'm supposed to compute T, Uc1(t) and Uc1(∞)
For circuit 1:
Doing this for a series circuit is straight forward. For parallel circuits, from whatever I can see in my notes and my book, I'm supposed to convert this circuit into a thevenin equivalent. When I do that, using C1 as load, pressing in the switch and other wise use my knowledge about thevenin equivalent circuits, I get R to be 0. When V1 is short circuited, the "current" will flow through the resistance free path, avoiding R1.
How to I solve this?
And my mathematical expressions for Uc1 and IR1, would they differ any from what I have from a series circuit (identical to circuit 1 here, only R1 and C1 are in series)
There I have:
T = RC = 4700Ω * 680*10-6F = 3.196S
UC1 = V1*(1-e-t/Tau) = 8v*(1-e-t/3.196s)
IR1 = V1*e-t/Tau = 8v*e-t/3.196s
Homework Statement
Attached to this post, I have two circuits. The left, circuit 1. The right, circuit 2.
For circuit 1 I'm supposed to compute T (tau). Find the expression for Uc1(t) and IR1(t). This is regarding discharging of a capacitor.
For circuit 2, I'm supposed to compute T, Uc1(t) and Uc1(∞)
Homework Equations
The Attempt at a Solution
For circuit 1:
Doing this for a series circuit is straight forward. For parallel circuits, from whatever I can see in my notes and my book, I'm supposed to convert this circuit into a thevenin equivalent. When I do that, using C1 as load, pressing in the switch and other wise use my knowledge about thevenin equivalent circuits, I get R to be 0. When V1 is short circuited, the "current" will flow through the resistance free path, avoiding R1.
How to I solve this?
And my mathematical expressions for Uc1 and IR1, would they differ any from what I have from a series circuit (identical to circuit 1 here, only R1 and C1 are in series)
There I have:
T = RC = 4700Ω * 680*10-6F = 3.196S
UC1 = V1*(1-e-t/Tau) = 8v*(1-e-t/3.196s)
IR1 = V1*e-t/Tau = 8v*e-t/3.196s
Attachments
Last edited: