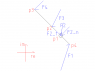
- #1
magwas
- 125
- 0
Homework Statement
I have came up with an example to illustrate my question.
There is a rod, which can turn around p1.
p1p2 = (-1+j) m
p1p3 = (-3 + 3j) m
p1p4 = (1 - j ) m
F1 = (1+3j) N
F3 = (-1 - 2j ) N
F4 = unknown, orthogonal to the rod
compute F2_n, orthogonal component of F2 to the rod
compute F2_t, parallel component of F2 to the rod
Homework Equations
The question is actually here:
The sum of moments is
[tex]\sum{\vec{F} \times \vec{l}} =0[/tex]
Where
[tex]a \times b = \Re{a} \Im{b} - \Im{a} \Re{b}[/tex]
Is that true?
Likewise, the force components parallel to the rod is:
[tex]\sum{\vec{F} \cdot \hat{\vec{l}}} = 0[/tex]
where
[tex] a \cdot b = a \overline{b} + b \overline{a} = 2 \Im{a} \Im{b} + 2 \Re{a} \Re{b}[/tex]
Is it correct?
The Attempt at a Solution
I write the moments around p3. I sum here because:
- all forces are on the same side of the turning point
- all arms are measured towards the turning point (this is why p1p3 - p1p4)
- the direction of forces are encoded in their vectors
so the equation for moments:
[tex] F_{1} \times \left(p1p3 - p1p4\right) + F_{3} \times \left(p1p3 - p1p2\right) + p1p3 \times \left \frac{\mathbf{\imath} p1p3}{\lvert{p1p3}\rvert} \lvert F_{2_{n}}\rvert} = $\\
$
\Im{p1p3} \Im\left(\frac{\lvert F_{2_{n}}\rvert p1p3}{\lvert{p1p3}\rvert}\right) + \Im\left(p1p3 - p1p2\right)
\Re{F_{3}} + \Im\left(p1p3 - p1p4\right) \Re{F_{1}} + \Re{p1p3} \Re
\left(\frac{\lvert F_{2_{n}}\rvert p1p3}{\lvert{p1p3}\rvert}\right) - \Im{F_{1}} \Re\left(p1p3 - p1p4\right) -
\Im{F_{3}} \Re\left(p1p3 - p1p2\right) = $\\
$
10.0 + 4.24264068711929 \lvert F_{2_{n}} \rvert = 0[/tex]
so
[tex]\lvert F_{2_{n}}\rvert =-2.3570226039551 [/tex] which gives
[tex]F_{2_{n}} = \lvert F_{2_{n}}\rvert \frac{\mathbf{\imath} p1p3}{\lvert{p1p3}\rvert} = 1.66666666666667 + 1.66666666666667 \mathbf{\imath}[/tex]
Now the forces parallel to the rod:
We use our unit vector [tex]\hat{l} = \frac{p1p3}{\lvert{p1p3}\rvert}[/tex]
, and forget F4 as it is orthogonal to the rod, so the sum:
[tex] F_{3} \cdot \hat{l} + \lvert F_{2_{t}}\rvert \cdot \hat{l} + F_{1} \cdot \hat{l} = $\\
2 \lvert F_{2_{t}}\rvert \Re{\hat{l}} + 2 \Im{F_{1}} \Im{\hat{l}} + 2 \Im{F_{3}} \Im{\hat{l}} +
2 \Re{F_{1}} \Re{\hat{l}} + 2 \Re{F_{3}} \Re{\hat{l}} = $\\
1.4142135623731 - 1.4142135623731 \lvert F_{2_{t}}\rvert = 0 [/tex]
so
[tex]\lvert F_{2_{t}}\rvert = 1[/tex]
which gives
[tex] F_{2_{t}} = -0.707106781186548 + 0.707106781186548 \mathbf{\imath} [/tex]
and
[tex] F_{2} = F_{2_{n}} + F{2_{t}} = 0.959559885480119 + 2.37377344785321 \mathbf{\imath}[/tex]
Attachments
Last edited: