Electronics: Find Thevenin equivalent voltage & resistance, etc.

[oddjobmj]

Homework Statement

http://imgur.com/w5AQ1A8

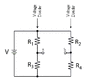
As shown in the image:

In the circuit below R₁=100Ω, R₂=1000Ω, R₃=99Ω, R₄=1000Ω, R₅=10Ω, and V=10V.

(a) Relying on the Thevenin theorem, find the equivalent voltage and equivalent resistance for the circuit below when the resistor R₅ is taken out.

(b) What is the current through R₅ when it is put back into the circuit?

(c) If R₅=10kΩ were put in instead of 10Ω, what would be the current through it?

(d) Calculate the voltage across R₅ for the conditions in (b) and (c), respectively.

Homework Equations

V=IR
Parallel Resistors=$\frac{1}{\frac{1}{R_a}+\frac{1}{R_b}}$
Series Resistors=R_a+R_b

The Attempt at a Solution

I am having a hard time with Thevenin equivalent circuits. The problem doesn't seem to make it clear where my terminals should be. If all I have to do is find the equivalent resistor of R1 and R3 in series, R2 and R4 in series, and those equivalent resistors in parallel then part (a) should be simple. I'm not sure if it matters where the terminals are.

If that is the case I get R_TH≈181Ω and V_TH would just be V=30V.

For part (b) I have to start over it seems. I have no idea how to do this without resorting to other methods such as meshes. I have a feeling I can simplify this circuit in a useful way using Thevenin's theorem but how I do that and still know where R5 comes in I don't know.

Suggestions? Thank you for your help.

EDIT: I was able to plug this into multism to find the answers to (b), (c), and (d) so I can check my work. I do need to understand this and also show my work though.

(b) 45.296 μA
(c) 45.296 μA (same?)
(d) V₁₀=448.873 μV, V_10k=23.816 mV

EDIT 2:

Hah! Just realized how badly I botched the title. *facepalm*

 

[phinds]

I am having a hard time with Thevenin equivalent circuits. The problem doesn't seem to make it clear where my terminals should be.

Yes, it does. Clearly R5 is to be considered the load resistor and the equivalent circuit is to be calculated around the terminals at R5 (and of course, with R5 not in the circuit). Once you have the equivalent circuit, part b is trivial as are the other questions.

 

[oddjobmj]

That definitely makes sense, thank you. I was worried you would say that because I don't know where to start in that case. I'll go back through some more examples with your clarification in mind. Hopefully something clicks.

Once you have the equivalent circuit, part b is trivial as are the other

That is good to hear!

EDIT:

I guess what is really confusing me is that every example I have seen has the load resistor on the outside of the circuit. In this case it is surrounded by resistors. Any suggestions on how to re-draw this circuit starting at the terminal points on either end of my load resistor would be greatly appreciated.

 

[gneill]

I guess what is really confusing me is that every example I have seen has the load resistor on the outside of the circuit. In this case it is surrounded by resistors. Any suggestions on how to re-draw this circuit starting at the terminal points on either end of my load resistor would be greatly appreciated.

In this case redrawing the circuit probably won't make it any simpler to understand or analyze --- it's already in a pretty simple and convenient form! If you consider what's left after R5 is removed you've got a pair of simple voltage dividers.

So finding the open-circuit potential between terminals a and b should be easy, right? Very slightly trickier is determining the resistance between a and b when the voltage source V is suppressed. See if you can find opportunities for series/parallel reductions.

 

[oddjobmj]

Ah yes, I recognized that form, thank you. What happens with the voltage with two parallel splitters I'm not sure. Would I be able to treat each splitter like a parallel resistor?

e.g. $\frac{1}{\frac{1}{R_a}+\frac{1}{R_b}}$

Where R_a=the resistance ratio of the first and similar for R_b


Doesn't seem right, really. Not sure how else to deal with that though.

 

[gneill]

Treat the two voltage dividers independently. Take the bottom rail as a reference point and determine the potential at a, then do the same for b. Knowing Va and Vb, what's Vab?

 

[oddjobmj]

Treat the two voltage dividers independently. Take the bottom rail as a reference point and determine the potential at a, then do the same for b. Knowing Va and Vb, what's Vab?

Using the bottom rail as a reference point the potential at a would be:
10V*(99/100)=4.975V

and at b:
10V*(1000/2000)=5V

The difference between b and a is 0.025V. Is that what you mean? If so, that makes sense. That difference would be the V_oc as measured at terminals a & b of the Thevenin equivalent. That also agrees with my circuit simulation in Multism.

To find R_TH I could divide that voltage by the current but how do I find that? Should I set up a mesh/loop diagram or is there a better way?

 

[gneill]

Using the bottom rail as a reference point the potential at a would be:
10V*(99/100)=4.975V $~~~~~~~~~~$typo?

and at b:
10V*(1000/2000)=5V

The difference between b and a is 0.025V. Is that what you mean? If so, that makes sense. That difference would be the V_oc as measured at terminals a & b of the Thevenin equivalent. That also agrees with my circuit simulation in Multism.

To find R_TH I could divide that voltage by the current but how do I find that? Should I set up a mesh/loop diagram or is there a better way?

While you could find the short circuit current and divide the open circuit voltage by it to find the resistance, the circuit is amenable to reduction in the usual parallel/serial reduction methods once the source is suppressed.

 

[oddjobmj]

Yeah, typo. Should be a denominator of 199, sorry.

Also, not sure why I was trying to find R_TH with that resistor in there. You're right... it is a lot easier without it. So, I have the Thevenin equivalent circuit which is simply a 181Ω resistor in series with a .025V source.

As noted the rest seems to fall into place when I attach my R₅ load resistor to the terminals.

For part (b) I basically have 181+10 as the circuit resistance. I can divide the .025V source by that total resistance to find the current through the load resistor, right? That just seems rather small. Also, if I did it that way the current through that resistor would change when I change the R5 resistance as required in (c). My simulation suggests the current would be the same for both (b) and (c). What am I missing?

Thank you again for all your help!

 

[gneill]

Yeah, typo. Should be a denominator of 199, sorry.

Also, not sure why I was trying to find R_TH with that resistor in there. You're right... it is a lot easier without it. So, I have the Thevenin equivalent circuit which is simply a 181Ω resistor in series with a .025V source.

181Ω does not look right for the Thevenin resistance. Can you show your work/reasoning?

 

[oddjobmj]

Ah, well, without R5 I looked at the circuit as if R1 and R3 were in series, R2 and R4 are in series, and then those two equivalent resistors are parallel:

$\frac{1}{\frac{1}{199}+\frac{1}{2000}}$

Perhaps that's not the equivalent resistance from the perspective of our R5 terminals though.

 

[gneill]

Ah, well, without R5 I looked at the circuit as if R1 and R3 were in series, R2 and R4 are in series, and then those two equivalent resistors are parallel:

$\frac{1}{\frac{1}{199}+\frac{1}{2000}}$

Perhaps that's not the equivalent resistance from the perspective of our R5 terminals though.

No, it's not. Those resistors are not in series. When V is suppressed (shorted) it makes the bottom rail and top rail all one node. That means, for example, the bottom terminal of R3 is connected to the top terminal of R1... so they're not in series...

 

[oddjobmj]

Ah wow, yes, thank you. I definitely forgot to consider shorting the source.

So R1 and R3 are clearly in parallel. R2 and R4 also share that common rail so they are also in parallel. Then those two equivalent resistors are also in parallel which leads to a total resistance of 45.25. That's still pretty high though.

Using that as a resistance my current is off by a factor of 10 compared to the simulation when I divide by (45.25+10). I=.0004525 A or 452μA.

If I instead assume that the two equivalent resistors are in series I get R_TH of 550Ω which I can use to find a current of 44.66 μA. That's still not what my simulation says (45.297 μA). Even though this is close I still think they are in parallel...

 

[gneill]

Ah wow, yes, thank you. I definitely forgot to consider shorting the source.

So R1 and R3 are clearly in parallel. R2 and R4 also share that common rail so they are also in parallel. Then those two equivalent resistors are also in parallel which leads to a total resistance of 45.25. That's still pretty high though.

Using that as a resistance my current is off by a factor of 10 compared to the simulation when I divide by (45.25+10). I=.0004525 A or 452μA.

If I instead assume that the two equivalent resistors are in series I get R_TH of 550Ω which I can use to find a current of 44.66 μA. That's still not what my simulation says (45.297 μA). Even though this is close I still think they are in parallel...

R1 and R3 are in parallel as you say. So are R2 and R4. From the perspective of the open terminals, those two equivalent resistances are in series.

You should be able to "see" the relationships in your mind's eye by allowing the connection points of components to "move" along the contiguous paths that comprise the node they are connected to. When in doubt, sketch intermediate transformations of the layout. For example, in this case if you understand the parallel connections of R1||R3 and R2||R4 you might sketch:

and see that the resulting parallel combinations are in series between the terminals.

 

[oddjobmj]

I'll definitely have to mess around with the layout some more so I am more comfortable with odd configurations like this.

Also, what am I missing with my simulation that suggests the current over R5 is the same whether R5 is 10 Ohm or 10k Ohm? Shouldn't I be dividing my voltage by the R_TH+R5? Did I make a mistake on my simulation or should I not be considering the R5 resistance when figuring current through it?

 

[gneill]

I'll definitely have to mess around with the layout some more so I am more comfortable with odd configurations like this.

Also, what am I missing with my simulation that suggests the current over R5 is the same whether R5 is 10 Ohm or 10k Ohm? Shouldn't I be dividing my voltage by the R_TH+R5? Did I make a mistake on my simulation or should I not be considering the R5 resistance when figuring current through it?

Sounds like a problem with the implementation of your simulation. The Thevenin equivalent model is pretty straightforward, being a voltage supply in series with resistance.