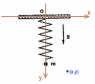
- #1
montrex
- 2
- 0
Homework Statement
A pointlike body of mass ##m=100## ##g## is attached to the extremity of an ideal spring, whose constant is ##K## and its length at relaxed position is negligible. The other extremity of the spring is attached to the fixed point ##O##.
At first the body mass, on which the gravitational force and elastic force act, is at rest on the vertical plane.
An horizontal impulse ##\vec J= 1## ##Kg \frac{m}{s}## acts on the body in a negligible time.
##(a)## Evaluate the maximum distance of the body from ##O##, specifying the trajectory of his motion.
##(b)## If the impulse acts at ##30°## grades respect to the horizontal direction, as in the picture, evaluate the minimum depth reached by ##m## respect to the altitude of ##O##.
Homework Equations
Hooke's law ##\vec F = - K \vec x##
Conservation of energy ##\Delta K +\Delta U_{g} +\Delta U_{e} =0##; where ##K## is the kinetic energy of the body, ##U_{g}## is its gravitational energy and ##U_{e}## is the elastic energy of the spring
Impulse ##\vec J## produced from time ##t_{1}## to ##t_{2}##: ##\vec J=\int \vec F \, dt = \Delta \vec p##
The Attempt at a Solution
This is what I thought. At the beginning, the body is at rest and the only forces are the gravitational and the elastic ones, which operate on a vertical axis. Thus, ##mg-kL_{0}=0##, where ##L_{0}## is the elongation of the spring at first. It is important to remember that its length at relaxed position is negligible, as hypothesis.
##(a)-(b)## Due to the impulse, the body has initial velocity ## V_{0}= \frac {I}{m}##, with the same direction of ##\vec J##. When it reaches the longest distance from ##O##, its velocity ##V_{f}=0##. Since there is a spring and not a simple rope, I am not able to relate the height reached by the body to the streching of the spring.
Thanks for considering my question, any suggestion for the problem or better translation is welcolmed.
Last edited: