Intuition on the direction of friction in a rotational dynamics problem

[FranzDiCoccio]

Homework Statement

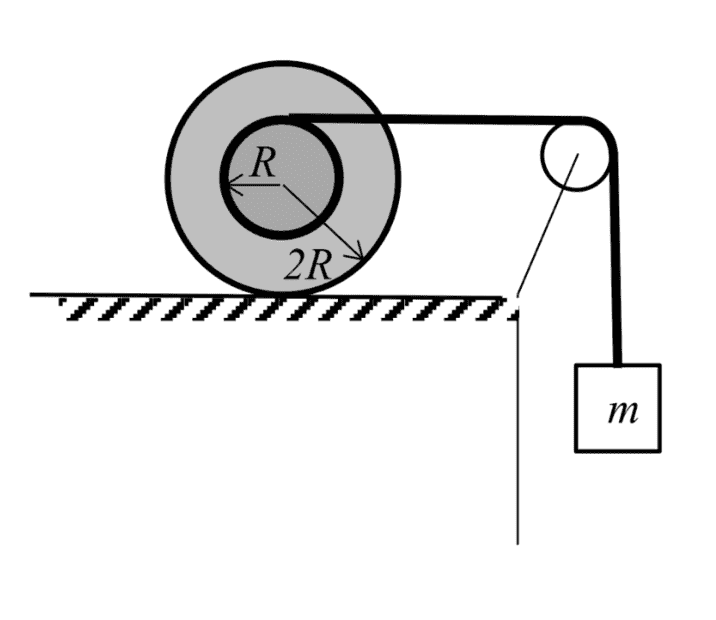
an object is suspended by a rope that goes around a pulley and connects to a disc that is free to roll without sliding on a horizontal plane. The rope is coiled around a smaller disc welded onto the larger disc. Find the acceleration of the suspended object and the static friction between the plane and the disc.

Relevant Equations

second law of dynamics OR energy conservation plus uniformly accelerated motion

The figure illustrates the situation. The radii of the larger and smaller discs are $2R$ and $R$, respectively. Their masses are $M$ and $2M$, respectively (the largst disc has the smallest mass).
Also, $m=5/4 M$, where $m$ is the mass of the suspended object. The pulley is "massless" (negligible moment of inertia).

One way of doing this is by writing three equations from the second law of dynamics: one for the acceleration of the object, one for the translational acceleration of the wheel, one for the rotational acceleration of the wheel. This is a system of three equations with three unknowns: the tension $T$ of the rope, the magnitude (and direction!) of the friction and the acceleration of the suspended object. These unknowns can be calculated.

A different approach consists in using energy conservation to find a relation between the translation of the object and its velocity. We expect this to be a uniformly accelerated motion, so we can calculate the acceleration.

In both cases I get
$$a = \frac{3}{7} g, \qquad F = \frac{1}{3} M g \qquad T = \frac{5}{7} Mg$$

I find there are two "tricky" points in this problem, which I hope I got right.
First, one has to realize that the acceleration of the object is $a=3 R \alpha$.
This is because the wheel moves while the coil unwinds. So the displacement of the object is given by the displacement of the wheel plus the extra length of rope due to its unwinding.

The second tricky point is the direction of the friction. At first I naively thought it was opposite to the tension of the rope (i.e. leftwards).
However the equations suggest that friction goes from left to right. This makes sense, because the tension alone would generate an angular acceleration that is greater than $\frac{a}{3R}$. The only "solution" is that friction cannot "add" to the torque of the tension, but has to hinder it.
It's kind of like when a car accelerates so much that its wheels slide on the street (bear with my English, I cannot find a better way of saying this).

And here comes my question: is there an intuitive argument for getting the direction of the friction right before solving the equations?
As I mention, when I first tried solving the problem I had to draw all the forces in order to write the second law for each object.
Since I originally chose the wrong direction for the friction, I obtained a "negative magnitude" for the friction.
This is not a big deal. It simply means I am working with the cartesian components of the forces, rather than with their magnitudes.
Still, I'm curious about an intuitive argument for the direction of the friction, which would allow one to work with magnitudes alone.

Thanks
Francesco

 

[haruspex]

No, there is no simple argument. If you vary the size of the smaller disc you will likely find a critical radius at which there is no frictional force. There is no way to guess where that is. Not sure if it will depend on the masses, but probably.

 

[Delta2]

In problems like this, the friction either opposes translational motion and augments rotational motion (e.g rolling cylinder in an incline), or augment translational motion and oppose rotational motion (like it happens in the wheels of the car or in this problem).

 

[FranzDiCoccio]

No, there is no simple argument. If you vary the size of the smaller disc you will likely find a critical radius at which there is no frictional force. There is no way to guess where that is. Not sure if it will depend on the masses, but probably.

I was afraid of that. So one has to start by guessing the direction of the friction. The equations will contain the cartesian component of the friction rather than its (positive) magnitude.
If this component turns out to be positive, the initial guess on the direction was correct. Otherwise, the friction goes the other way.

In problems like this, the friction either opposes translational motion and augments rotational motion (e.g rolling cylinder in an incline), or augment translational motion and oppose rotational motion (like it happens in the wheels of the car or in this problem).

Sure, my question was about the possibility of guessing the right direction before solving the equations.

I find similar issues when solving equilibrium problems with torques.
There, I have to impose equilibrium both for torques and for forces. But the direction of some of these forces is sometimes unknown. In that case I work with the torque, which has to vanish wrt any axis. Upon chosing the correct axis one can correctly guess the direction of the unknown force, even if its magnitude remains unknown.
This problem is much trickier, basically because there's too much stuff moving, and the motion is accelerated.

Just to be on the safe side, I'm including the equations I used for the solution. As I say $a = 3 R \alpha$. Also, the moment of inertia of the wheel is $I=3M R^2$, its total mass is $3M$ and its linear acceleration is $2 R \alpha = \frac{2}{3} a$.
After a little algebra I get

$$P - T = \frac{5}{4} M a\qquad T - 2F = M a \qquad T+F = 2 M a$$

where I used my knowledge that $F$ has the same direction as $T$.

As I say, the solutions I get are
$$g = \frac{3}{7} g \qquad F = \frac{1}{7} Mg \qquad T = \frac{5}{7} Mg$$

According to the equations, the magnitude of $T$ does not depend on the friction. What I mean here is that I'd get exactly the same result even with the wrong choice for the direction of the friction (which would result in a change of the sign in front of $F$ in the above equations).

Now, to make sense of the direction of the friction I reasoned like this: if only the tension was present, the resulting angular acceleration would be greater than $\frac{a}{3R}$, which is my starting point.
For the result to be consistent, the torque coming from the friction has to fight the torque coming from the tension, which means that these two vector have the same direction.

 

[haruspex]

So one has to start by guessing the direction of the friction.

No, you just choose a positive direction. That's not the same as guessing which way it will go.

 

[Delta2]

No, you just choose a positive direction. That's not the same as guessing which way it will go.

Don't understand you, choose and guess are synonyms for me in this context...

 

[FranzDiCoccio]

No, you just choose a positive direction. That's not the same as guessing which way it will go.

Yes, I think I understand what you mean.

I was just referring to the fact that the vector I initially draw before writing and solving the equations might have the wrong direction.
After solving the equations I might have to draw a new figure where the direction of the unknown vector is the correct one.

 

[haruspex]

Don't understand you, choose and guess are synonyms for me in this context...

But you are not choosing the positive direction based on which way you think it will go. The choice is arbitrary. Don't even waste time trying to predict it. If the value comes out negative, so be it. There's no failure implied.

 

[FranzDiCoccio]

I think the point is kind of subtle, and has to do with the definition of cartesian components.
I think that, rigorously, components are scalars.
Sometimes these are represented as vectors along the relevant axis. If the sign of the cartesian component is known, there is no real problem with that. Everything makes immediate sense.
If, however, the sign of the component is unknown, the vector you draw might be wrong.

Having to make a choice, I agree that the less confusing one is that the vector has the same direction as the axis. This way, the number you get after solving the equations is actually the cartesian component.

In a less rigorous way, one can choose one direction, and work with magnitudes as if this was the correct one. The unknown magnitude might turn out to be negative, which does not make sense, rigorously.
This still tells you that the direction you chose is the wrong one, but the number you get could not be the cartesian component.
For instance, one decides that the vector points against the axis, and turns out to be right. The solution gives the magnitude of the vector, which however is the opposite of its cartesian coordinate, which should be negative.
So, rigorously this is less satisfactory, because the signs are all over the place.

 

[haruspex]

After solving the equations I might have to draw a new figure where the direction of the unknown vector is the correct one.

The direction in which you draw the vector should match which way you take as positive in your equations. It does not have to be the direction that it turns out to act in.
When all the vectors are horizontal or vertical, it is common to take right and up as positive for everything. Vectors drawn in the FBD should all point in those two directions, though that is often violated.

 

[haruspex]

In a less rigorous way, one can choose one direction, and work with magnitudes as if this was the correct one.

With Cartesian coordinates, the vector elements are scalars, so that's how you should work.
With polar coordinates, r is technically a magnitude, so take the angle to be anywhere from 0 to 2pi.

 

[FranzDiCoccio]

The direction in which you draw the vector should match which way you take as positive in your equations. It does not have to be the direction that it turns out to act in.

What you are describing is an algorithm for determining the unknown vector. As you say, when the vectors are unknown, sometimes there is no way of drawing them in their actual direction for sure. I agree that in these cases the cleanest way to go is to draw them in the same direction as the axis. But the resulting drawing might be wrong, in the sense it does not depict all the vectors correctly.

I am just saying that, in my opinion, a complete discussion of the problem should include a drawing where the (previously) unknown vector points in the correct way (of course only if it points in the wrong way in the original drawing).

When all the vectors are horizontal or vertical, it is common to take right and up as positive for everything. Vectors drawn in the FBD should all point in those two directions, though that is often violated.

Well, sometimes a different choice might be more convenient.

But these are subtleties. I was more interested in an opinion about my solution of the actual problem. Specifically, the facts that $a_{\rm object} = 3 R \alpha$ and $a_{\rm wheel}=2R \alpha = 2/3 R a$.

 

[Lnewqban]

...
And here comes my question: is there an intuitive argument for getting the direction of the friction right before solving the equations?

I believe so, in most cases.
You have to keep in mind that all forces manifest themselves in an action-reaction pair.

If you are doing the free body diagram of the disc, you will see a reaction force at the point of contact with the horizontal plane.
If you do the same diagram for the plane, you will see a force of same magnitude pointing in the exact opposite direction.
Which one is considered friction?

If you can imagine both extreme situations accurately, your intuition will seldom fail: no friction, and maximum friction.

No friction between disc and horizontal plane:
The disc will slide in an accelerated way, no matter how massive it may be.
It eventually will star an accelerated rotation, if the driving force is not aligned with the center of mass.
Just like if you were skatting on ice and somebody pulled you with a rope.

Maximum friction between the disc and the horizontal plane:
This situation can be visualized as being similar to a rack and pinion mechanism.
The teeth of the rack will be a solid obstacle, over which the wheel can only rotate (driven by the force) by leaving its teeths behind, at the spatial point where each was meshed up with its corresponding tooth of the rack.

At each point of contact, the tooth of the rack is pushing in the direction opposite to the linear movement of the wheel; in a reactive way, the tooth of the wheel will push back in the opposite direction of that force, which coincides with the direction of linear movement of the center of mass of the wheel.

Please, see:
https://upload.wikimedia.org/wikipedia/commons/2/28/Animated_rack_and_pinion.gif

 

[haruspex]

I was more interested in an opinion about my solution of the actual problem. Specifically, the facts that $a_{\rm object} = 3 R \alpha$ and $a_{\rm wheel}=2R \alpha = 2/3 R a$.

Yes.