Principle of Virtual Work and Feynman

[lugita15]

I was just reading the Feynman Lectures on Physics when Feynman discussed virtual work. Unfortunately, I found his explanation somewhat confusing.
The following are the three examples Feynman uses in order to illustrate the principle of virtual work.
1.

2.

3.

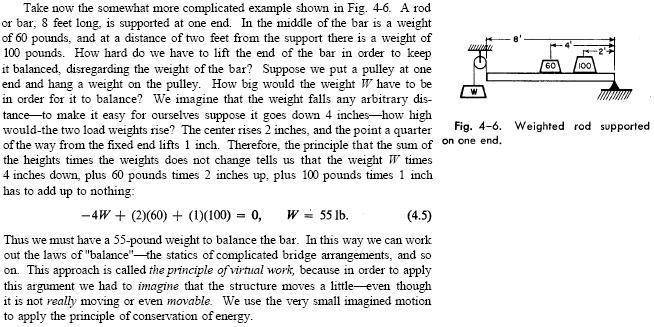
I'm trying to understand the principle of virtual work. Is there anyone who could elucidate Feynman's explanation.

Thank You in Advance.

 

[HallsofIvy]

I find it difficult to understand just about everything Feynman says!

But there's nothing very complicated here: He's say suppose a body moved under a force- calculating the work done and then dividing by the distance tells us the force. Of course, this only works when you can calculate the work done without using the unknown force- essentially conservative forces where you can use potential energy. If you added friction to any of those examples, the method would not work any more.

 

[Cyrus]

Without going into depth,

Virtual work has the following properties:

- Infinitesimal displacements
- Consistent with system constraints
- The variation of displacements occur instantaneously, so time is not involved.

Virtual work is useful because constraint forces disappear when you perform your analysis and so you don't have to solve for unknown internal reactions.

 

[lugita15]

Using your explanation, I kind of understand the principle of virtual work, and fully understand the first example.
I almost understand the second example, except I don't see where Feynman got the 126 inches from. How do you know the radius of the screw?
However, I still don't see how the third example works. How do you know that when the weight falls 4 inches that the center will rise 2 inches? Also, how do you know that the point 2 inches from the fixed end will rise 1 inch?

Any further help would be greatly appreciated.

 

[lugita15]

Of course, this only works when you can calculate the work done without using the unknown force- essentially conservative forces where you can use potential energy. If you added friction to any of those examples, the method would not work any more.

I disagree. The energy dissipated by friction becomes thermal or internal energy. So friction is also, ultimately, a conservative force.

 

[HallsofIvy]

I disagree. The energy dissipated by friction becomes thermal or internal energy. So friction is also, ultimately, a conservative force.

I wasn't talking about "conservation of energy". Look up "conservative force".

 

[HallsofIvy]

Using your explanation, I kind of understand the principle of virtual work, and fully understand the first example.
I almost understand the second example, except I don't see where Feynman got the 126 inches from. How do you know the radius of the screw?

You are told that the handle is 20 inches long so the end of the handle goes around in a circle with diameter 40 inches. 40\pi is approximately 126 inches.

However, I still don't see how the third example works. How do you know that when the weight falls 4 inches that the center will rise 2 inches? Also, how do you know that the point 2 inches from the fixed end will rise 1 inch?

Use proportions. If the weight falls 4 inches, since the cord attaching it to the end of the bar does not stretch, the end of the bar rises 4 inches. You should be able to see that, looking at the initial position of the bar and its (virtual) position after "moving" that you have 3 similar triangles. One with vertex at the end of the bar, another with vertex at the center weight and one with vertex at the lower weight. Since the triangles are similar, the vertical distances raised are in the same proportions as the distances from the pivot to the weights and end of bar. The center weight has distance from the pivot 1/2 the length of the bar and so rises half the distance the end of the bar does. The lower weight is 1/4 the length of the bar from the pivot and so rises 1/4 the distance the end of the bar does.

 

[lugita15]

I wasn't talking about "conservation of energy". Look up "conservative force".

I know you what a conservative force is. When a ball is rolled and starts to slow down due to friction, the energy is still kinetic energy. Heat is simply the kinetic energy of individual molecules. If you roll a ball around a closed path on a rough surface, zero work is done on it.