Pulley-to-Shaft-to-Pulley Calculation?

[playludesc]

Hello all! I'm working with a particular pulley configuration and I realized after a few calculations that I don't have an accurate way to calculate one part of the set up.

Here's a quick MSPaint sketch showing what I need to solve for. If I know the outer diameter of both pulleys, and the RPM of the black pulley, how can I solve for the RPM at the outer diameter of the blue pulley? (If you care, this is regarding the jackshaft for my supercharger!)

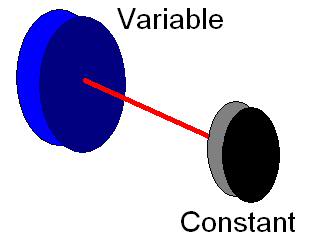
Thanks very much for your help!

 

[playludesc]

So I figured that this is probably a simple mechanical advantage equation, as the two pulleys simply work like levers on each other. Here's what I ended up with:

Blue pulley diameter = 1.835"
Black pulley diameter = 2.000"
Black pulley RPM = 22,023.5

1.835/2.000 = 0.9175

22,023.5*0.9175 = RPM for Blue pulley of 20,206.5

Can anyone confirm if I've done that correctly; or, more importantly, if mechanical advantage is the right way to calculate this?

 

[sophiecentaur]

If they're on the same shaft (as in the picture) then they would both rotate at the same rate so the speeds of the two belts (?) will be proportional to the diameters. If they are coupled by a belt then the peripheral speeds will be the same.
Take your pick.

 

[playludesc]

If they're on the same shaft (as in the picture) then they would both rotate at the same rate so the speeds of the two belts (?) will be proportional to the diameters. If they are coupled by a belt then the peripheral speeds will be the same.
Take your pick.

Right, would the belts' speed be directly proportional, like in my calculation above? Or should I be using a different formula?

 

[sophiecentaur]

Right, would the belts' speed be directly proportional, like in my calculation above? Or should I be using a different formula?

Which bit are you asking is right? In one bit you seem to imply that the RPMs would be different. How could that be if they are on the same shaft?
The belt speed 'out' will be belt speed 'in' times the ratio of diameters - it's that simple.

 

[DennisN]

Hi playludesc! I'm not sure I understand your sketch (what does variable and constant mean in the sketch?). If two wheels are connected via a normal axle, they will have the same RPM (revolutions/minute), regardless of the diameter of the wheels (unless you mean some entirely different setup). You may be confusing rotational speed (revolutions/minute) with tangential speed (e.g. centimeters/second).

The circumferences c of the wheels are (d are diameters):

c_black = pi*d_black

c_blue = pi*d_blue

so the tangential velocities v at the circumference of the wheels will be

v_black = c_black/t = pi*d_black * RPM

v_blue = c_blue/t = pi*d_blue * RPM

If you measure the diameters in cm, the velocities above will be cm/minute (and t is time in minutes).
If you measure the diameters in inches, the velocities above will be inches/minute.
Edit: I saw sophiecentaur had already answered about the RPM, while I was writing my reply...

 

[playludesc]

Which bit are you asking is right? In one bit you seem to imply that the RPMs would be different. How could that be if they are on the same shaft?
The belt speed 'out' will be belt speed 'in' times the ratio of diameters - it's that simple.

That's what I thought. Sorry if my phrasing was confusing.

I'm just asking if my method of calculating the "out" speed for the blue pulley is correct in my second post. Your posts seem to agree with my method, so we're good!

 

[playludesc]

Hi playludesc! I'm not sure I understand your sketch (what does variable and constant mean in the sketch?). If two wheels are connected via a normal axle, they will have the same RPM (revolutions/minute), regardless of the diameter of the wheels (unless you mean some entirely different setup). You may be confusing rotational speed (revolutions/minute) with tangential speed (e.g. centimeters/second).

The circumferences c of the wheels are (d are diameters):

c_black = pi*d_black

c_blue = pi*d_blue

so the tangential velocities v at the circumference of the wheels will be

v_black = c_black/t = pi*d_black * RPM

v_blue = c_blue/t = pi*d_blue * RPM

If you measure the diameters in cm, the velocities above will be cm/minute (and t is time in minutes).
If you measure the diameters in inches, the velocities above will be inches/minute.
Edit: I saw sophiecentaur had already answered about the RPM, while I was writing my reply...

Thanks very much for taking the time to answer so thoroughly. I think sophie confirmed that I'm on track for my application. Even so, I'll wrap my English degree head around your post and get back to you in a bit!

 

[jim hardy]

A jackshaft, also called a countershaft, is a common mechanical design component used to transfer or synchronize rotational force in a machine. A jackshaft is often just a short stub with supporting bearings on the ends and two pulleys, gears, or cranks attached to it.

http://en.wikipedia.org/wiki/Jackshaft

Aha that solves the mystery. Sophie and dennis both picked up on it.

Is your blue pulley a variable diameter sheave?
What are you supercharging, just out of curiosity?

 

[playludesc]

Nah, blue pulley will be a timing belt pulley, I'm just trying to figure out exactly what size to make it, which in the calculation/selection phase makes it the variable.

I've got a ported M62 going on the H22a4 in my fifth generation Prelude.

 

[DennisN]

Hi again! I might as well also simplify my two velocity equations further. If the pullies have the same RPM (as I suppose), then the velocity relations can be divided to yield the following relation;

v_blue/v_black = d_blue/d_black

which means e.g.

d_blue = (d_black*v_blue)/v_black

(d=diameters, v=tangential speeds)
I don't know if it helps you, I'm not sure about the other stuff in your project . (Btw, the t I used before was the period, i.e. the time for one revolution.)