- #1
Huumah
- 28
- 0
Can anyone explain why we obtain the part where i put the red underline.
I understand everything until then
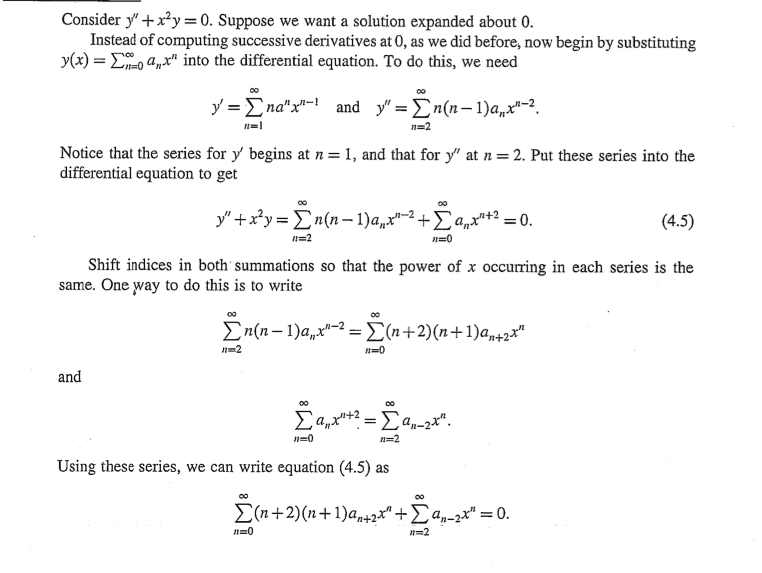

I understand everything until then
A recurring relation is a mathematical relationship between two or more variables, expressed in the form of a recurrence equation. This equation shows how the value of one variable is related to its previous value, and can be used to solve for unknown values.
A differential equation is an equation that relates the rate of change of a variable to its current value and other variables. It is commonly used to model natural phenomena and physical systems, and can be solved to find the behavior or evolution of a system over time.
Recurring relations can be used as a tool to simplify and solve differential equations. By converting a differential equation into a recurrence equation, it becomes easier to manipulate and solve for unknown values. This can be especially useful for complex systems with multiple variables.
Some common methods for solving recurring relations in differential equations include the method of undetermined coefficients, the method of variation of parameters, and the method of power series. These methods involve using different techniques to manipulate the recurrence equation and solve for the desired variable.
Solving recurring relations in differential equations has a wide range of applications, from understanding the behavior of physical systems to predicting future trends in economics and population growth. It also has practical uses in fields such as engineering, physics, and biology, where differential equations are commonly used to model and analyze complex systems.