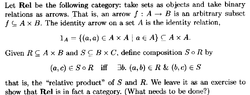Rel as a Category .... Awodey Section 1.4, Example 4, Pae 7 ....

[Math Amateur]

I am reading Steve Awodey's book: Category Theory (Second Edition) and am focused on Section 1.4 Examples of Categories ...

I need some further help in order to fully understand some aspects of the definition of the category Rel ... ...

The definition of the category Rel ... reads as follows:View attachment 8387
I am having trouble fully understanding the definition of the identity arrow ...I will use an example to illustrate my problems ...Let the category \(\displaystyle \text{Rel}_1\) consist of two sets \(\displaystyle A, B\) where

\(\displaystyle A = \{ 1, 2, 3 \}\)

\(\displaystyle B = \{ 3, 4 \} \)

so ... two arrows, for example, may be \(\displaystyle f_1 : A \to B\) where \(\displaystyle f_1 = \{ (1, 3), (1, 4), (3, 4) \}\)

and \(\displaystyle f_2 : A \to B\) where \(\displaystyle f_2 = \{ (1, 3) \}\)
Now .. consider \(\displaystyle f_3 : A \to A\) where \(\displaystyle f_3 = \{ (1, 1) \}\)

and \(\displaystyle f_4 : A \to A\) where \(\displaystyle f_4 = \{ (2, 2) \}\)

and \(\displaystyle f_5 : A \to A\) where \(\displaystyle f_5 = \{ (3, 3) \}\) ... BUT ...... according to Awodey's definition these arrows are all equal to the identity arrow of A ...... the identity arrow is meant to be unique ... ?Does this mean \(\displaystyle f_3 = f_4 = f_5\) ... ? ... but why and how are they equal ...
Can someone please clarify the above ... ?

Peter

 

[steenis]

No, $1_A=\{(a,a) | a \in A \}$, that is all the pairs $(a,a)$ with $a \in A$

Thus $1_A=\{ (1,1), (2,2), (3,3) \}$

In your notation $1_A = f_3 \cup f_4 \cup f_5$

Now compute $f_1 \circ 1_A$.

 

[Math Amateur]

No, $1_A=\{(a,a) | a \in A \}$, that is all the pairs $(a,a)$ with $a \in A$

Thus $1_A=\{ (1,1), (2,2), (3,3) \}$

In your notation $1_A = f_3 \cup f_4 \cup f_5$

Now compute $f_1 \circ 1_A$.

Thanks Steenis ... that solves that one ...

Now working on the computation you mentioned..

Thanks again for the help ...

Peter

 

[Math Amateur]

No, $1_A=\{(a,a) | a \in A \}$, that is all the pairs $(a,a)$ with $a \in A$

Thus $1_A=\{ (1,1), (2,2), (3,3) \}$

In your notation $1_A = f_3 \cup f_4 \cup f_5$

Now compute $f_1 \circ 1_A$.

Hi Steenis ...You write:"... ... Now compute $f_1 \circ 1_A$ ... ... "

First ... define composition ...

If we are given two arrows \(\displaystyle f : A \to B\) and \(\displaystyle g : B \to C\) ...

... then ...

\(\displaystyle g \circ f = \{ \left \langle a, c \right \rangle \in A \times C \mid \exists \ b \ ( \left \langle a, b \right \rangle \in f \text{ and } \left \langle b, c \right \rangle \in g ) \} \)so, in particular, for \(\displaystyle f_1 : A \to B\) and \(\displaystyle 1_A : A \to A\) we have ...

\(\displaystyle f_1 \circ 1_A = \{ \left \langle a, b \right \rangle \in A \times B \mid \exists \ x \ ( \left \langle a, x \right \rangle \in 1_A \text{ and } \left \langle x, b \right \rangle \in f_1 ) \}\) ... ... ... ... ... (1)
Now ... showing informal working ... following (1) we have

\(\displaystyle \left \langle 1, 1 \right \rangle \in 1_A \text{ and } \left \langle 1, 3 \right \rangle \in f_1 \Longrightarrow \left \langle 1, 3 \right \rangle \in f_1 \circ 1_A \) where \(\displaystyle x = 1\)\(\displaystyle \left \langle 1, 1 \right \rangle \in 1_A \text{ and } \left \langle 1, 4 \right \rangle \in f_1 \Longrightarrow \left \langle 1, 4 \right \rangle \in f_1 \circ 1_A\) where \(\displaystyle x = 1\)\(\displaystyle \left \langle 3, 3 \right \rangle \in 1_A \text{ and } \left \langle 3, 4 \right \rangle \in f_1 \Longrightarrow \left \langle 3, 4 \right \rangle \in f_1 \circ 1_A\) where \(\displaystyle x = 3\)Thus \(\displaystyle f_1 \circ 1_A = \{ \left \langle 1, 3 \right \rangle , \left \langle 1, 4 \right \rangle , \left \langle 3, 4 \right \rangle \}\)
Is the above computation correct?

Peter

 

[steenis]

Yes, correct, and why did you already expect this answer ?

 

[Math Amateur]

Yes, correct, and why did you already expect this answer ?

Hmm ... not sure that i did expect it ...

Still reflecting on this ...

Peter***EDIT***

Oh! Obvious really ... because\(\displaystyle f_1 \circ 1_A = f_1\) ...

Peter

 

[steenis]

allright, $1_A$ is an identity arrow in Rel