Separable Polynomials - Remarks by Dummit and Foote .... ....

[Math Amateur]

Dummit and Foote in Section 13.5 on separable extensions make some remarks about separable polynomials that I do not quite follow. The remarks follow Corollary 34 and its proof ...

Corollary 34, its proof and the remarks read as follows:

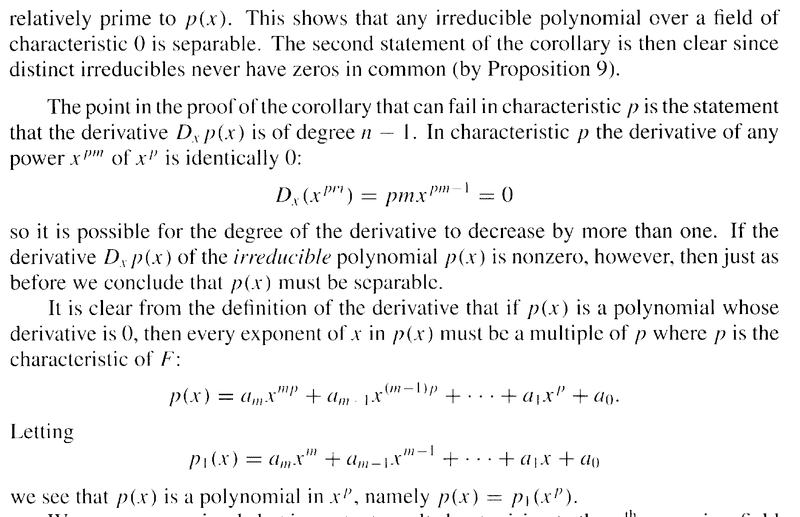
In the above text by D&F, in the remarks after the proof we read:

" ... in characteristic $p$ the derivative of any power $x^{pm}$ of $x^p$ is identically $0$:

$D_x( x^{pm} ) = pm x^{pm - 1 } = 0$

so it is possible for the degree of the derivative to decrease by more than $1$.

If the derivative $D_x p(x)$ of the irreducible polynomial $p(x)$ is non-zero, however, , then just as before we conclude that $p(x)$ must be separable. ... ... "My questions are as follows:Question 1

I am assuming that when the degree of the derivative decreases by more than $1, p(x)$ is still relatively prime to $D_x p(x)$ and so $p(x)$ is separable ... is that the correct reasoning here ...Question 2

In stating that "if the derivative $D_x p(x)$ of the irreducible polynomial $p(x)$ is non-zero, however, , then just as before we conclude that $p(x)$ must be separable". D&F are implying that if $D_x p(x) = 0$ then $p(x)$ is not separable (or not necessarily separable) ... is this the case ... if it is the case, why/how is this true ...
Hope that someone can help ...

Peter

 

[andrewkirk]

First another notation whinge: it's a really bad idea for the author to use $p$ both as the label for the polynomial and as the characteristic of the field. The two have nothing to do with one another, and the opportunities for confusion are rife. In protest I will use $q$ to denote the polynomial.

For Question 2: If $D_x q$ is nonzero then it must be co-prime with $q$ in $F[x]$. So all factors in $F[x]$ of $D_xq$ must also be co-prime with $q$. Hence, by proposition 9, none of those factors have any roots/zeros (over the splitting field $K$) in common with $q$, and hence $D_xq$ also has no roots in common with $q$. But any multiple root of $q$ must also be a root of $D_xq$ (with multiplicity one less than in $q$), so we can conclude that $q$ has no multiple roots. Hence $q$ is separable.

However if $D_xq$ is zero, that argument does not work, because $q$ divides 0, so $q$ and $D_xq$ are not co-prime. It looks like he's going to go on from there to show how to find a multiple root of an irreducible with zero derivative, over a field of nonzero characteristic.

For Question 1:

I am assuming that when the degree of the derivative decreases by more than 1, $p(x)$ is still relatively prime to $D_x p(x)$ and so $p(x)$ is separable ... is that the correct reasoning here ...

Yes, the argument works as long as the degree doesn't go all the way down to -1 (indicating the zero polynomial).