Tangent Spaces and Subspaces .... McInerney Theorem 3.3.13 ....

[Math Amateur]

TL;DR Summary

Thread involves finding the dimension of a tangent subspace ...

I am reading Andrew McInerney's book: First Steps in Differential Geometry: Riemannian, Contact, Symplectic ... and I am focused on Chapter 3: Advanced Calculus ... and in particular on Section 3.3: Geometric Sets and Subspaces of $T_p ( \mathbb{R}^n )$ ... ...

I need help with an aspect of the proof of Theorem 3.3.13 ... ...

Theorem 3.3.13 (together with a relevant definition) reads as follows:

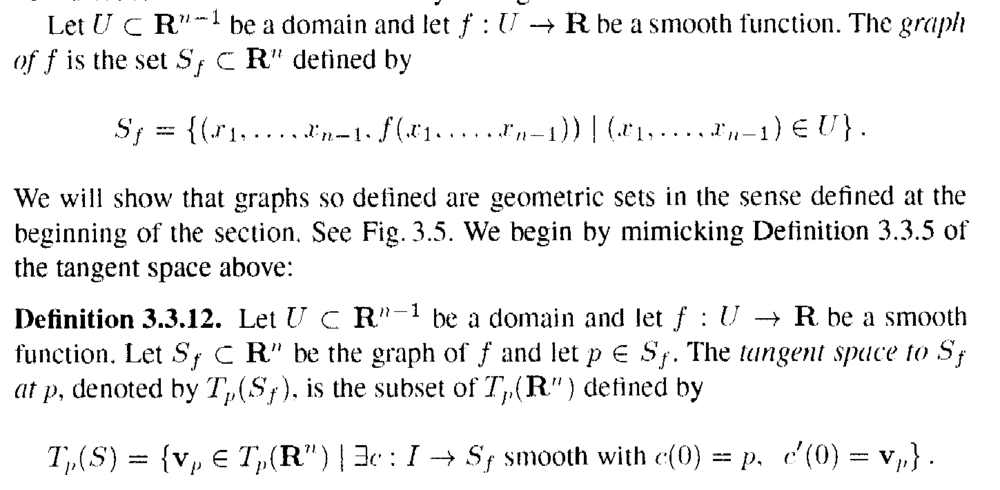
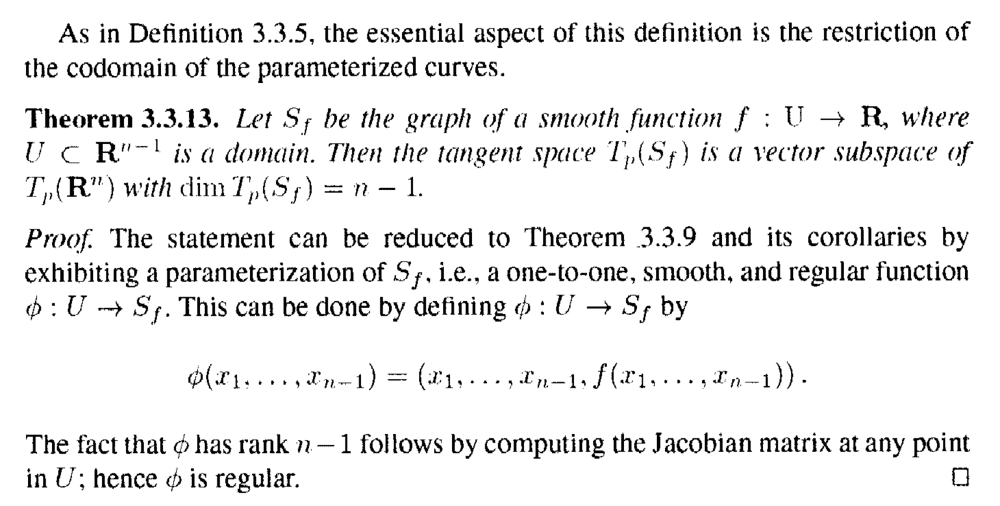
In the above text from McInerney we read the following:

" ... ... The fact that $\phi$ has rank $n -1$ follows by computing the Jacobian matrix at any point in $U$ ... ... "Can someone please demonstrate rigorously, formally and explicitly that $\phi$ has rank $n -1$ ... ...My computations with respect to the Jacobian $[D \phi(p) ]$ were as follows:

We have $\phi ( x_1, \ ... \ ... \ x_{n-1} ) = ( x_1, \ ... \ ... \ x_{n-1}, f( x_1, \ ... \ ... \ x_{n-1}) )$

Now put ...

$f_1( x_1, \ ... \ ... \ x_{n-1} ) = x_1$

$f_2( x_1, \ ... \ ... \ x_{n-1} ) = x_2$

... ... ...

... ... ...

$f_{n-1}( x_1, \ ... \ ... \ x_{n-1} ) = x_{n-1}$

$f_n( x_1, \ ... \ ... \ x_{n-1} ) = f( x_1, \ ... \ ... \ x_{n-1} )$Then ... the Jacobian ...$[D \phi(p) ] = \begin{bmatrix} \frac{ \partial f_1 }{ \partial x_1} & ... & ... & \frac{ \partial f_1 }{ \partial x_{n-1} } \\ ... & ... & ... & ... \\ ... & ... & ... & ... \\ \frac{ \partial f_{n-1} }{ \partial x_1} & ... & ... & \frac{ \partial f_{n-1} }{ \partial x_{n-1} } \\ \frac{ \partial f }{ \partial x_1} & ... & ... & \frac{ \partial f }{ \partial x_{n-1} } \end{bmatrix}$$= \begin{bmatrix} 1 & 0 & 0 & ... & ... & 0 \\ 0 & 1 & 0 & ... & ... & 0 \\ ... & ... & ... & ... & ... & ... \\ ... & ... & ... & ... & ... & ... \\ \frac{ \partial f }{ \partial x_1} & ... & ... & ... & ... & \frac{ \partial f }{ \partial x_{n-1} } \end{bmatrix}$

... now ... how do we show that the rank of $[D \phi(p) ]$ is $n-1$ ...?

Hope someone can help ...

Peter

 

[fresh_42]

Hi Peter,

if you write down a bit more of the Jacobi matrix, then it will be
$[D \phi(p) ] = \begin{bmatrix} 1 & 0 & 0 & ... & ... & 0 \\ 0 & 1 & 0 & ... & ... & 0 \\ ... & ... & ... & ... & ... & ... \\ ... & ... & ... & ... & 1 & 0 \\ ... & ... & ... & ... & 0 & 1 \\ \frac{ \partial f }{ \partial x_1} & ... & ... & ... & ... & \frac{ \partial f }{ \partial x_{n-1} } \end{bmatrix}$

We have a matrix of dimensions $n$ times $n-1$, so the rank can at most be $n-1$ because we cannot have $n$ independent columns, if there are only $n-1$. The rank is also at least $n-1$ since the first $n-1$ rows are linear independent.

 

[Math Amateur]

Thanks fresh_42 ...

Appreciate your help ...

Peter

 

[mathwonk]

note also the matrix is that of a linear map from R^n-1 to R^n, hence the rank cannot be more than n-1.

 

[Math Amateur]

Thanks mathwonk ...

Peter