MHB Triangle: calculate angle between raised height and angle follower

[HotPrompt]

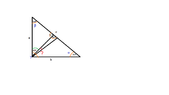
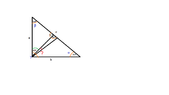
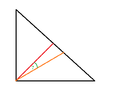
One sharp corner of a right-angled triangle is 50º. Calculate the angle between the raised height and the angle follower at right angles.
So I know that the angles are 90º, 50º and 40º. How do I find the angle between the raised height and angle follower?
View attachment 8178

 

[HOI]

I don't know what you mean by "angle follower". Trying to google it I get a lot of hits on 'cams' that don't seem to have anything to do with this problem.

 

[HotPrompt]

One of the lines is angle bisector and the other is a line drawn from the 90º corner to the hypotenuse.

 

[I like Serena]

One of the lines is angle bisector and the other is a line drawn from the 90º corner to the hypotenuse.

The sum of the angles in a triangle is 180º.
So γ = 180º - α - β = 180º - 50º - 40º = 90º.
Its angle bisector (if that is what it is, although I kind of doubt it when looking at the drawing) would therefore be 45º. (Thinking)

 

[HotPrompt]

I have to draw a line from the 90º corner to the hypotenuse c, which fill split the triangle into bisectors. After that I have to draw a new line from the same 90º corner to the hypotenuse height line, and I have to calculate the angle between these two lines. If this makes it clearer..
View attachment 8186
I have to find the angle where green "?" is, knowing only that one corner is 50º. But since it's a right-angled triangle then we know that one of the corners is 90º.

 

[I like Serena]

I have to draw a line from the 90º corner to the hypotenuse c, which fill split the triangle into bisectors. After that I have to draw a new line from the same 90º corner to the hypotenuse height line, and I have to calculate the angle between these two lines. If this makes it clearer..

I have to find the angle where green "?" is, knowing only that one corner is 50º. But since it's a right-angled triangle then we know that one of the corners is 90º.

The angle $\gamma$ of 90º is split into 3 smaller angles.
We already know that the top one is 40º.
So if the new line is an angle bisector (which is not clear from the drawing), it bisects $\gamma$ into 2 angles of 45º each.
It means that the unknown angle in between is 5º.

 

[HotPrompt]

Thank you, I think I got it now!