Understanding Operators in Matrix Mechanics

[Avardia]

I'm trying to understand some notes that I have been given on Matrix Mechanics, specifically how the matrix element comes about and builds a matrix which when used applies the effect of an operator on a wavefunction. But I'm having some difficulties following what's being done in the notes with confidence. I've seen others do this another way which I can follow well enough but I really want to see how this one works.

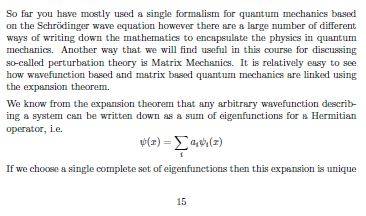
As far as I understand the notes start off recapping expansion theorem and say if we work with a single complete set of eigenfunctions to build any old wavefunction then what we get is a unique list of coefficients for each wavefunction we are expanding. We then see an operator acting on a generic wavefunction and since we work with linear operators its equivalent to applying the operator to each of the eigenfunctions that we use to expand the generic wavefunction. Seen in the equation above equation (2.1).

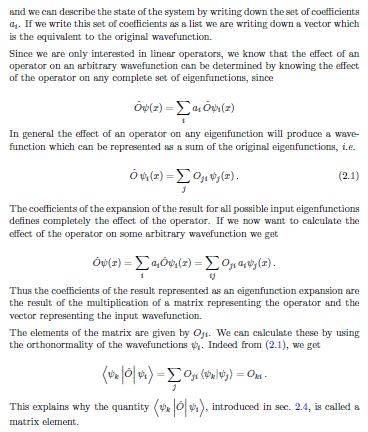
Then comes my problem, just above equation (2.1) we are told

In general the effect of an operator on any eigenfunction will produce a wavefunction which can be represented as a sum of the original eigenfunctions, i.e.

From my understanding the quote says to further expand the result of the operator acting on each of the eigenfunctions we used to expand the generic wavefunction. Also basing that off the use of the subscript i on the LHS of (2.1) and the general flow of where the notes seems to be taking me. Part of my issue is how that is represented in (2.1). What I think (shakily) is happening is that we have applied the operator to the eigenfunction, don't exactly know if the eigenfunction is a eigenfunction of that generic operator, and it will produce a wavefuction which can be expanded using the same eigenfunctions that we used in the first expansion of the generic wavefunction. And here the O_ji I believe is the coefficient of the each of the eigenfunctions?

Then putting it all together in the equation below (2.1) is what makes me question what I'm working with here. The operator O is working on a generic wavefunction, that's equal to O working on each eigenfunction that we break the wavefunction into then we get the RHS of (2.1) except we are we are expanding every eigenfunction and we also have the a_j coefficient from the original expansion. This is somehow supposed to show me that O_ji contains the effect of the operator. I can't seem to see how this works. Will be grateful for any help you can give!

 

[tnich]

I think $\bar O$ is just an arbitrary operator that looks like this: $\bar O = \sum_{jm} | \psi_j \rangle O_{jm} \langle \psi_m |$. Try substituting that into each of the equations in your text and see if they make sense.