- #1
caffeine
Homework Statement
Let X and Y be random variables. The pdfs are [itex]f_X(x)=2(1-x)[/itex] and [itex]f_Y(y) = 2(1-y)[/itex]. Both distributions are defined on [0,1].
Let Z = X + Y. Find the pdf for Z, [itex]f_Z(z)[/itex].
Homework Equations
I'm using ideas, not equations.
The Attempt at a Solution
I'm dying of curiosity about where I'm going wrong. I'm so sure of each step, but my answer can't be correct because [itex]\int_0^2 f_Z(z)\,dz[/itex] is zero! Here's my logic.
Consider the cdf (cumulative distribution function) for Z:
[tex]
F_Z(z) = P(Z\le z) = P(X+Y \le z)
[/tex]
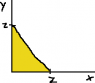
Here, [itex]F_Z(z)[/itex] is the volume above the triangle shown in the image I attached to this message (in case something happens to the attachment, it's the triangle in quadrant 1 bounded by x=0, y=0 and x+y=z.)
The volume above the shaded region represents [itex]F_Z(z)[/itex].
[tex]
F_Z(z) = \int_{x=0}^{x=z} 2(1-x)\int_{y=0}^{y=z-x} 2(1-y)\,dy\,dx
[/tex]
Performing the integrals gives [itex]F_Z(z) = \frac{1}{6}z^4 - \frac{4}{3}z^3 + 2z^2[/itex]. Then taking the derivative of the cdf gives the pdf:
[tex]
f_Z(z) = \partial_z F_Z(z) = \frac{2}{3}z^3 - 4z^2 +4z
[/tex]
Unfortunately, this can't be right because the integral of this function over [0,2] gives zero.
I also would've expected that the maximum of [itex]f_Z(z)[/itex] would be at z=0 since individually, X and Y are most likely to be zero.
I checked my algebra and calculus with Mathematica; it looked fine. I think there's a conceptual problem. There must be something I don't understand or some point I'm not clear about.
What did I do wrong?
Attachments
Last edited by a moderator: