- #1
Dell
- 590
- 0
in a given shaft with a circular cross section, the radius changes linearly
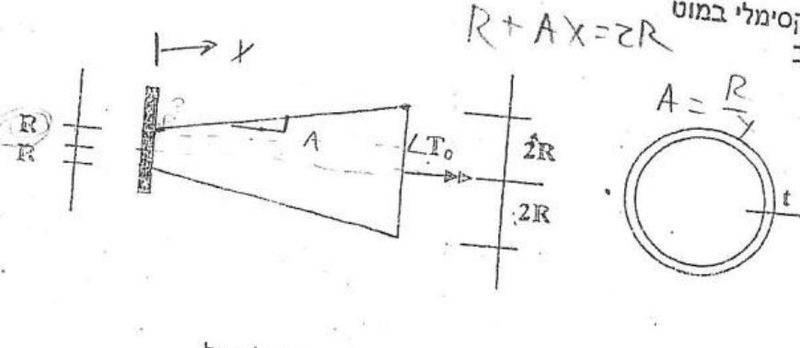
find the maximum shear stress
i used T*r/J
and since J is dependant on r^4, i found that the cross section with the smallest radius will feel the largest stress.
find the angle of twist at the end of the shaft
T/(GJ)*dx while J is a function of X and i integrate from 0 to L
if the bottom of the shaft is reinforced, -thickened- to 2t, what is the largest shear stress felt in the shaft??
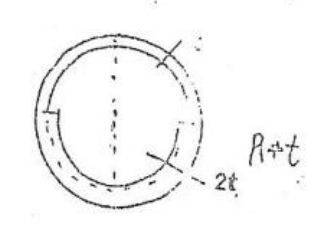
up till now i have only solved questions with either circular/ rectangular closed cross sections, or other open cross sections
can i use the equation for maximum stress
T/(2A*t)
where A is the area surrounded by an axis through the center of the side of the shape?? if so how do i do this ? what would that axis look like? would it be 2 half circles with 90 degree joints? meaning the area would be pi/2*(R12+R22) where R1 and R2 are the average radii of the 2 half circles??
using this logic i would find the maximum stress in the thin walled circle
is this correct? can i do this?
this is where i am stumped,
find the maximum shear stress
i used T*r/J
and since J is dependant on r^4, i found that the cross section with the smallest radius will feel the largest stress.
find the angle of twist at the end of the shaft
T/(GJ)*dx while J is a function of X and i integrate from 0 to L
if the bottom of the shaft is reinforced, -thickened- to 2t, what is the largest shear stress felt in the shaft??
up till now i have only solved questions with either circular/ rectangular closed cross sections, or other open cross sections
can i use the equation for maximum stress
T/(2A*t)
where A is the area surrounded by an axis through the center of the side of the shape?? if so how do i do this ? what would that axis look like? would it be 2 half circles with 90 degree joints? meaning the area would be pi/2*(R12+R22) where R1 and R2 are the average radii of the 2 half circles??
using this logic i would find the maximum stress in the thin walled circle
is this correct? can i do this?
this is where i am stumped,