- #1
jkthejetplane
- 29
- 4
- Homework Statement
- Find the general series solution for laplace in cylindrical coordinates
- Relevant Equations
- for this i have always used (s,phi,z)
Here is the initial problem and my attempt at getting Laplace solution. I get lost near the end and after some research, ended up with the Bessel equation and function. I don't completely understand what this is or even if this i the direction I go in.
This is a supplemental thing that I want to nail down for review to get my brain up to speed again for this semester
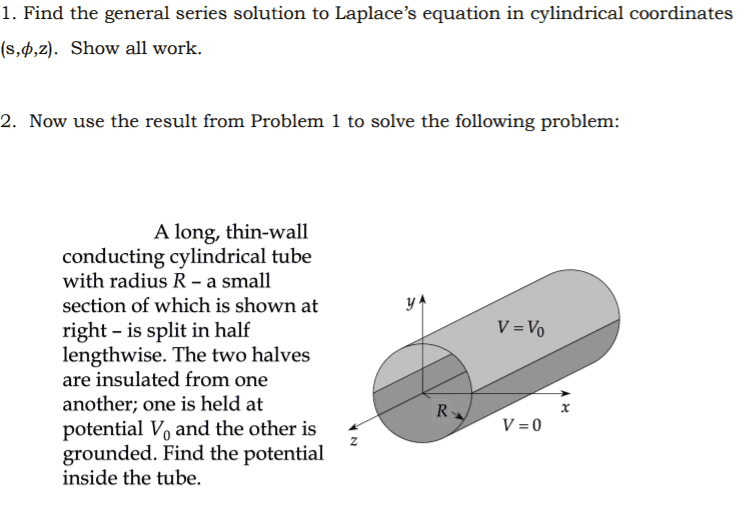

This is a supplemental thing that I want to nail down for review to get my brain up to speed again for this semester
Attachments
Last edited by a moderator: