Fermat's Little Theorem .... Anderson and Feil, Theorem 8.7 .

[Math Amateur]

I am reading Anderson and Feil - A First Course in Abstract Algebra.

I am currently focused on Ch. 8: Integral Domains and Fields ...

I need some help with an aspect of the proof of Theorem 8.7 (Fermat's Little Theorem) ...

Theorem 8.7 and its proof read as follows:

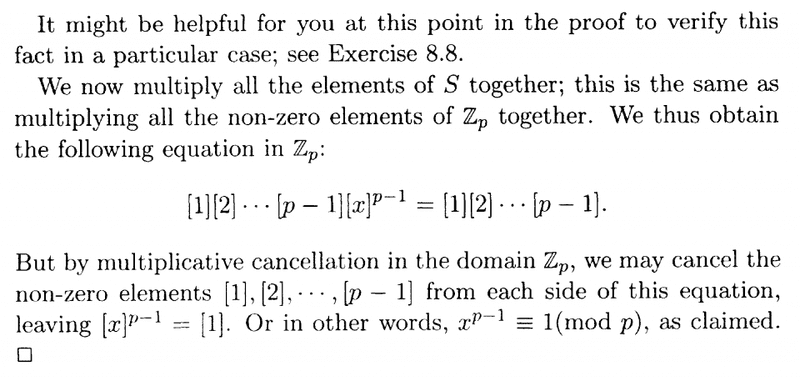My questions regarding the above are as follows:
Question 1

In the above text from Anderson and Feil we read the following:

" ... ... Because a field has no zero divisors, each element of $S$ is non-zero ... "Can someone please demonstrate exactly why this follows ... ?

Question 2

In the above text from Anderson and Feil we read the following:" ... ... Because a field satisfies multiplicative cancellation, no two of these elements are the same .. ... "Can someone please demonstrate exactly why it follows that no two of the elements of $S$ are the same .. ... "
Help will be appreciated ...

Peter*** EDIT ***

oh dear ... can see that the answer to Question 1 is obvious ... indeed it follows from the definition of zero divisor ... apologies ... brain not in gear ...

 

[fresh_42]

Question 2
In the above text from Anderson and Feil we read the following:
" ... ... Because a field satisfies multiplicative cancellation, no two of these elements are the same .. ... "
Can someone please demonstrate exactly why it follows that no two of the elements of $S$ are the same .. ... "

It's also obvious. If we assumed $[x\cdot n]=[x\cdot m]$ then what would this mean? You can use all field operations and again the lack of zero divisors. In general, the question to be answered is: why do fields allow multiplicative cancellations? The reason doesn't require this special field, so you may as well prove: $a\cdot m = b \cdot m \Longrightarrow a = b$ for $a,b \neq 0$ of course.

 

[Math Amateur]

Thanks for the help fresh_42 ... grateful for your help ...

If $[ x \cdot n ] = [ x \cdot m ]$ then by cancellation in a field, we have n = m which cannot be the case for the members of the set S ...

Cancellation works in fields since every element of a field has an inverse ...

... so if $a \cdot m = b \cdot m$ then we can post-multiply by $m^{-1}$ and get $a = b$ ...

Hope that's right ...

Peter

 

[fresh_42]

Thanks for the help fresh_42 ... grateful for your help ...

If $[ x \cdot n ] = [ x \cdot m ]$ then by cancellation in a field, we have n = m which cannot be the case for the members of the set S ...

Cancellation works in fields since every element of a field has an inverse ...

... so if $a \cdot m = b \cdot m$ then we can post-multiply by $m^{-1}$ and get $a = b$ ...

Hope that's right ...

Peter

Well, partially. You cannot rule out the case $m=0$ at prior and operate with $m^{-1}$.

Therefore wait as long as you can with additional assumptions.

This is more of a general advice. Instead operate without (multiplicative) inverse elements.