- #1
Niles
- 1,866
- 0
Most liquids can be assumed to be incompressible, since the Mach-number is much smaller than 1. That means that the density variations are negligible and from the relation between pressure p and density ρ,
[tex]
p=c_s^2 \rho
[/tex]
we see that the pressure in constant as well. Now, say that I look at a pipe with the following geometry:
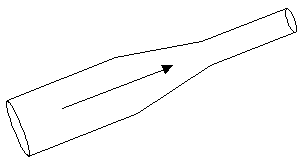
From Bernoulli's equation we get that the pressure and velocity will be different between the large-radius part of the pipe and the small-radius part. How does this varying pressure conform with the constant pressure/density obtained from the equation of state?
[tex]
p=c_s^2 \rho
[/tex]
we see that the pressure in constant as well. Now, say that I look at a pipe with the following geometry:
From Bernoulli's equation we get that the pressure and velocity will be different between the large-radius part of the pipe and the small-radius part. How does this varying pressure conform with the constant pressure/density obtained from the equation of state?