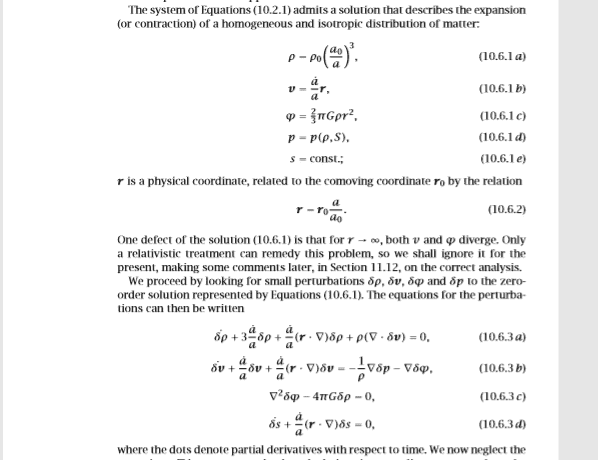
- #1
- 2,168
- 193
I am trying to derive the equation for a case, where we have a dust(zero-pressure) in an expanding universe.
There are 4 equations but I think exercising on one of them would be helpful for me.
I am trying to derive the equation for a case, where we have a dust(zero-pressure) in an expanding universe.
There are 4 equations but I think exercising on one of them would be helpful for me.
So we have a continuity equation
$$\frac{\partial \rho}{\partial t} + \nabla \cdot (\rho \vec{v})=0$$
In an expanding universe
##\rho = \rho_0a^{-3}##
##\vec{v} = \frac{\dot{a}}{a}\vec{r}##
##\vec{r} = \vec{r_0}a##
where ##a=a(t)##
So the problem is I am not sure how to define the perturbation
I thought I can write
$$\rho = \rho_0a^{-3} + \delta \rho$$
$$\vec{v} = \frac{\dot{a}}{a}\vec{r} + \delta \vec{v}$$
If I put it into the equation I get
$$\frac{\partial }{\partial t}(\rho_0a^{-3} + \delta \rho) + \nabla \cdot ((\rho_0a^{-3} + \delta \rho)(\frac{\dot{a}}{a}\vec{r} + \delta \vec{v}))=0$$
$$\frac{\partial }{\partial t}(\rho_0a^{-3}) + \frac{\partial }{\partial t}(\delta \rho) + \nabla \cdot (\rho_0a^{-3}\frac{\dot{a}}{a}\vec{r} + \rho_0a^{-3}\delta \vec{v}+ \delta \rho\frac{\dot{a}}{a}\vec{r})=0$$
since ##\rho = \rho_0a^{-3}##
$$\frac{\partial }{\partial t}(\rho_0a^{-3})+\frac{\partial }{\partial t}(\delta \rho) + \nabla \cdot (\rho\frac{\dot{a}}{a}\vec{r} + \rho\delta \vec{v}+ \delta \rho\frac{\dot{a}}{a}\vec{r})=0$$
$$\frac{\partial }{\partial t}(\rho_0a^{-3})+\frac{\partial }{\partial t}(\delta \rho) + \rho\frac{\dot{a}}{a} (\nabla \cdot \vec{r}) + \rho(\nabla \cdot \delta \vec{v})+ \delta \rho\frac{\dot{a}}{a} (\nabla \cdot \vec{r})=0$$The answer is
$$\frac{\partial }{\partial t}(\delta \rho) + 3\delta \rho\frac{\dot{a}}{a}+ \rho(\nabla \cdot \delta \vec{v})+ \delta \rho\frac{\dot{a}}{a} (\vec{r} \cdot \nabla )=0$$
Also ##(\nabla \cdot \vec{r}) = 3 ?##
For reference : https://www.amazon.com/dp/0471489093/?tag=pfamazon01-20 Page 216

There are 4 equations but I think exercising on one of them would be helpful for me.
I am trying to derive the equation for a case, where we have a dust(zero-pressure) in an expanding universe.
There are 4 equations but I think exercising on one of them would be helpful for me.
So we have a continuity equation
$$\frac{\partial \rho}{\partial t} + \nabla \cdot (\rho \vec{v})=0$$
In an expanding universe
##\rho = \rho_0a^{-3}##
##\vec{v} = \frac{\dot{a}}{a}\vec{r}##
##\vec{r} = \vec{r_0}a##
where ##a=a(t)##
So the problem is I am not sure how to define the perturbation
I thought I can write
$$\rho = \rho_0a^{-3} + \delta \rho$$
$$\vec{v} = \frac{\dot{a}}{a}\vec{r} + \delta \vec{v}$$
If I put it into the equation I get
$$\frac{\partial }{\partial t}(\rho_0a^{-3} + \delta \rho) + \nabla \cdot ((\rho_0a^{-3} + \delta \rho)(\frac{\dot{a}}{a}\vec{r} + \delta \vec{v}))=0$$
$$\frac{\partial }{\partial t}(\rho_0a^{-3}) + \frac{\partial }{\partial t}(\delta \rho) + \nabla \cdot (\rho_0a^{-3}\frac{\dot{a}}{a}\vec{r} + \rho_0a^{-3}\delta \vec{v}+ \delta \rho\frac{\dot{a}}{a}\vec{r})=0$$
since ##\rho = \rho_0a^{-3}##
$$\frac{\partial }{\partial t}(\rho_0a^{-3})+\frac{\partial }{\partial t}(\delta \rho) + \nabla \cdot (\rho\frac{\dot{a}}{a}\vec{r} + \rho\delta \vec{v}+ \delta \rho\frac{\dot{a}}{a}\vec{r})=0$$
$$\frac{\partial }{\partial t}(\rho_0a^{-3})+\frac{\partial }{\partial t}(\delta \rho) + \rho\frac{\dot{a}}{a} (\nabla \cdot \vec{r}) + \rho(\nabla \cdot \delta \vec{v})+ \delta \rho\frac{\dot{a}}{a} (\nabla \cdot \vec{r})=0$$The answer is
$$\frac{\partial }{\partial t}(\delta \rho) + 3\delta \rho\frac{\dot{a}}{a}+ \rho(\nabla \cdot \delta \vec{v})+ \delta \rho\frac{\dot{a}}{a} (\vec{r} \cdot \nabla )=0$$
Also ##(\nabla \cdot \vec{r}) = 3 ?##
For reference : https://www.amazon.com/dp/0471489093/?tag=pfamazon01-20 Page 216
Last edited: