Line integral of a vector field (Polar coordinate)

[Lambda96]

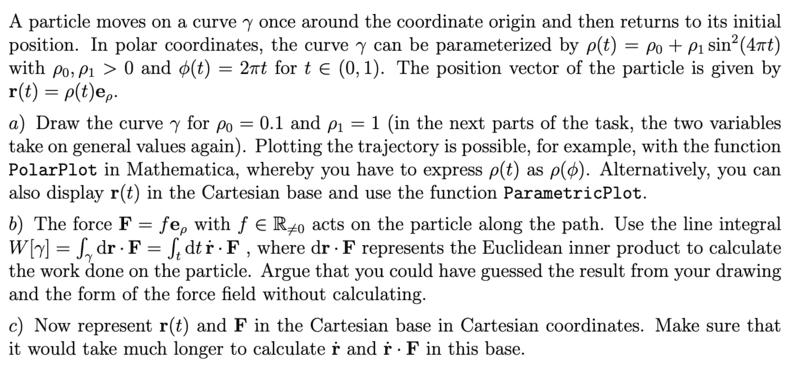
Homework Statement

Calculate the work done, which acts on the particle

Relevant Equations

none

Hi,

I am not sure if I have solved task b correctly

According to the task, $\textbf{F}=f \vec{e}_{\rho}$ which in Cartesian coordinates is $\textbf{F}=f \vec{e}_{\rho}= \left(\begin{array}{c} \cos(\phi) \\ \sin(\phi) \end{array}\right)$ since $f \in \mathbb{R}_{\neq 0}$ is constant, $\textbf{F}$ would simply be $f$ in polar coordinates, wouldn't it?

$\dot{r}(t)$ would be $\dot{\rho}(t)$ and therefore $\dot{\rho}(t)=8 \pi \sin(4 \pi t) cos(4 \pi t)$

The line integral is:

$\int_{0}^{1} dt f \cdot 8 \pi \sin(4 \pi t) cos(4 \pi t)=0$

 

[TSny]

According to the task, $\textbf{F}=f \vec{e}_{\rho}$ which in Cartesian coordinates is $\textbf{F}=f \vec{e}_{\rho}= \left(\begin{array}{c} \cos(\phi) \\ \sin(\phi) \end{array}\right)$

Did you leave out a factor of $f$ in the expression on the far right?

For Cartesian coordinates, I would express the force in the form $\textbf{F}=(...) \textbf e_x + (...) \textbf e_y$.
For part (b) you are staying in polar coordinates.

$\textbf{F}$ would simply be $f$ in polar coordinates, wouldn't it?

$\textbf F$ is a vector while $f$ is a scalar. So, they can't be equal. In polar coordinates, you would express the force in the form $\textbf{F}=(...) \textbf e_{\rho} + (...) \textbf e_{\phi}$.

$\dot{r}(t)$ would be $\dot{\rho}(t)$ and therefore $\dot{\rho}(t)=8 \pi \sin(4 \pi t) cos(4 \pi t)$

The line integral is:

$\int_{0}^{1} dt f \cdot 8 \pi \sin(4 \pi t) cos(4 \pi t)=0$

I think your line integral expression is correct.

However, it might be good to show your instructor how the integrand $\dot{\mathbf r} \cdot \mathbf F$ reduces to your expression. Thus, how would you write the vector $\dot{\mathbf r}$ in polar coordinate form $(...) \textbf e_{\rho} + (...) \textbf e_{\phi}$? Then you can take the dot product $\dot{\mathbf r} \cdot \mathbf F$.

In part (a) you considered the case where $\rho_0 = 0.1$ and $\rho_1 = 1$. But it said to let $\rho_0$ and $\rho_1$ take on general values again in parts (b) and (c).

 

[Lambda96]

Thank you TSny for your help

Did you leave out a factor of $f$ in the expression on the far right?

For Cartesian coordinates, I would express the force in the form $\textbf{F}=(...) \textbf e_x + (...) \textbf e_y$.
For part (b) you are staying in polar coordinates.

You're right, unfortunately, I had forgotten the f on the right-hand side of the equation.

In Cartesian coordinates, $\textbf{F}$ would then be $\textbf{F}=f \cos(\phi) \textbf{e}_x + f \sin(\phi) \textbf{e}_y$

$\textbf{Task b}$

$\dot{\textbf{r}}$ would have to be derived using the chain rule, so it would look like this

$\dot{\textbf{r} }= \dot{\rho}(t) \textbf{e}_{\rho} + \rho(t) \dot{\phi}(t) \textbf{e}_{\phi}$

I'm still a little unsure about $\textbf{F}$, would this be as follows?

$\textbf{F}=f \textbf{e}_{\rho} + 0 \textbf{e}_{\phi}$

Then, the scalar product of $\dot{\textbf{r}} \cdot \textbf{F}=f \dot{\rho}(t)$ since $\textbf{e}_{\rho}$ and $\textbf{e}_{\phi}$ are orthogonal to each other.

Is that correct?

 

[TSny]

That all looks very good to me.

 

[Lambda96]

Thank you TSny once again for your help and explanation, which helped me a lot