Noetherian Modules and Submodules

[Math Amateur]

I am reading J A Beachy's Book, Introductory Lectures on Rings and Modules"... ...

I am currently focused on Chapter 2: Modules ... and in particular Section 2.4: Chain Conditions ...

I need help with the proof of Proposition 2.4.5 ...

Proposition 2.4.5 reads as follows:

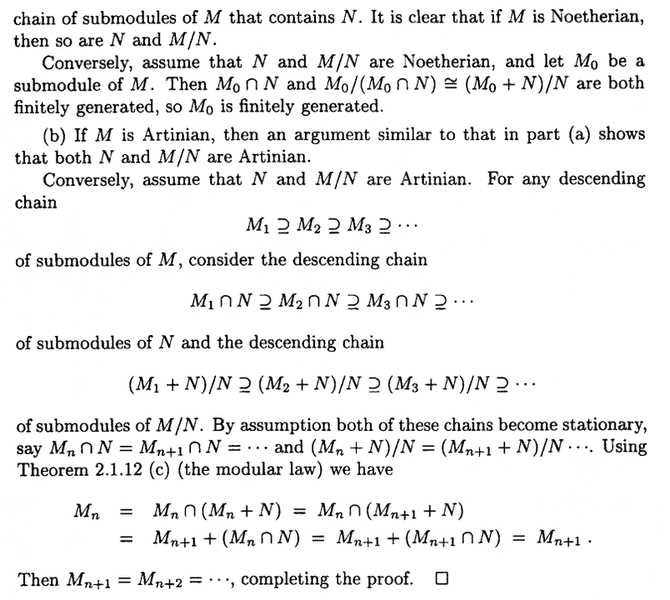
In the above text by Beachy ... in the proof of part (a) ... we read the following:

"... ... ... Conversely, assume that $N$ and $M/N$ are Noetherian, and let $M_0$ be a submodule of $M$. Then $M_0 \cap N$ and $M_0 / ( M_0 \cap N ) \cong (M_0 + N) / N$ are both finitely generated, so $M_0$ is finitely generated ... ... ...
I am very unsure of this part of the proof ... but overall Beachy seems to be trying to prove that an arbitrary submodule of $M$, namely $M_0$, is finitely generated ... ... and this means that $M$ is Noetherian ...

(Beachy, in his Proposition 2.4.3 has shown that every submodule of $M$ being finitely generated is equivalent to $M$ being Noetherian ... ... )

BUT ... I do not see how it follows in the above that ... ... $M_0 \cap N$ and $M_0 / ( M_0 \cap N ) \cong (M_0 + N) / N$ are both finitely generated ... ... AND ... exactly why it then follows that $M_0$ is finitely generated ... ...

Hope someone can help ...

Peter

 

[fresh_42]

You might reconsider what those cosets are.

Let me start with an example with the integers again, i.e. $R=\mathbb{Z}$.
Then $M=6\mathbb{Z}$ is a submodule. Now we can write $\mathbb{Z} = 6\mathbb{Z}\cup(1+6\mathbb{Z})\cup(2+6\mathbb{Z})\cup(3+6\mathbb{Z})\cup(4+6\mathbb{Z})\cup(5+6\mathbb{Z})$, that is every integer is exactly in one of those six sets. They are called cosets with respect to $M$, which itself corresponds to $0+6\mathbb{Z}$.

The representatives $\{0_M,1_M,2_M,3_M,4_M,5_M\}$ of these cosets form again a module. Not a submodule! Its elements are (co-)sets which are only represented by these six numbers $n_M$. For short we write $R / M = \mathbb{Z}/ 6\mathbb{Z} \cong \mathbb{Z}_6$ because they behave like the remainders of integer division by $6$.

Those modules are called quotient modules (and sometimes also factor modules - a matter of taste).

What is useful to bear in mind for the proof above, is the correspondence theorem (if I remember its name correctly) and the isomorphism theorems in connection with the short exact sequence $N \rightarrowtail M \twoheadrightarrow M/N\,$.

To practice you could think about the submodules in the example above, that is the submodules of $M=6\mathbb{Z}$ and the submodules of the quotient module $R / M = \mathbb{Z}/6 \mathbb{Z}$. Why must the latter contain $M$?

With respect to your question:

Conversely, assume that $N$ and $M/N$ are Noetherian

$M_0$ is a submodule of $M \;$, $M_0 / (M_0 \cap N)$ a quotient module of $M_0$.

(Beachy, in his Proposition 2.4.3 has shown that every submodule of $M$ being finitely generated is equivalent to $M$ being Noetherian ... ... )

 

[andrewkirk]

I wonder whether this one requires using the axiom of choice (AC). I note that certain equivalences in this area do require using AC.

I had a go at proving the $M_0\cap N$ one and came up against an apparent need to make an infinite number of choices.

But there may be some result obtained earlier in the text, that has used AC, that can be used in this problem to prove the result, and thereby avoid having to deploy the AC afresh.

@fresh_42
, do you know whether AC is required to prove the $M_0\cap N$ version?

 

[mathwonk]

Assuming N is noetherian, means all submodules are fin gen so the submodule M0meetN is trivially fin gen. same for the quotient, since he uses the basic isomorphism law to equate it with a submodule of the quotien M/N which is assumed noetherian. then knowing that the quotient and the sub are fin gen gives the result since combining generators of the two (actually representatives of generators of the quotient) gives generators of the module M0.

 

[fresh_42]

I wonder whether this one requires using the axiom of choice (AC). I note that certain equivalences in this area do require using AC.

I had a go at proving the $M_0\cap N$ one and came up against an apparent need to make an infinite number of choices.

But there may be some result obtained earlier in the text, that has used AC, that can be used in this problem to prove the result, and thereby avoid having to deploy the AC afresh.

@fresh_42
, do you know whether AC is required to prove the $M_0\cap N$ version?

Not by heart. AC appears as Zorn's Lemma in these contexts and is needed, e.g. to prove the existence of maximal ideals in arbitrary rings. And also in Peter's book the author uses it to guarantee the existence of maximal elements. IMO most authors don't care a lot about it which makes it difficult to detect where it might be hidden.

In the case of Noetherian Rings / Modules to be equivalent to finite generation I think it is needed. At least Peter's book uses it as well as my Atiyah / MacDonald does. In the case above I don't think it's needed. The stationary chains should do.

Edit: It's so common to "algebraic" thinking people, that they usually don't care much. As I read your post I first thought you meant ACC instead of AC.

 

[andrewkirk]

Assuming N is noetherian, means all submodules are fin gen

I couldn't get a clear sense from the OP whether that theorem has been proved yet, and hence can be used, or whether alternatively the above Proposition is a step on the way towards proving that theorem.

Looking at the last bit of (a), it appears to be taking as previously proven that:

. (all submods of $M$ are fin gen) $\Rightarrow$ ($M$ is Noetherian)

But we'd need the converse implication here.

BTW I would guess that only one of those directions requires AC. If so, which is it?

 

[fresh_42]

BTW I would guess that only one of those directions requires AC. If so, which is it?

finitely generated $\Rightarrow$ ACC / Noetherian
Noetherian + AC $\Rightarrow$ finitely generated

 

[Math Amateur]

Thanks to fresh_42, Andrew and mathwonk for help and guidance ...

Current thinking is as follows:Assume that $N$ and $M/N$ are Noetherian ... and let $M_0$ be a submodule of $M$ ...... ... need to show that $M$ is Noetherian ... ...Follow J A Beachy and consider $M_0 \cap N$

All elements of $M_0 \cap N$ belong to $N$ ... and $N$ is finitely generated ...

Therefore some subset of the generating set for $N$ will generate $M_0 \cap N$ ... ...

so, then ... $M_0 \cap N$ is finitely generated ... BUT ... what can we usefully conclude from this ...?

... or more precisely ... how do we use this in proving that $M_0$ is finitely generated ...?Now, again following J A Beachy's proof ... consider $M_0 / ( M_0 \cap N )$

Beachy claims that $M_0 / ( M_0 \cap N )$ is finitely generated ... BUT ... how do we show this ...

... and further ... how do we use this fact to show that $M_0$ is finitely generated?

Now ... Beachy points out that $M_0 / ( M_0 \cap N ) \cong ( M + N ) / N$ ...

So presumably we need to show $( M + N ) / N$ is finitely generated ...

... and then conclude $M_0 / ( M_0 \cap N )$ is finitely generated ...

But how do we do this ... and further ... how do we use this to prove $M_0$ is finitely generated ...?

Hope someone can help ...

Peter

 

[andrewkirk]

All elements of $M_0 \cap N$ belong to $N$ ... and $N$ is finitely generated ...

Therefore some subset of the generating set for $N$ will generate $M_0 \cap N$ ... ...

That assumes that a submodule of a finitely-generated module is finitely-generated, which is not in general true.

If we already have the result that all submodules of a Noetherian module are FG then we can immediately conclude that $M_0\cap N$ is, since it is a submodule of $N$, which we are told is Noetherian. But if we don't already have that result, we cannot take that step without additional argument (the nature of which is not immediately apparent to me, other than that it's probably going to have to involve some strictly ascending chains).

Has it been proven in the text, prior to the Proposition being discussed, that all submodules of a Noetherian module are FG?

 

[fresh_42]

Thanks to fresh_42, Andrew and mathwonk for help and guidance ...

Current thinking is as follows:Assume that $N$ and $M/N$ are Noetherian ... and let $M_0$ be a submodule of $M$ ...... ... need to show that $M$ is Noetherian ... ...Follow J A Beachy and consider $M_0 \cap N$

All elements of $M_0 \cap N$ belong to $N$ ... and $N$ is finitely generated ...

Therefore some subset of the generating set for $N$ will generate $M_0 \cap N$ ... ...

so, then ... $M_0 \cap N$ is finitely generated ... BUT ... what can we usefully conclude from this ...?

... or more precisely ... how do we use this in proving that $M_0$ is finitely generated ...?

Now, again following J A Beachy's proof ... consider $M_0 / ( M_0 \cap N )$

Beachy claims that $M_0 / ( M_0 \cap N )$ is finitely generated ... BUT ... how do we show this ...

... and further ... how do we use this fact to show that $M_0$ is finitely generated?

Now ... Beachy points out that $M_0 / ( M_0 \cap N ) \cong ( M + N ) / N$ ...

So presumably we need to show $( M + N ) / N$ is finitely generated ...

... and then conclude $M_0 / ( M_0 \cap N )$ is finitely generated ...

But how do we do this ... and further ... how do we use this to prove $M_0$ is finitely generated ...?

Hope someone can help ...

Peter

By the isomorphism theorem $M_0 / ( M_0 \cap N ) \cong (M_0 +N / N) \subseteq M / N$ is a submodule.

Submodules of finitely generated modules are finitely generated.

Therefore not only $M_0 \cap N$ is finitely generated, but also $M_0 / (M_0 \cap N)$ since $M/N$ is.

Now I assume that there has been a proposition that says:

If $A \rightarrowtail B \twoheadrightarrow C$ is short exact and $A$ and $C$ are finitely generated, so is $B$.
Applied to $A=M_0 \cap N\, , \, B=M_0 \, , \, C= M_0 / ( M_0 \cap N )$ it means $M_0$ is finitely generated.

As a personal remark, I don't understand, why we need a submodule $M_0$ of $M$ instead of $M$ in the first place.
I would have expected an induction on the number of generators though (starting with $\{0\}$).
In addition and having the discussion above about Zorn's Lemma in mind, I think a proof using only chains and the ascending chain conditions on $N$ and $M/N$ instead of generators would have been better in this case. (You might try this as an exercise.)

@andrewkirk He already has the equivalence of Noetherian and FG (Prop. 2.4.3). So only "submodules and quotient modules of FG modules are FG and vice versa" is needed. But I don't understand the role of $M_0$ either.

Edit: However, if you already have
"Given the exact sequence $\{0\} \rightarrow A \rightarrow B \rightarrow C \rightarrow \{0\}$ then $B$ is finitely generated if and only if $A$ and $C$ are finitely generated." (*)
which Beachy apparently uses, then together with the equivalence from Proposition 2.4.3 there is nothing left to show, since (*) is the exact same (equivalent) statement as Prop. 2.4.5 (a).

 

[andrewkirk]

Submodules of finitely generated modules are finitely generated

For Noetherian modules, yes.
For modules in general, no. See for instance here. There's a better example in my Hartley and Hawkes, but that's at home and I'm at work.

@andrewkirk He already has the equivalence of Noetherian and FG (Prop. 2.4.3).

Ah, so he has. I didn't notice that comment in the OP. Given that, the FG-ness of $M_0\cap N$ is immediate.

 

[fresh_42]

For Noetherian modules, yes.
For modules in general, no. See for instance here. There's a better example in my Hartley and Hawkes, but that's at home and I'm at work.

Interesting, thanks.

 

[Math Amateur]

By the isomorphism theorem $M_0 / ( M_0 \cap N ) \cong (M_0 +N / N) \subseteq M / N$ is a submodule.

Submodules of finitely generated modules are finitely generated.

Therefore not only $M_0 \cap N$ is finitely generated, but also $M_0 / (M_0 \cap N)$ since $M/N$ is.

Now I assume that there has been a proposition that says:

If $A \rightarrowtail B \twoheadrightarrow C$ is short exact and $A$ and $C$ are finitely generated, so is $B$.
Applied to $A=M_0 \cap N\, , \, B=M_0 \, , \, C= M_0 / ( M_0 \cap N )$ it means $M_0$ is finitely generated.

As a personal remark, I don't understand, why we need a submodule $M_0$ of $M$ instead of $M$ in the first place.
I would have expected an induction on the number of generators though (starting with $\{0\}$).
In addition and having the discussion above about Zorn's Lemma in mind, I think a proof using only chains and the ascending chain conditions on $N$ and $M/N$ instead of generators would have been better in this case. (You might try this as an exercise.)

@andrewkirk He already has the equivalence of Noetherian and FG (Prop. 2.4.3). So only "submodules and quotient modules of FG modules are FG and vice versa" is needed. But I don't understand the role of $M_0$ either.

Edit: However, if you already have
"Given the exact sequence $\{0\} \rightarrow A \rightarrow B \rightarrow C \rightarrow \{0\}$ then $B$ is finitely generated if and only if $A$ and $C$ are finitely generated." (*)
which Beachy apparently uses, then together with the equivalence from Proposition 2.4.3 there is nothing left to show, since (*) is the exact same (equivalent) statement as Prop. 2.4.5 (a).

Thanks for the above post fresh_42 ...

You write:

"... ... By the isomorphism theorem $M_0 / ( M_0 \cap N ) \cong (M_0 +N / N) \subseteq M / N$ is a submodule. ... .. "

How do we know that $(M_0 +N / N)$ is a submodule of $M / N$ ... ... ?

Peter

 

[fresh_42]

Thanks for the above post fresh_42 ...

You write:

"... ... By the isomorphism theorem $M_0 / ( M_0 \cap N ) \cong (M_0 +N / N) \subseteq M / N$ is a submodule. ... .. "

How do we know that $(M_0 +N / N)$ is a submodule of $M / N$ ... ... ?

Peter

There is a small parenthesis sloppyness from my side. It better would have been written $(M_0 +N) / N$.
$M_0 +N$ is a submodule of $M$ that contains $N$. So $(M_0 +N) / N$ is a submodule of $M/N$.
I think it has been called correspondence principle in your books, but I'm not sure.

You can simply write down the arithmetic in $(M_0 +N) / N$:

$(a+N)+(b+N)=(a+b)+N\, , \,(a,b \in M_0+N)$
$r(a+N)=ra+rN=ra+N\, , \,(a \in M_0+N\, , \,r\in R)$

What has to be shown is the well-definition of them, i.e. that one gets the same result for different representatives.
That is $(a+N)+(b+N)=(a'+N)+(b'+N)$ for $a'=a+n_a\, , \,b'=b+n_b$ and $r(a+N)=ra'+N$. Because we are dealing with cosets here, which are sets, their representatives may vary. In $(M_0+N)/N$ (and all other quotient objects), $a+N$ and $a'+N$ is the same element iff $a-a' \in N$.

 

[steenis]

1) $N$ is noetherian and $M_0 \cap N \leq N$, so $M_0 \cap N$ is fingen (finitely generated).

2) $M/N$ is noetherian and $(M_0 + N)/N \leq M/N$, so $(M_0 + N)/N$ is fingen.

3) $M_0/(M_0 \cap N) \cong (M_0 + N)/N$, so $M_0/(M_0 \cap N$ is fingen.

4) $M_0 \cap N$ is fingen and $M_0/(M_0 \cap N)$ is fingen, thus $M_0$ is fingen, by a remark of Beachy on page.70.

 

[mathwonk]

I am kind of worried about the fact that anyone would ask why the non existence of an infinite ascending chain of submodules implies all submodules are finitely generated. That is about the most automatic self proving statement I can think of. I guess you do have to have the reflex to prove something by negation, but please just try this, if you think this is a problem. some day a chick has to fly.