Solving for ##\theta## in a Complex Grinding Problem

[Mister T]

Homework Statement

Solve for $\theta$:

$\cot \theta \sin \beta + \rho \csc^2 \theta = \cos \beta$

where $0^\circ<\beta<90^\circ, \ 0^\circ<\theta<90^\circ$, and $0<\rho<1$.

Homework Equations

$\cot^2 x +1 = \csc^2x$, the quadratic formula.

The Attempt at a Solution

$\cot \theta = \frac{\sin \beta}{2 \rho} \pm \sqrt{\Big(\frac{\sin \beta}{2 \rho}\Big)^2 + \frac{\cos \beta}{ \rho}-1}$
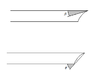
This is not really a homework question, but since it looks like one I decided to post it here. It's actually a relationship between the variables involved in grinding an edge tool such as a carpenter's chisel. $\rho$ is the ratio of the tool's thickness to the diameter of the circular grindstone, so it's a positive constant, usually much smaller than unity. The issue is that a round grindstone produces a hollow grind rather than the flat surface you'd get sharpening on a flat stone, and I'm interested in the geometry of the hollow grind. $\beta$ is the bevel angle, or angle that the two edges make when they meet at the cutting edge.

$\theta$ is the angle the two edges would make if the grinding were done on a flat surface.

My solution seems to work as it gives results that match what I measure and also that a friend got using AutoCAD or some such software program. There are two roots to the solution, though. The positive root gives the value of $\theta$ but the negative root gives the supplement of $\theta-\beta$. I realize this negative root is outside the bounds of $\theta$ but it is nevertheless a curiosity to me.

So I have two questions. Can anyone derive a simpler solution and can anyone explain the negative root's value?

 

[haruspex]

Not sure how you arrived at your equation. I got an equivalent equation, but it came out in a more useful form for finding theta.
Hint: try working it into a form that involves trig functions of 2θ rather than θ.

 

[Mister T]

Not sure how you arrived at your equation.

Starting with

$\cot \theta \sin \beta + \rho \csc^2 \theta = \cos \beta$

I replaced $\csc^2 \theta$ with $\cot^2 \theta +1$.

That gave me a quadratic equation in $\cot \theta$.

I then used the quadratic formula to get

$\cot \theta = \frac{\sin \beta}{2 \rho} \pm \sqrt{\Big(\frac{\sin \beta}{2 \rho}\Big)^2 + \frac{\cos \beta}{ \rho}-1}$.

I got an equivalent equation, but it came out in a more useful form for finding theta.
Hint: try working it into a form that involves trig functions of 2θ rather than θ.

I tried replacing $\cot \theta$ with $\pm \sqrt{\frac{1+\cos 2\theta}{1-\cos 2\theta}}$

and $\csc^2 \theta$ with $\frac{2}{1-\cos 2\theta}$

but that seemed to go nowhere.

 

[haruspex]

Starting with

$\cot \theta \sin \beta + \rho \csc^2 \theta = \cos \beta$

I replaced $\csc^2 \theta$ with $\cot^2 \theta +1$.

That gave me a quadratic equation in $\cot \theta$.

I then used the quadratic formula to get

$\cot \theta = \frac{\sin \beta}{2 \rho} \pm \sqrt{\Big(\frac{\sin \beta}{2 \rho}\Big)^2 + \frac{\cos \beta}{ \rho}-1}$.
I tried replacing $\cot \theta$ with $\pm \sqrt{\frac{1+\cos 2\theta}{1-\cos 2\theta}}$

and $\csc^2 \theta$ with $\frac{2}{1-\cos 2\theta}$

but that seemed to go nowhere.

OK. From the geometry, I got
$\rho=\cos(\beta)-\cos(2\theta-\beta)$
which results in
$\theta = \frac 12(\beta+\cos^{-1}(\cos(\beta)-\rho))$
It seems to be the same functionally.

The spurious negative value in this form comes from the ambiguity of $\cos^{-1}$. Generally these nonphysical solutions arise because the equations you wrote down apply in some other set-up also. Maybe here it represents the curvature going the other way.

 

[Mister T]

OK. From the geometry, I got
$\rho=\cos(\beta)-\cos(2\theta-\beta)$

Hmmm... So does this mean you were looking at some triangles with angles of $\beta$ and $2 \theta$?

Can you help me reconstruct what you did?

 

[Mister T]

OK. From the geometry, I got
$\rho=\cos(\beta)-\cos(2\theta-\beta)$
which results in
$\theta = \frac 12(\beta+\cos^{-1}(\cos(\beta)-\rho))$

I think these should be
$2\rho=\cos(\beta)-\cos(2\theta-\beta)$
which results in
$\theta = \frac 12(\beta+\cos^{-1}(\cos(\beta)-2\rho))$

I just now found this when I checked things with numerical examples.

 

[haruspex]

I think these should be
$2\rho=\cos(\beta)-\cos(2\theta-\beta)$
which results in
$\theta = \frac 12(\beta+\cos^{-1}(\cos(\beta)-2\rho))$

I just now found this when I checked things with numerical examples.

Ah, yes. I had ρ as the ratio of the thickness to the radius, not to the diameter.
Let the tool edge be A, the obtuse angle (the other end of the arc) be B, the midpoint of the arc C, and the centre of the circle O. Call the upper surface of the metal AA' and the lower BB', the distance A'B' being the thickness h. Radius = AO=BO=r.
Angle A'AB is θ. The tangent to the arc at A makes angle β with AA'.
The tangent to the arc at C makes angle θ to AA', so θ-β to the first tangent.
The tangent to the arc at B makes angle θ-β to the second tangent, so 2θ-β to AA'.
It follows that AOB is 2θ-β.
Angle OAA' is β+π/2.
h=r cos(β)- r cos(2θ-β)

 

[Mister T]

The tangent to the arc at C makes angle θ to AA', so θ-β to the first tangent.
The tangent to the arc at B makes angle θ-β to the second tangent, so 2θ-β to AA'.

Yes! This is the piece of the geometry I needed to realize. Thank you!

In other words, let us look at the angle between a tangent line (that is, a line tangent to the rim of the grindstone) and a line parallel to the upper surface of the tool. At the point on the rim touched by the upper surface of the tool this angle equals $\beta$ (by definition). As we move along the rim towards the lower surface of the tool this angle increases. It's value halfway along is $\theta$ (because there the tangent line is parallel to the chord that cuts across the hollow grind!). Thus it increased by $\theta-\beta$. And therefore by symmetry its value must again increase by $\theta-\beta$ as we proceed the remaining halfway to the point on the rim touched by the lower surface of the tool. Thus its value at that point is $2\theta-\beta$.

(Note that I am using t for the tool thickness rather than the h that you've used).

It follows that AOB is 2θ-β.

Hmmm... If I've followed your description it seems it would be $2\theta-2\beta$.

 

[haruspex]

Hmmm... If I've followed your description it seems it would be 2θ−2β.

Yes, sorry, I didn't mean AOB. You can see what I meant... the angle radius OB makes to the "vertical".