- #1
Aurelius120
- 152
- 16
- Homework Statement
- A 300kg crate is dropped vertically onto a conveyer belt moving at constant speed of 1.20m/s under the action of motor. If coefficient of kinetic friction is 0.400 find work done my the motor.
- Relevant Equations
- NA
The question was this:
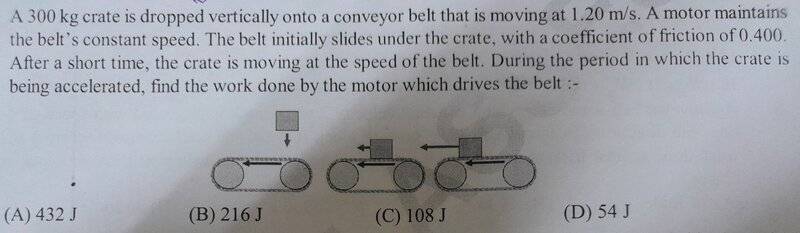
My calculations show that the answer should be equal to work done on crate to make it reach the same velocity which is equal to 216 J but the answer given is 432 J
It is believed that extra energy is needed to overcome friction but friction is an internal force and there is no mention of heat loss so work done should be independent of friction.
Also if extra energy was needed to overcome friction between belt and crate, it should also mean that the work done in pushing two blocks of metal placed on each other would be more than that of a single block made by melting both which is not true?
Please Explain
Thank You
My calculations show that the answer should be equal to work done on crate to make it reach the same velocity which is equal to 216 J but the answer given is 432 J
It is believed that extra energy is needed to overcome friction but friction is an internal force and there is no mention of heat loss so work done should be independent of friction.
Also if extra energy was needed to overcome friction between belt and crate, it should also mean that the work done in pushing two blocks of metal placed on each other would be more than that of a single block made by melting both which is not true?
Please Explain
Thank You