Relativistic submarine paradox
Note: I have edited this post to correct my mistaken assumption that that if a particle falls a given distance in gamma less time in a moving frame then it requires gamma squared less gravitational force for that to happen. Upon reflection, in relativity force is F' = (dp/dt)' = (dmdx/dt^2)' = (dm y)(dx)/(dt^2 y^2) = F y^{-1}. In other words gamma less force results in gamma squared less acceleration which gives the required gamma less falling interval.
=================================================================
Hi,
To try and shed more light on the subject I have referred to a paper by Matsas
http://arxiv.org/PS_cache/gr-qc/pdf/0305/0305106v1.pdf where he "solves the submarine paradox. Another simplified version of the paper is here.
http://arxiv.org/PS_cache/arxiv/pdf/0708/0708.2488v1.pdf
The analysis done by Matsas is relevant here because in his own words:
" ... we will look for a background with planar symmetry. This is necessary in order to avoid the appearance of centrifugal effects which are not part of the submarine paradox. This is accomplished by the Rindler spacetime."
After a lengthy calculation he concludes that that the forces acting on the submarine as measured by an observer co-moving with the submarine experiences a total force of:
F' = -mg \gamma (\gamma - 1/ \gamma)
where -mg \y^2 is the force accelerating the sub downwards and mg is the buoyancy force acting upwards on the sub. mg is also the magnitude of the forces that are in equilibrium when the submarine is stationary with respect to the seabed.
He then does a Lorentz transformation and concludes that the total forces acting on the moving submarine as measured by an observer stationary with water and the sea bed as:
F= -mg (\gamma - 1/ \gamma)
We see in this last equation that both the gravitational force acting on the moving submarine and the bouyancy force is greater by gamma compared to when it is not moving.
Now we adapt the experiment by removing the water and buoyancy forces.
Lets say it takes one second for the non moving sub to fall vertically to the seabed when released, as timed by an observer on the seabed. Now if the sub is moving horizontally at 0.8c the sub takes 1*(0.6) = 0.6 seconds to fall to the seabed as timed by that same observer.
In Earth strength gravity the correction required to allow for the difference in clock rates due to height is of the order of parts per billion. This is insignificant compared to the reduction of 40% in falling time interval due to the horizontal velocity. Clearly we should not get too hung up on whether the observer is free falling or not.
Now the time it takes to fall from the point of view of an observer co-moving with the submarine is the time measured by the seabed observer reduced by gamma. This requires the downward acceleration in the moving submarine frame to be greater by gamma than in the seabed frame.
Analysis of the buoyancy force
===============================================================
When an object with no horizontal motion falls the time period for the object to fall is increased by gamma as viewed by an observer moving horizontally relative to the object. This suggests the force of gravity is reduced by gamma squared in this situation.
We can apply this observation to the buoyancy calculation. One description of buoyancy is that the upward force is proportional to the weight of water displaced. When the sub is moving wrt the water it is length contracted so it displaces gamma less water so there is gamma less upward force from the pov of the observer on the seabed. (mg/y)
To an observer on the sub there is gamma more mass of water displaced due to length contraction of the water relative to the length of the submarine. To this observer the water is co-moving with sea bed so it experiences a gravitational force that is reduced by gamma. Theses two effects cancel out and the weight of the displaced water is simply mg. (in agreement with Matsas)
Matsas used a different method. He reasoned that when the sub is moving relative to the seabed observer, it is length contracted relative to the water so there are gamma less columns of water supporting the sub. To an observer on the sub there gamma more columns of water underneath the sub than when the sub was at rest with water. Each of these columns exert an upward force that is reduced by gamma due to the relative motion of the water so the net effect is that the upward buoyancy force is the same as when the sub was stationary wrt the water.
=================================================================
So along the way we have concluded:
When the massive body is moving relative to the observer (and the test particle is not) the effective force of gravity is F' = F y^2
When the particle is moving wrt the observer (and the massive body is not) then the effective force of gravity on the particle is F' = F y
When the test particle and the massive body are both moving with the same velocity relative to the observer then the effective force of gravity on the particle is F' = F / y
The last observation is a requirement of Special Relativity.
An equation that wraps all these apparently contradictory observations into one unified result is:
F' = \frac{GMm}{R^2} \frac{(1-Vv/c^2)^2}{(1-V^2/c^2)\sqrt{1-v^2/c^2}}
where V is the velocity of the massive body (M) wrt to the observer and v is the velocity of the test particle (m) with respect to the observer.
It is assumed here that M is much greater than m so that we do have to be concerned with acceleration of M towards m.
The principle involved to formulate that equation is that the force of gravity between M and m increases by gamma squared as a function of the relative velocity (V-v) of M and m to each other (using the relativistic subtraction formula) and at the same time, the force of gravity acting on m is reduced by a factor of gamma as a function of the velocity (v) of the test particle (m) relative to the observer.
The equation is a bit easier to visualise when expressed as
F' = \frac{GMm}{R^2} \frac{\sqrt{1-v^2}}{(1-(V-v)^2)}
where it is understood that (V-v) is the relativistic subtraction of v from V.
=============================================================
The problem I still have is that this experiment that is designed to be as close as possible to a gravitational field simulating an upwardly accelerating rocket shows there are significant differences, and the Equivalence Principle breaks down under even these supposedly ideal conditions.


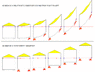
 ) Matsas concludes in his paper that a horizontally moving object will experience \sqrt{1-v^2/c^2} greater gravitational "force" than an object falling purely vertically. This presumably translates into a greater downward acceleration of (gamma squared) for the horizontally moving object.
) Matsas concludes in his paper that a horizontally moving object will experience \sqrt{1-v^2/c^2} greater gravitational "force" than an object falling purely vertically. This presumably translates into a greater downward acceleration of (gamma squared) for the horizontally moving object.