- 36,475
- 15,225
I was thinking about how one would teach special relativity using a spacetime-geometric approach. I think you would first show spacetime diagrams in a single frame and describe velocity as the slope of the worldline. Then when you moved onto other frames it would be natural to leave the slope of a light cone at a 45º angle.
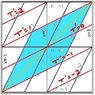
However, I believe that the natural tendency of students would be to try to preserve absolute simultaneity by drawing the spactime diagram as attached. Such a diagram would preserve c as a frame invariant, would show length contraction, no time dilation, and absolute simultaneity. How could you get to the Lorentz transform from here?
However, I believe that the natural tendency of students would be to try to preserve absolute simultaneity by drawing the spactime diagram as attached. Such a diagram would preserve c as a frame invariant, would show length contraction, no time dilation, and absolute simultaneity. How could you get to the Lorentz transform from here?