4-acceleration of a vector in a linearly moving train

[yuiop]

Consider the thought experiment in the diagram below.

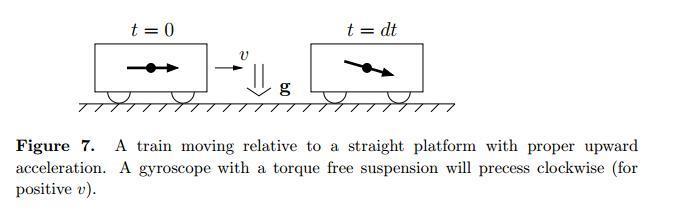
According to the authors, the vector will rotate even in the rest frame of the moving train relative to the body of the train. Is it possible to consider the spin axis of the gyroscope represented by the vector as a simple rod and analyse the situation as a simple 4-acceleration problem? If, so would the rod rotate by exactly the same amount in the rest frame of the train, irrespective of its original orientation? I suspect there is a critical orientation of the rod that would be exactly parallel to the boosted acceleration, such that no rotation of the rod occurs. If so, this would suggest that an initial assembly of rods that are orthogonal to each other would not remain orthogonal, because they rotate at different rates.

The above diagram comes from this paper http://www.relativitet.se/Webarticles/2006CQG-Jonsson23p37.pdf which is specifically about gyroscopes. Would we expect rods and gyroscopes to rotate differently is no torque is directly applied to them? One issue that is mentioned in the paper is that in certain reference frames, the proper centre of mass is no longer at the normal centre of mass of a perfectly balanced gyroscope. This might be a source of torque even when we think we are applying the acceleration to the centre of mass of the gyroscope in a torque free way.

 

[WannabeNewton]

God I love the threads you make :)

Anyways, read through the following article: http://arxiv.org/pdf/0708.2490v1.pdf

It's shorter than the one you linked but analyzes the same scenario using a much simpler mathematical formalism and explains why the train has a rotation relative to the gyroscope. Section V.A, with equation (15) in particular, should answer your questions. The effect of course is rather easy to understand. If we do as the article says and go to a momentary rest frame of the platform and then boost to the momentary rest frame of the train on the platform at this instant then in this frame the platform actually looks parabolic due to relativity of simultaneity-a very simple Lorentz transformation calculation verifies this explicitly. Since the platform+train system is stationary (no time dependence) this should obviously be the case no matter what momentary rest frame of the platform we choose and so we can conclude that in the rest frame of the train, the platform is a backwards moving parabolic shape. This will of course make the gyroscope precess relative to the train frame in a clockwise manner since the parabola is convex.

However I don't see the need to consider the spin axis of the gyroscope as a rod. Why not just keep it as a spin 4-vector space-like relative to the gyroscope as usual? Why represent it as a rod? The mathematical analysis only requires us to represent the gyroscope's spin axis as a spin vector.

 

[yuiop]

God I love the threads you make :)

Anyways, read through the following article: http://arxiv.org/pdf/0708.2490v1.pdf

Thanks ;) Not digested the full article yet, but I note it is by the same author.

However I don't see the need to consider the spin axis of the gyroscope as a rod. Why not just keep it as a spin 4-vector space-like relative to the gyroscope as usual? Why represent it as a rod? The mathematical analysis only requires us to represent the gyroscope's spin axis as a spin vector.

I note that in the other gyroscope threads the only real contributors are you and Peter. That is partly understandable as gyroscopes are inherently scary and mysterious things even before you introduce relativity, so I was hoping to get a response from the members that I know are experts with 4 acceleration and leave the gyroscope complication out. Hence the rods. I notice that the author of the article you linked also uses a grid of rigid rods to simplify his analysis or at least the visualisation.

 

[WannabeNewton]

That is partly understandable as gyroscopes are inherently scary and mysterious things even before you introduce relativity, so I was hoping to get a response from the members that I know are experts with 4 acceleration and leave the gyroscope complication out.

Well you certainly don't need the whole formalism of Fermi-transport and spin 4-vectors for this problem. The second paper for example tackles the problem very elegantly (especially from a physics standpoint) using elementary calculations so if what you're really after is an elementary analysis of the gyroscopic precession in the train frame then the second paper does a fine job of providing just that. But notice that a key ingredient of the calculations is the relationship between the differential change in angle and differential change in boost velocity; since the only 4-acceleration in this problem is that of the platform, you cannot use only 4-acceleration to analyze the problem as you will not be able to employ said key ingredient.

 

[PeterDonis]

I was hoping to get a response from the members that I know are experts with 4 acceleration and leave the gyroscope complication out. Hence the rods.

You can't leave the gyroscope out completely because a key assumption of the problem is that the "gyroscope" vector is Fermi-Walker transported. If you just make it a "rod", then what ensures, physically, that it is Fermi-Walker transported? There has to be *some* mechanism, physically, that does that; a gyroscope is just a convenient mechanism.

 

[WannabeNewton]

By the way just to answer your original questions:

If, so would the rod rotate by exactly the same amount in the rest frame of the train, irrespective of its original orientation?

The introduction of the rod really won't change the analysis at all so you may as well stick to talking about the spin axis of the gyroscope. Anyways, no the initial orientation would not affect the precession-think back to the picture of the platform in its curved parabolic shape moving backwards relative to the train rest frame mentioned in post #2.

I suspect there is a critical orientation of the rod that would be exactly parallel to the boosted acceleration, such that no rotation of the rod occurs.

The precession of the spin axis is given in terms of the cross product of the train velocity relative to the platform and the upwards platform acceleration. The only way to have the precession vanish is to consider the trivial case of the train having a velocity parallel to the platform acceleration. Apart from that, no orientation of the spin axis can prevent it from precessing in the train rest frame.

 

[pervect]

I'm not quite sure what the question is, but there isn't any difference between a force-free rod with no angular momentum and a gyroscope. However, not all rods are force-free, and not all rods have zero angular momentum. A force free gyroscope is less ambiguous.

 

[WannabeNewton]

Sorry not to derail the thread or anything but I was just wondering if someone could check something really quickly for me. I was just trying to see if one could derive the expression for the gyroscopic precession relative to the rest frame of the train by using vorticity. More precisely, if we replace the train moving at constant speed to the right relative to the upwards accelerating platform by a train moving to the right at constant speed in a uniform gravitational field $g$ then we could setup the problem in Rindler coordinates with metric $ds^{2} = -g^2 z^2 dt^2 + dx^2 + dy^2 + dz^2$.

The vector field $\partial_t$ is the tangent field to the family of observers at rest in the gravitational field so presumably $\xi = \partial_t + v \partial_x$ would be the tangent field to the family of observers moving to the right with a constant speed $v$ in this gravitational field. The squared norm of $\xi$ is $\langle \xi, \xi \rangle = -g^2 z^2 + v^2 < 0$ so this tangent field would only be defined in the region $z > \frac{v}{g}$. Furthermore, $\xi$ is obviously a Killing field.

Now $\xi^{\flat} = gz(-gzdt) + vdx = gz e^{t} + v e^{x}$ so the vorticity is $\omega = \star (\xi^{\flat}\wedge d\xi^{\flat}) = vg \partial_y$. The gyroscopic precession is given by $\Omega = -\frac{1}{2}\langle \xi, \xi \rangle^{-1}\omega = \frac{1}{2}\frac{vg}{g^2 z^2 - v^2}\partial_y$ the magnitude of which is $\Omega = \frac{1}{2}\frac{vg}{g^2 z^2 - v^2}$. The paper on the other hand has $\tilde{\Omega} = \gamma^2 vg = \frac{vg}{1 - v^2}$ (http://arxiv.org/pdf/0708.2490v1.pdf see p.6). Why the discrepancy? If I happen to choose the altitude of the train in the gravitational field so that $gz = 1$ then I get $\Omega = \frac{1}{2}\gamma^2 vg$ but that seems contrived not to mention still fails to yield the same result as in the paper. Thanks.

 

[PeterDonis]

The vector field \partial_t is the tangent field to the family of observers at rest in the gravitational field

No, it isn't; it's $\left( 1 / gz \right) \partial_t$. The coefficient is needed to make the norm come out to be $-1$.

presumably $\xi = \partial_t + v \partial_x$ would be the tangent field to the family of observers moving to the right with a constant speed $v$ in this gravitational field.

No, it would be $\xi = \left( \gamma / gz \right) \partial_t + \gamma v \partial_x$, where $\gamma = 1 / \sqrt{1 - v^2}$. Again, the coefficients must be that way to make the norm come out to be $-1$.

Furthermore, $\xi$ is obviously a Killing field.

Not if $\xi$ is the 4-velocity of observers at rest in the "gravitational field", as above. The vector field $\xi = \partial_t + v \partial_x$ that you originally wrote down *is* a KVF, but its norm is not always $-1$; like the "static" KVF in any "gravitational field", its norm gives the "redshift factor" along its integral curves. What is true is that this KVF has the same integral curves as the 4-velocity field given above, so observers at rest in the "gravitational field" follow integral curves of the KVF--but with tangent vectors whose magnitudes are adjusted, relative to the norm of the KVF, so that they are always unit timelike vectors.

 

[WannabeNewton]

No, it isn't; it's $\left( 1 / gz \right) \partial_t$. The coefficient is needed to make the norm come out to be $-1$.

The tangent field doesn't need to be normalized. In fact the formula relating vorticity to gyroscopic precession employed above as well as in the previous threads explicitly demands the use of the (non-normalized) Killing field parallel to the 4-velocity field and not the 4-velocity field itself (which in general is not a Killing field). Both the Killing field and the 4-velocity field are tangent fields but it is only the former that we need when computing gyroscopic precession.

 

[PeterDonis]

The tangent field doesn't need to be normalized.

But if it isn't normalized, you won't be computing its derivatives properly, so you won't be computing the kinematic decomposition properly.

Take a simple example: the proper acceleration of the Rindler congruence. If we use the 4-velocity field (the one I wrote down), we have $u^a \nabla_a u^b = u^t \left( \partial_t u^t \partial_t + \Gamma^z{}_{tt} u^t \partial_z \right) = \left( 1 / gz \right) g^2 z \left( 1 / gz \right) \partial_z = \left( 1 / z \right) \partial_z$, which is correct.

However, if we use the Killing field (the one you wrote down), we have $u^a \nabla_a \xi^b = u^t \left( \partial_t \xi^t \partial_t + \Gamma^z{}_{tt} \xi^t \partial_z \right) = \left( 1 / gz \right) g^2 z \partial_z = g \partial_z$, which is wrong--it says the proper acceleration is the same for every worldline in the Rindler congruence.

In fact the formula relating vorticity to gyroscopic precession employed above as well as in the previous threads explicitly demands the use of the (non-normalized) Killing field parallel to the 4-velocity field and not the 4-velocity field itself (which in general is not a Killing field).

Can you give specific references? It's always been my understanding that the vorticity of a timelike congruence is computed using the 4-velocity field of the congruence, just like all the other elements of the kinematic decomposition. Otherwise you get wrong answers, as above.

 

[pervect]

I'm not quite sure what the question is, but there isn't any difference between a force-free rod with no angular momentum and a gyroscope. However, not all rods are force-free, and not all rods have zero angular momentum. A force free gyroscope is less ambiguous.

To expand on this a bit, if the train is rotating, and the gyroscope isn't, something is exerting a torque on the train to make it rotate. If the train was accelerated in a torque free manner through it's center of mass without touching the floor, it would rotate relative to the floor just like the gyroscope does.

 

[WannabeNewton]

Can you give specific references? It's always been my understanding that the vorticity of a timelike congruence is computed using the 4-velocity field of the congruence, just like all the other elements of the kinematic decomposition. Otherwise you get wrong answers, as above.

See, for example, expression (2.161) in Straumann. We don't actually need to compute the vorticity of the 4-velocity field itself. It suffices to compute the vorticity of the Killing field. This combined with the norm of the Killing field determines the gyroscopic precession as evidenced by expression (2.161). The factor of $\frac{1}{2}$ that I'm off by shouldn't be a problem as that's probably just the result of the usual difference in convention when defining vorticity. I'm just not sure if I'm allowed to set $gz = 1$ if all I care about is the observer (or train or whatever) accelerating with magnitude $g$ due to the gravitational field (or upwards accelerating platform) along with the constant velocity $v$ to the right.

EDIT: In other words, it doesn't matter if we use the 4-velocity field $u^{\mu} = (-\langle \xi, \xi \rangle)^{-1/2} \xi^{\mu}$ or the time-like Killing field $\xi^{\mu}$ itself so as long as we employ the correct formulas. We can write the gyroscopic precession directly in terms of the vorticity of $u^{\mu}$ as $$\Omega^{\mu} = \frac{1}{2}\epsilon^{\mu\nu\alpha\beta}u_{\nu}\partial_{\alpha}u_{\beta} \\= -\frac{1}{2}\langle \xi,\xi \rangle^{-1}\epsilon^{\mu\nu\alpha\beta}\xi_{\nu}\partial_{\alpha}\xi_{\beta} - \frac{1}{2}(-\langle \xi, \xi \rangle)^{-1/2}\epsilon^{\mu[\nu\beta]\alpha}\xi_{[\nu}\xi_{\beta]}\partial_{\alpha}(-\langle \xi,\xi \rangle)^{-1/2} \\= -\frac{1}{2}\langle \xi, \xi \rangle^{-1}\epsilon^{\mu\nu\alpha\beta}\xi_{\nu}\partial_{\alpha}\xi_{\beta}$$ so we get back the formula used in post #8 regardless.

I mainly just want to know if setting $z = \frac{1}{g}$ in the expression $\Omega = \frac{1}{2}\frac{vg}{g^2 z^2 - v^2}$ is actually warranted given that the observer (train) is subject to an acceleration of $g$ from the gravitational field (accelerating platform) and so would presumably be at a constant altitude $z = \frac{1}{g}$ in the gravitational field in Rindler coordinates whilst drifting to the right at a constant velocity $v$.