Current theories on the lepton masses?

[jmheer]

Hi, all!
I'm currently studying the 3 families of leptons.
1) Are there any accepted theories about why the lepton masses are what they are?
2) What are the best journals to read about this kind of thing?

I'm aware of the Koide formula, but that seems to be an empirical formula, not a theory.

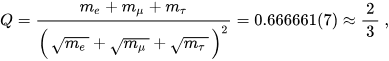
Thanks!
-Joe Heer

 

[mfb]

They are free parameters in the Standard Model. For all we know they can have arbitrary values. The Koide formula is interesting but we don't know if it is exact, and if it is then we don't know why.

2) What are the best journals to read about this kind of thing?

In practice everyone uses arXiv, but you can also go to the usual particle physics journals (check where arXiv submissions get published):
https://arxiv.org/list/hep-ex/new
https://arxiv.org/list/hep-ph/new
https://arxiv.org/list/hep-th/new

 

[jmheer]

So if I understand what you are saying, there are currently no accepted theories and the lepton masses are considered to be arbitrary free parameters, for practical purposes. That was my understanding - thank you for confirming it, and sharing the arXiv links.
-Joe

 

[Vanadium 50]

Before going to far down this path, an important fact is that masses have dimensions (like MeV), so you can only do this with respect to some other mass, like the W mass. (Or the Higgs vev, which is the same thing) So you aren't calculating a mass so much as a ratio.

 

[Orodruin]

The Koide formula is interesting

Also known as numerology. And not in a flattering sense …

 

[Vanadium 50]

Be careful - the denizens of the BTSM sub-forum love love love their Koide.

To me, given two numbers and an infinite number of possible equations it's not surprising one can "predict" a third.

 

[Orodruin]

To me, given two numbers and an infinite number of possible equations it's not surprising one can "predict" a third.

Yes, I have seen way too much of this in neutrino mixing. A thousand different symmetries with a million different ways to break them. ”We just need to measure the mixing angles precisely enough to know which of the symmetries is realized in Nature”
… even though the possible symmetry predictions seem to form a dense subset of the possible mixing values.

 

[fresh_42]

Be careful - the denizens of the BTSM sub-forum love love love their Koide.

Well, the last time a numerologist had accidentally been right was 2,550 years ago (Pythagoras). Maybe Koide is lucky.

 

[jmheer]

There are cases where empirical equations like the Balmer series were predictive and helped motivate later theoretical developments. I view the Koide formula as interesting, but its main value will come only if it motivates theoretical developments that help explain why the ratio is the way it is.

 

[Vanadium 50]

I have seen way too much of this in neutrino mixing.

Youngsters.

Before that, it was the CKM matrix. (Look up "textures")
Before that, it was why $\sin^2(\theta_W)$ was exactly 1/4. (For the non-experts, it's not)
Before that, it was why the fine structure constant was exactly 1/137. (For the non-experts, it's not)

Why is it always numerology? Why not phrenology Or the reading of entrails of animals?

 

[jmheer]

Before going to far down this path, an important fact is that masses have dimensions (like MeV), so you can only do this with respect to some other mass, like the W mass. (Or the Higgs vev, which is the same thing) So you aren't calculating a mass so much as a ratio.

Obviously the dimensions have to work out. So it is either a ratio with respect another mass, or some formula where the units work out in a way that gives mass.

 

[Orodruin]

Youngsters.

Before that, it was the CKM matrix. (Look up "textures")
Before that, it was why $\sin^2(\theta_W)$ was exactly 1/4. (For the non-experts, it's not)
Before that, it was why the fine structure constant was exactly 1/137. (For the non-experts, it's not)

Why is it always numerology? Why not phrenology Or the reading of entrails of animals?

At least in neutrinos we have tribimaximal mixing … plus corrections
(We do not)

 

[fresh_42]

Why is it always numerology? Why not phrenology Or the reading of entrails of animals?

Because Pythagoras had a hit. Not that he found something new or previously unknown, but the formula carries his name anyway. And Pythagoras was nothing else but a numerologist. 2550 years ago. Time to land another lucky punch?

 

[Vanadium 50]

the Balmer series were predictive and helped motivate later theoretical developments

Well, let's see. Rydberg was 1888. Rutherford was 1911. Twenty-three years.
Koide was 1981. It's now 42 years later. Coming on a factor of 2. (Interestingly, if you inscribe and circumscribe circles at the base of the Great Pyramid, their ratio is also 2. Coincidence? I think not!)

Let's write down the genera; Koide formula:

$$\frac{(m_1^a+m_2^a+m_3^a)^b}{(m_1^c+m_2^c+m_3^c)^d} = \frac{e}{f}$$

Where a = 1, b = 1, c = 1/2, d = 2, e = 2 and f = 3. So with my choice of equations and eight numbers, I can "predict" the ninth. This is why people are unimpressed.

 

[Vanadium 50]

Further, here's what the Koide rtatio looks like vs. Tau lepton mass. It's close to 2/3? How shocking!

 

[topsquark]

Well, the last time a numerologist had accidentally been right was 2,550 years ago (Pythagoras). Maybe Koide is lucky.

Don't forgot about Newton: he was an Alchemist!

-Dan

 

[fresh_42]

Don't forgot about Newton: he was an Alchemist!

-Dan

And royal astrologist!

 

[Vanadium 50]

And royal astrologist!

And a royal pain in the....nevermind.

 

[PeterDonis]

42

We already know that's the answer, now we just need to find out the question...

 

[topsquark]

We already know that's the answer, now we just need to find out the question...

Clearly, we need to grow a beard, tie a bone in it, and run around on a pre-historic supercomputer, masquerading as a planet, chasing a Chesterfield.

It will all make sense after that.

-Dan

 

[mitchell porter]

So if I understand what you are saying, there are currently no accepted theories and the lepton masses are considered to be arbitrary free parameters, for practical purposes. That was my understanding - thank you for confirming it, and sharing the arXiv links.
-Joe

The masses come from coupling to the Higgs field ("yukawa couplings"). These are the quantities which, in the standard model, are free parameters, i.e. their values are to be determined by experiment.

It is easy to formulate extensions to the standard model in which these couplings are constrained in some way. For example, one can postulate symmetries which force the yukawa couplings to have specific relations to each other.

In terms of the development of theory, the constraints on the masses which arise most naturally, are those which stem from "grand unification" of the forces. Positing that gluons and photons and weak bosons are all aspects of a unified force, also implies that e.g. certain leptons and quarks are aspects of the same ensemble of matter particles, which in turn implies that their yukawas aren't independent.

The simplest forms of grand unification are all but falsified, but there are hundreds of papers proposing variations on grand unification, plus various "flavor symmetries" affecting the yukawas; and these are field theories which naturally arise in string theory. In the context of string theory, the couplings are not merely constrained, but their exact values should follow from the shape of the extra dimensions, and so forth (though in practice, such calculations are still mostly beyond reach).

So there is a huge theoretical edifice in terms of which predictions are possible, and there are innumerable papers which fit into that edifice, and which make different predictions. The main problem is that such unified theories always predict other phenomena (this is how the simplest versions of grand unification were falsified), and none of those other predictions have been confirmed, though one may always hope that the latest bump at the colliders is a new particle showing up, or that the extra particles in one's unified theory can explain dark matter, inflation, or the cosmic excess of matter over antimatter...

But be aware: the mass predictions which come from this paradigm are typically like "the top quark is much heavier than everything else", or "electrons and up quarks are light". They are very qualitative predictions. For the most part, numerically exact predictions await the utopia in which string theory learns how to calculate particle masses; and most algebraic formulas that have been found for particle masses, lack an actual theory.

Out of such "numerological" formulas, the Koide formula maximizes the combination of elegance, intricacy, and attention from actual physicists. But, while there really is some attention to the formula from actual physicists, it's still a very marginal topic, because it is a relationship between "pole masses", whereas theory suggests that relationships should arise among "running masses" (pole mass and running mass being different technical definitions of mass, in quantum field theory). Also, the formula itself has an unusual form, compared to the mass relationships that normally appear in unified theories.

Nonetheless, a little bit of work has been done on how to obtain it, notably by Yoshio Koide himself, and I am definitely one of the Koide fans from the "Beyond the Standard Models" subforum.

 

[mfb]

Further, here's what the Koide rtatio looks like vs. Tau lepton mass. It's close to 2/3? How shocking!

It's only that close to 2/3 for 0.25 MeV in the 2300 MeV range you plotted.

The relation was proposed when the best tau mass estimate had an uncertainty of +3-4 MeV. That has shrunk to 0.12 MeV and the ratio is still 2/3 within the uncertainties. That's using the PDG average, this recent Belle II measurement will shrink uncertainties further and move the best fit ratio even closer to 2/3.

I don't say it has to be true, but if not then it is an exceptionally well-working coincidence.

 

[jmheer]

The masses come from coupling to the Higgs field ("yukawa couplings"). These are the quantities which, in the standard model, are free parameters, i.e. their values are to be determined by experiment.

It is easy to formulate extensions to the standard model in which these couplings are constrained in some way. For example, one can postulate symmetries which force the yukawa couplings to have specific relations to each other.

In terms of the development of theory, the constraints on the masses which arise most naturally, are those which stem from "grand unification" of the forces. Positing that gluons and photons and weak bosons are all aspects of a unified force, also implies that e.g. certain leptons and quarks are aspects of the same ensemble of matter particles, which in turn implies that their yukawas aren't independent.

The simplest forms of grand unification are all but falsified, but there are hundreds of papers proposing variations on grand unification, plus various "flavor symmetries" affecting the yukawas; and these are field theories which naturally arise in string theory. In the context of string theory, the couplings are not merely constrained, but their exact values should follow from the shape of the extra dimensions, and so forth (though in practice, such calculations are still mostly beyond reach).

So there is a huge theoretical edifice in terms of which predictions are possible, and there are innumerable papers which fit into that edifice, and which make different predictions. The main problem is that such unified theories always predict other phenomena (this is how the simplest versions of grand unification were falsified), and none of those other predictions have been confirmed, though one may always hope that the latest bump at the colliders is a new particle showing up, or that the extra particles in one's unified theory can explain dark matter, inflation, or the cosmic excess of matter over antimatter...

But be aware: the mass predictions which come from this paradigm are typically like "the top quark is much heavier than everything else", or "electrons and up quarks are light". They are very qualitative predictions. For the most part, numerically exact predictions await the utopia in which string theory learns how to calculate particle masses; and most algebraic formulas that have been found for particle masses, lack an actual theory.

Out of such "numerological" formulas, the Koide formula maximizes the combination of elegance, intricacy, and attention from actual physicists. But, while there really is some attention to the formula from actual physicists, it's still a very marginal topic, because it is a relationship between "pole masses", whereas theory suggests that relationships should arise among "running masses" (pole mass and running mass being different technical definitions of mass, in quantum field theory). Also, the formula itself has an unusual form, compared to the mass relationships that normally appear in unified theories.

Nonetheless, a little bit of work has been done on how to obtain it, notably by Yoshio Koide himself, and I am definitely one of the Koide fans from the "Beyond the Standard Models" subforum.

Thanks for the very detailed reply, Mitchell. It was quite helpful!

 

[fresh_42]

You can find dozens of formulas around the Lie groups of type $A_1$ and $A_2.$

Example: If $A$ is the Cartan matrix of $A_2$ then $2=\dim A, 3=\det A, 2\cdot 2=\operatorname{trace}(A)$ and $\{1,3\}$ are the eigenvalues.

This isn't evidence pro or contra Koide. However, if we write the formula with $E$ for energy instead of $m$ for mass to make it look less strange, then why shouldn't there be a relation built from all those elementary constants around the Lie groups we use to describe the electroweak field? Sure, a theory with a "Eureka $2/3$!" at the end is more satisfying than the other way around. But anything involving so small integers cannot be ruled out. It could easily be a coincidence of values, e.g.
$$
\dfrac{2}{3}=\dfrac{l}{n}=\dfrac{l}{l+1}=\dfrac{\dim CSA(\mathfrak{sl}(3))}{\dim \mathbb{C}^3}=\dfrac{\dim \mathbb{C}^2}{\dim \mathfrak{su}(2)}
$$
And this is the real problem. There are dozens of ways to end up with $2/3$ when all fundamental constants in such a case are taken from $\{\pm 1,\pm 2,\pm 3,\pm 4\}.$ That makes the formula useless because it does not provide hints, not even a glimpse of what might be behind it. Anything could be true, which means nothing is helpful. It hinders insights more than it points to them.

So if there is a relation between $\|(x,y,z)\|$ and $x+y+z$ then it could well be $2/3.$ The opposite of that statement, however, remains numerology.

The Greek Pythagoras only considered $(3,4,5)$ or $(5,12,13).$ etc. The triangle $(1,1,\sqrt{2})$ was irrational, not existent to him. $(3,4,5)$ is numerology, $(a,b,c)$ is the theory regardless of whether $a,b,c$ are rational or not. $(3,4,5)$ is thus only an example.

 

[mitchell porter]

Mathematically, the formula can only take values between 1/3 and 1. 2/3 is the exact midpoint. So while I would not want to completely discourage such group-theoretic experimentation, the evidence suggests that it is the algebraic formula, and not the specific value, that is the biggest clue.

It is also unusual for the square root of a mass to be part of a sum rule or other mass formula.

 

[ohwilleke]

Before going to far down this path, an important fact is that masses have dimensions (like MeV), so you can only do this with respect to some other mass, like the W mass. (Or the Higgs vev, which is the same thing) So you aren't calculating a mass so much as a ratio.

In the narrow sense Standard Model, the charged leptons are themselves massless prior to their interaction with the Higgs field, and the more fundamental numbers are the Yukawa couplings of the charged leptons to the Higgs field, rather than lepton masses per se. The Higgs vev of 246.22 GeV more or less is the conversion faction from Yukawas to fundamental particle masses which we observe.

Another bit of numerology, somewhat more recent than Koide's rule but (barely) within two sigma of the experimental data, is that the sum of the Yukawas for all of the Standard Model fundamental particles add up to 1 (with most of the uncertainty over the truth of this statement due to imprecision in the top quark pole mass measurement).

The number 1 could also be described as 100% and in this case, the Yukawas are basically allocating differing percentages of the Higgs vev to different fundamental particles.

 

[mfb]

There are dozens of ways to end up with $2/3$ when all fundamental constants in such a case are taken from $\{\pm 1,\pm 2,\pm 3,\pm 4\}.$

If fundamental constants are determined by small integers - which would be a revolutionary insight far more interesting than the Koide formula alone.

That's the point here. It is easy to get 2/3 if your numbers are connected in some way. If they are unrelated constants, as they are in the SM, then there is no reason for the relation to be 2/3.