Can Bloch Waves Reveal Periodic Potentials in Quantum Mechanics?

[hilbert2]

TL;DR Summary

Finding a 1d quantum system with potential energy V(x) producing a closed form wave function.

I was thinking about a problem I had considered a long time ago in some thread, finding an example of a wave function $\displaystyle \psi (x) =e^{iax}\phi (x)$ with $\displaystyle\phi (x)$ being periodic with period $\displaystyle L$ and the corresponding Schrödinger equation

$\displaystyle -\frac{\hbar^2}{2m}\frac{d^2 \psi (x)}{dx^2} + V(x)\psi (x) = E\psi (x)$,

such that the

$\displaystyle V(x) = E+\frac{\hbar^2}{2m}\frac{\psi'' (x)}{\psi (x)}$

is a periodic potential with period $L$, not singular anywhere and has a closed form expression.

Now, I was able to guess a wave function $\displaystyle \psi (x) = \sin (x) - \frac{1}{4}\sin (2x) + \frac{1}{16}\sin (3x)$, for which the potential is

$\displaystyle V(x) = -\frac{\hbar^2}{2m}\frac{\sin(x) - \sin (2x) + \frac{9}{16}\sin (3x)}{\sin (x) - \frac{1}{4}\sin (2x) + \frac{1}{16}\sin (3x)}$

(when the energy eigenvalue is arbitrarily set to $E=0$)

and for which the graphs of $\displaystyle\psi (x)$ (red line) and $\displaystyle V(x)$ (blue line) are like in the image (where it is set that $\hbar^2 /2m = 1$)

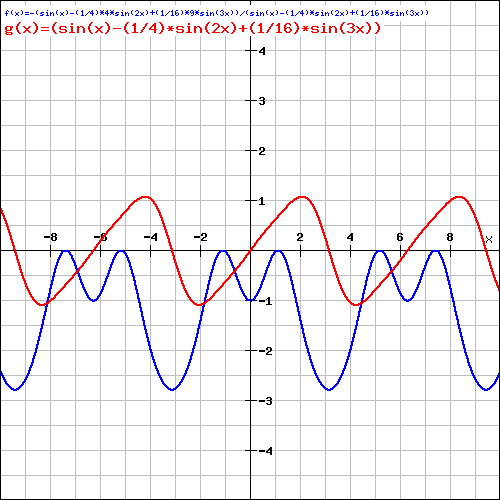
The plane wave part of the Bloch wave function, $\displaystyle e^{iax}$, is just a constant $1$ in this case, which means that $a=0$.

Had I chosen $\displaystyle \psi (x) = \sin (x) + \sin (2x) + \sin (3x)$, the potential $V(x)$ would have singular points and not look like a physically possible one.

So, anyone have clues on how it could be seen from the coefficients $C_k$ of $\displaystyle\psi (x) = \sum\limits_{k=1}^{\infty}C_k \sin (k x)$ whether the potential energy producing that eigenstate is also a 'nice' function?

 

[Haborix]

The only thing that really jumps to mind is trying to figure out a relation between $\psi$ and $\psi''$ such that their zeroes are coincident. The singularities must come from $\psi$ passing through zero. Also, for the taylor series around a zero of $\psi''$ the lowest order term can't have a power of $x$ less than that of the $\psi$. Maybe you can play around with the series and some of these facts to get a relation on the $C_k$?

 

[hilbert2]

Yes, the position of zeroes also came to my mind. If the series contains terms like $\cos kx$ instead of $\sin kx$, it's more difficult to make them coincide because a zero of $\cos (x)$ is not necessarily a zero of $\cos (kx)$ with $k\in\mathbb{N}$.

I couldn't find a nonzero value of $a\in\mathbb{R}$ such that the potential energy corresponding to the function $\psi (x) = e^{iax}\left(\sin (x) - \frac{1}{4}\sin (2x) + \frac{1}{16}\sin (3x)\right)$ would be a real valued function, so this probably only happens at individual values of $a$. The idea of the Bloch theorem is that a periodic $V(x)$ should produce a wave function that is periodic except possibly for the phase, as it is in the case of that $\psi (x)$ with period $L=\pi$. Maybe I should plot functions $\psi'' /\psi$ for many different values of $a$ and then see where the imaginary part stays small.

 

[DrClaude]

I'm not addressing your post, but it jumped at me that

$\displaystyle V(x) = -\frac{\hbar^2}{2m}\frac{\sin(x) - \sin (2x) + \frac{9}{16}\sin (3x)}{\sin (x) - \frac{1}{4}\sin (2x) + \frac{1}{16}\sin (3x)}$

has the wrong dimensions.

 

[hilbert2]

Yeah, the multipliers of $x$ in the $\sin (kx)$ terms should have dimensions of reciprocal length, but the system will be made dimensionless anyway by setting $\hbar^2 /2m = 1$ and this calculation is just to see what form of the function $\psi$ produces an acceptable function $V$.

 

[DrClaude]

Yeah, the multipliers of $x$ in the $\sin (kx)$ terms should have dimensions of reciprocal length, but the system will be made dimensionless anyway by setting $\hbar^2 /2m = 1$ and this calculation is just to see what form of the function $\psi$ produces an acceptable function $V$.

But it should work with dimensions. You can have a normalization constant with dimensions of $L^{-1/2}$ to take care of the dimensions of $\psi$, but $\psi''$ will not have the right dimensions. It makes me think that the $\psi$ you used is not a proper wave function.

 

[hilbert2]

If the $\psi$ has terms like $\sin (kx)$ and the $k$ is of dimensions $1/L$, then the 2nd derivative gets multipliers like $1/L^2$ in front of the terms. I'd usually handle something like this by choosing atomic units by setting $\hbar = m = 1$ and then taking for granted that the $V(x)$ is in units of hartree and $x$ in units of bohr. A more complicated way would be to form a dimensionless length variable $s$ of form $s = \hbar^\alpha m^\beta e^\gamma \epsilon_{0}^{\delta} x$ with $\alpha ,\beta ,\gamma ,\delta$ some rational numbers and a similar dimensionless energy variable. The $e$ and $\epsilon_{0}$ there are the elementary charge and vacuum permittivity. Then the $\psi (x)$ could be written in terms of $s$.

 

[Dr_Nate]

The only thing that really jumps to mind is trying to figure out a relation between $\psi$ and $\psi''$ such that their zeroes are coincident.

This must be it. We all know that we can't divide by zero.

Looking at your graph of $V(x)$, your choice of prefactors gives you -1 at $x=0$. But inputting 0 into $V(x)$ gives you $-0/0$. I applied L'Hopitals rule to $$V(x)=-\frac{C_1\sin{x}+4C_2\sin{2x}+9C_3\sin{3x}}{C_1\sin{x}+C_2\sin{2x}+C_3\sin{3x}}$$as $x\rightarrow 0$ and set it equal to -1. $C_1$ subtracts out and leaves $C_2=-4C_3$. That is consistent with your prefactors. Looking at your graph for the next value and repeating that same method with $$\lim_{x \rightarrow \pi} V(x) = -11/4$$ gives me a value for $C_1$ when I choose $C_3=\frac{1}{16}$. However, it does not reproduce your graph and, in fact, gives me poles when plotted.

It's late here. You should probably try the calculation yourself to see if I made a mistake.

 

[hilbert2]

Looking at your graph for the next value and repeating that same method with $$\lim_{x \rightarrow \pi} V(x) = -11/4$$ gives me a value for $C_1$ when I choose $C_3=\frac{1}{16}$. However, it does not reproduce your graph and, in fact, gives me poles when plotted.

If I calculate the $\lim\limits_{x\rightarrow \pi}V(x)$ with L'Hopital, it gets the value

$\displaystyle\lim\limits_{x\rightarrow \pi}\left(-\frac{\sin x - \sin 2x + \frac{9}{16}\sin 3x}{\sin x - \frac{1}{4}\sin 2x + \frac{1}{16}\sin 3x}\right)\\\displaystyle =\lim\limits_{x\rightarrow \pi}\left(-\frac{\cos x - 2\cos 2x + \frac{27}{16}\cos 3x}{\cos x - \frac{1}{2}\cos 2x + \frac{3}{16}\cos 3x}\right)\\\displaystyle =-\frac{\cos \pi .-2\cos 2\pi + \frac{27}{16}\cos 3\pi}{\cos \pi - \frac{1}{2}\cos 2\pi + \frac{3}{16}\cos 3\pi}\\\displaystyle =-\frac{1+2+\frac{27}{16}}{1+\frac{1}{2}+\frac{3}{16}}\\\displaystyle =-\frac{25}{9} \approx -2.78$.

This is clearly the same value as in the graph.

 

[Dr_Nate]

I now get $C_1 = 16 C_3, C_2=-4C_3$ given your values at $x=0$ and $\pi$. This seems like a method that works.

 

[hilbert2]

Another wavefunction I thought of is an approximation of the cosine function made from alternating downward and upward opening parabolas:

$\displaystyle\psi (x) = \sum\limits_{n\in 2\mathbb{Z}}(-1)^{n/2}(1-(x-n)^2 )\theta (1-|x-n|)$

where $\theta (x)$ is the Heaviside theta function and the graph of which is like

Then, because for $\displaystyle\psi (x) = 1-(x-n)^2$

$\displaystyle\frac{\psi '' (x)}{\psi (x)} \propto \frac{1}{1-(x-n)^2}$

the potential energy $V(x)$ is like a 1D "lattice" of inverse square potential energies

at least if the "lattice spacing" is made large enough for the $V(x)$ to be very close to zero at the midpoint between two singularities.

Didn't immediately find any applications of this lattice model with a Google search, but that piecewise defined parabola function was a quite curious object.