Find the surface area of the given solid

[chwala]

Homework Statement

See attached

Relevant Equations

Parametric equations

My question is on how did they determine the limits of integration i.e $2$ and $3$ as highlighted? Thanks

 

[Frabjous]

The problem statement is incomplete.

 

[chwala]

Find the link here;

http://ltcconline.net/greenl/course... Derivative of Parametric,x with respect to t.

 

[Frabjous]

I meant that the problem statement did not include the limits, i.e., poorly written.

 

[Mark44]

I meant that the problem statement did not include the limits, i.e., poorly written.

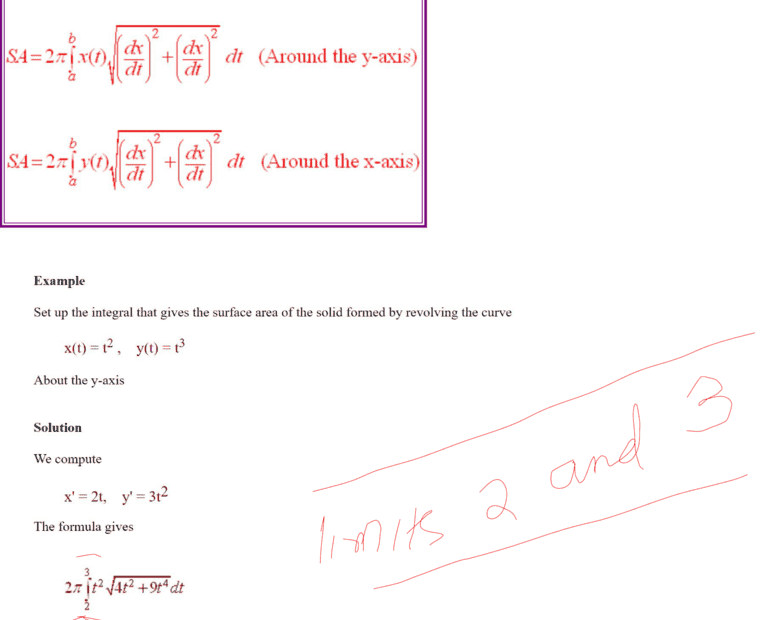
I agree. The graph of the parametric curve $x = t^2, y = t^3$ lies in Quadrants I and IV, and is unbounded. There has to be additional but unstated constraints for the limits of integration that are shown.

 

[WWGD]

@chwala : It seems in your square root, you're using $\frac {dx}{dt}$twice, rather than what I believe is correct, $\frac {dx}{dt}, \frac{dy}{dt}$

 

[chwala]

@chwala : It seems in your square root, you're using $\frac {dx}{dt}$twice, rather than what I believe is correct, $\frac {dx}{dt}, \frac{dy}{dt}$

@WWGD This is not my working rather notes that i came across as indicated by the given internet link;

yes, there is a mistake there... it ought to be

$$Surface area (y-axis) = 2π \int_ a^b x(t)\sqrt{(x^{'})^2+(y{'})^2}$$

where

$$x^{'}=\dfrac{dx}{dt}$$

$$y^{'}=\dfrac{dy}{dt}$$

 

[Mark44]

Since there seems to be two errors on that page, the missing information about boundaries, and the formula for surface area, perhaps you should look elsewhere for information on how to calculate surface area.