Fuel paradox arising from Galilean transformation?

[SeniorGara]

TL;DR Summary

Does the fuel paradox arise when the same engine operates with different power in different frames of reference?

I have encountered a problem related to the Galilean Transformation. Let's consider two observers who will be referred to as $O$ and $O^{'}$, with their corresponding coordinates $(t,x,y,z)$ and $(t^{′},x^{′},y^{′},z^{'})$ respectively. They are initially at the same location, at time zero. Furthermore, observer $O^{'}$ moves away from observer $O$ as shown in the picture.

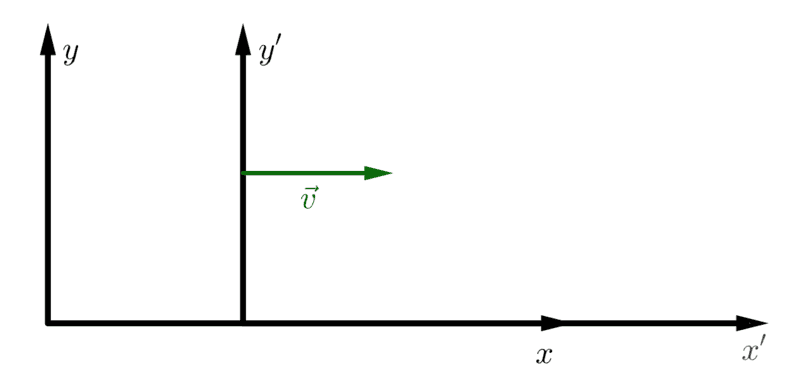
Any point $P$ that does not move in relation to $O$ will be described by $O^{'}$ with the following equations: $$x_{P}'=x_{P}-vt,\quad{y'_{P}=y_{P},}\quad{z'_{P}=z_{P},}\quad{t'=t}.$$ If an object (for example a car) moves in relation to $O$ according to the equation $$x(t)=vt+\frac{1}{2}at^2,$$ $O'$ will describe this movement in the following way: $$x'(t)=x(t)-vt=\frac{1}{2}at^2.$$ Assuming that no resistance force is present, the resultant force is equal to the force of engine thrust. Of course, $F=F'=ma$ because $\ddot{x}(t)=\ddot{x'}(t)=a$. Observer $O$ claims that the work done by the force of engine thrust is equal to $$W(t)=F\cdot{x(t)}=ma\left(vt+\frac{1}{2}at^2\right)=mavt+\frac{1}{2}ma^2t^2\mathrm{,}$$ whereas $O'$ observes that the engine has performed work equal to $$W'(t)=F'\cdot{x'(t)}=ma\left(\frac{1}{2}at^2\right)=\frac{1}{2}ma^2t^2.$$ Thus, $O$ concludes that the engine is operating at power $$P(t)=\frac{dW}{dt}(t)=mav+ma^2t,$$ while $O'$ considers that the engine power is equal to $$P'(t)=\frac{dW'}{dt}(t)=ma^2t.$$ Here is my question: If the same engine works with different power in two frames of reference, wouldn't it lead to the "fuel paradox"? In other words, according to $O$, the fuel will be depleted faster than according to $O'$. Of course, it can't be true. So, where is the mistake?

 

[jbriggs444]

In other words, according to $O$, the fuel will be depleted faster than according to $O'$. Of course, it can't be true. So, where is the mistake?

What about the work being done on the exhaust stream? [Always the answer in this flavor of paradox]

 

[Ibix]

Notice that momentum is not conserved in your example, so you don't have a closed system and energy is slipping out unaccounted for. Account for the reactive acceleration of the Earth and you will find your missing energy.

Edit: scooped by mere seconds!

 

[PeroK]

You don't need anything as elaborate as your calculations. If a $2 \ kg$ object increases its velocity from $0$ to $1 \ m/s$ in one frame, then it gains $1J$ of energy. But, in a frame where it changes from $1$ to $2 \ m/s$ it gains $3J$ of energy. This gives the same potential paradox when considerihg the energy supply.

 

[jbriggs444]

You don't need anything as elaborate as your calculations. If a $2 \ kg$ object increases its velocity from $0$ to $1 \ m/s$ in one frame, then it gains $1J$ of energy. But, in a frame where it changes from $1$ to $2 \ m/s$ it gains $3J$ of energy. This gives the same potential paradox when considerihg the energy supply.

To carry this scenario through, let us consider that this $2 \text{ kg}$ object gets its velocity increment by pushing off at $1 \text{ m/s}$ from an equally massive object. Our object moves off to the right at $+1 \text{ m/s}$ and the other object moves off to the left at $-1 \text{ m/s}$.

In the original rest frame of the two objects, that is $2J$ of total energy increment, $1J$ for each.

In a frame where the two objects start at $1 \text{m/s}$, that is $-1J$ for the left hand object and $+3J$ for the right hand object. The total is $2J$. Same as before.

The change in mechanical energy is invariant under a Galilean transformation to a new inertial frame. Also under a Lorentz transform as it turns out, though the formula for mechanical energy needs to be corrected for that to work.