- #1
applestrudle
- 64
- 0
Case 2:
I get that D = c I means A must also be proportional to I but how does that mean B must be diagonal?
Question:
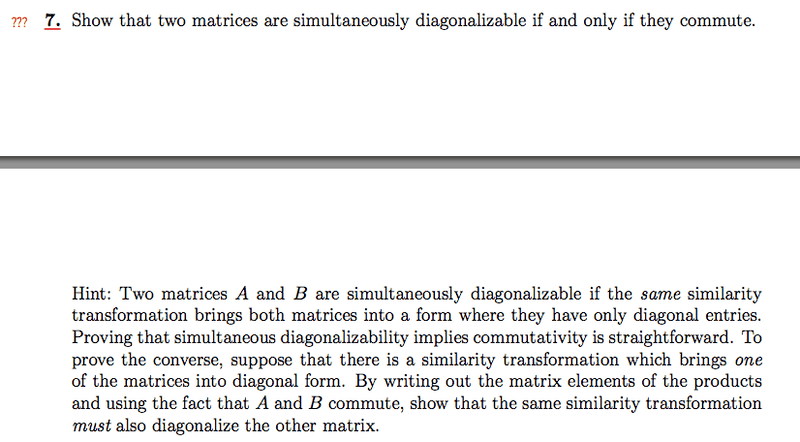
Answers:
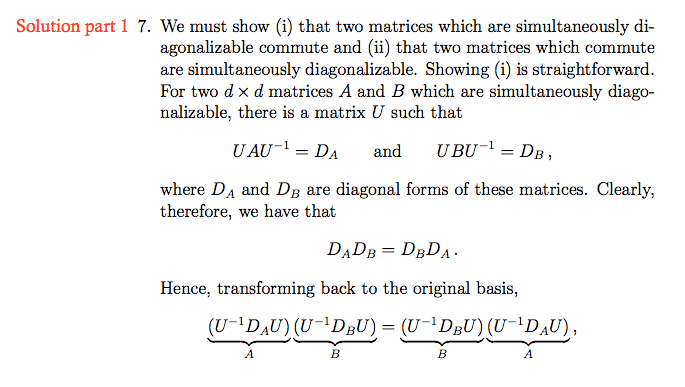
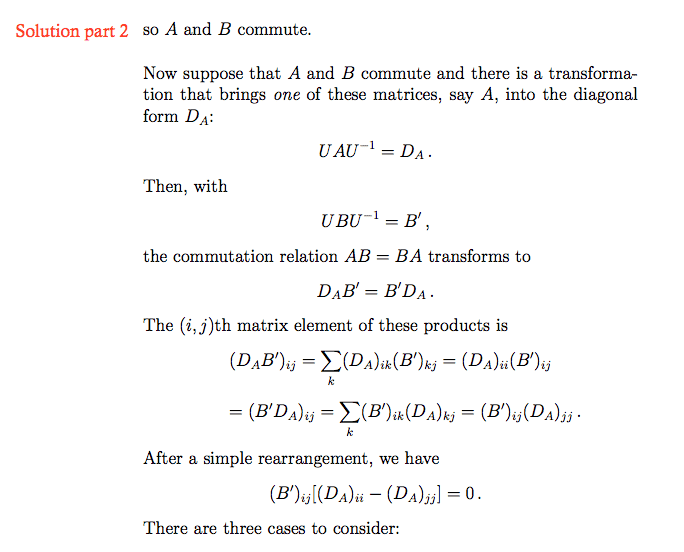
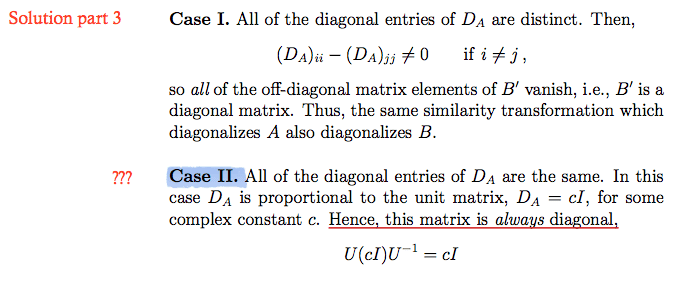
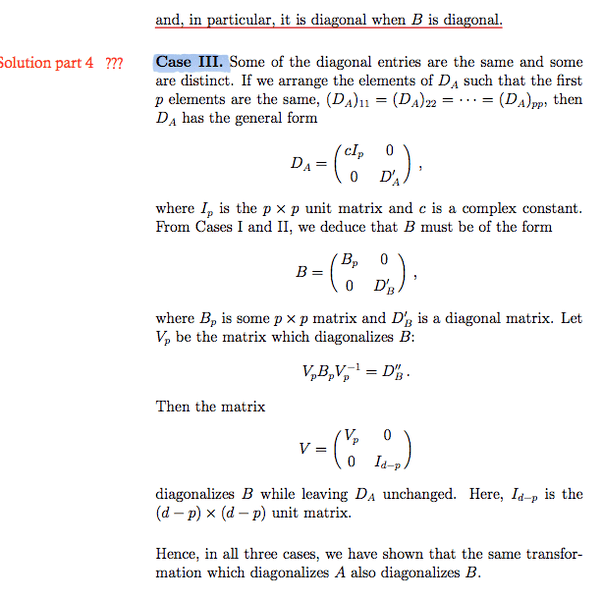
I get that D = c I means A must also be proportional to I but how does that mean B must be diagonal?
Question:
Answers: