Integral - cylindrical coordinates

[Chipset3600]

Hello, my best problem is about find the integration limits.
in cylindrical coordinates- where V is limited by the cylinder y^2+z^2=9 and the planes x = 0, y = 3x and z = 0 in the first octant.

 

[Ackbach]

Re: Integral- cylindrical coordinates

So, let's say that you have
\begin{align*}
z&=z\\
y&=r \sin(\theta) \\
x &= r \cos(\theta).
\end{align*}

You have several constraints. How about being inside the cylinder $y^{2}+z^{2}=9$? How about being in the first octant? How about being bound by the three planes? How could you restrict your three integration variables, $z,r,\theta$, to account for these constraints?

 

[Chipset3600]

Re: Integral- cylindrical coordinates

So, let's say that you have
\begin{align*}
z&=z\\
y&=r \sin(\theta) \\
x &= r \cos(\theta).
\end{align*}

z=0..3
y= 0..sqrt(9-y^2)
x=0..y/3
it is correct?

 

[Ackbach]

Re: Integral- cylindrical coordinates

Hmm. I'm not sure I agree with that.

Step one: determine in what order you're going to integrate. I'd recommend either
$$\iiint r\, dr\, d\theta\, dz$$
or
$$\iiint dz\, r\, dr\, d\theta.$$

Step two: each integral can have variables in the integration limits that appear outside the integral, but not inside. So you could have
$$\int_{0}^{1} \int_{0}^{z} \int_{0}^{\theta}r\, dr\, d\theta\, dz,$$
but not
$$\int_{0}^{\theta} \int_{0}^{z} \int_{0}^{1} r\, dr\, d\theta\, dz.$$
So what constraints does that place on you?

 

[Chipset3600]

Re: Integral- cylindrical coordinates

Hmm. I'm not sure I agree with that.

Step one: determine in what order you're going to integrate. I'd recommend either
$$\iiint r\, dr\, d\theta\, dz$$
or
$$\iiint dz\, r\, dr\, d\theta.$$

Step two: each integral can have variables in the integration limits that appear outside the integral, but not inside. So you could have
$$\int_{0}^{1} \int_{0}^{z} \int_{0}^{\theta}r\, dr\, d\theta\, dz,$$
but not
$$\int_{0}^{\theta} \int_{0}^{z} \int_{0}^{1} r\, dr\, d\theta\, dz.$$
So what constraints does that place on you?

I prefer the first suggestion.
is correct my plot: http://i.imgur.com/0KfKPLG.jpg
actually i guess my best problem is in 3D graph =/

 

[Ackbach]

Re: Integral- cylindrical coordinates

Not too bad. You also have a plane coming out from the $z$-axis, $y=3x$.

So if you're integrating w.r.t. $r$ first, what is it going to range from? You're allowed to have $r=r(\theta,z)$. Then you integrate w.r.t. $\theta$, and you're allowed to have $\theta=\theta(z)$. Finally, you integrate w.r.t. $z$, and $z$ must range from one number to another. What will you have?

 

[Chipset3600]

Re: Integral- cylindrical coordinates

Not too bad. You also have a plane coming out from the $z$-axis, $y=3x$.

So if you're integrating w.r.t. $r$ first, what is it going to range from? You're allowed to have $r=r(\theta,z)$. Then you integrate w.r.t. $\theta$, and you're allowed to have $\theta=\theta(z)$. Finally, you integrate w.r.t. $z$, and $z$ must range from one number to another. What will you have?

sorry didn't get the english, what is w.r.t?

 

[Ackbach]

Re: Integral- cylindrical coordinates

sorry didn't get the english, what is w.r.t?

My apologies: w.r.t. = "with respect to". It's a common phrase in calculus and analysis when taught in English.

 

[Chipset3600]

Re: Integral- cylindrical coordinates

My apologies: w.r.t. = "with respect to". It's a common phrase in calculus and analysis when taught in English.

Sorry but I am not able to follow the reasoning. Maybe the technical english don't help me, i just tried understand something in forum just interpreting the equations.

 

[Ackbach]

Re: Integral- cylindrical coordinates

Sorry but I am not able to follow the reasoning. Maybe the technical english don't help me, i just tried understand something in forum just interpreting the equations.

Ok, let me use math instead. You have the following:
$$\int_{a}^{b} \int_{\theta_{1}(z)}^{\theta_{2}(z)}
\int_{r_{1}(\theta,z)}^{r_{2}( \theta,z)} r \, dr\, d\theta \, dz.$$
What are the functions $r_{1},r_{2},\theta_{1},\theta_{2}$ and the constants $a,b$?

 

[Chipset3600]

Re: Integral- cylindrical coordinates

Ok, let me use math instead. You have the following:
$$\int_{a}^{b} \int_{\theta_{1}(z)}^{\theta_{2}(z)}
\int_{r_{1}(\theta,z)}^{r_{2}( \theta,z)} r \, dr\, d\theta \, dz.$$
What are the functions $r_{1},r_{2},\theta_{1},\theta_{2}$ and the constants $a,b$?

z= 0..3
theta= 0..pi/2
r= 0..3 or the radius will not be ever 3 depending of angle?

 

[Ackbach]

Re: Integral- cylindrical coordinates

I don't think you've quite got it yet. Your $r$ is going to have to depend on either $\theta$ or $z$ or both. You know that $r^{2}=x^{2}+y^{2}$, and that $\theta=\tan^{-1}(y/x)$. Can you use these relationships to find an upper bound for $r$? I would agree with the lower bound: $0<r$.

Try this: $y^{2}+z^{2}=3$, so $x^{2}+y^{2}+z^{2}=3+x^{2}$, and thus $r^{2}+z^{2}=3+x^{2}=3+r^{2}\cos^{2}(\theta)$. Or, more directly, you can say that since $y=r \sin(\theta)$, that $r^{2} \sin^{2}(\theta)+z^{2}=3$. Solving for $r^{2}$ yields that
$$r^{2}= \frac{3-z^{2}}{ \sin^{2}( \theta)}.$$
Can you continue from here?

 

[Chipset3600]

Re: Integral- cylindrical coordinates

I don't think you've quite got it yet. Your $r$ is going to have to depend on either $\theta$ or $z$ or both. You know that $r^{2}=x^{2}+y^{2}$, and that $\theta=\tan^{-1}(y/x)$. Can you use these relationships to find an upper bound for $r$? I would agree with the lower bound: $0<r$.

Try this: $y^{2}+z^{2}=3$, so $x^{2}+y^{2}+z^{2}=3+x^{2}$, and thus $r^{2}+z^{2}=3+x^{2}=3+r^{2}\cos^{2}(\theta)$. Or, more directly, you can say that since $y=r \sin(\theta)$, that $r^{2} \sin^{2}(\theta)+z^{2}=3$. Solving for $r^{2}$ yields that
$$r^{2}= \frac{3-z^{2}}{ \sin^{2}( \theta)}.$$
Can you continue from here?

So the radius go from 0 to sqrt[(3-z^2)/(sin^2(theta))]

 

[Ackbach]

Re: Integral- cylindrical coordinates

So the radius go from 0 to sqrt[(3-z^2)/(sin^2(theta))]

Right. Now, what are the limits on $\theta$? Hint: it'll be partly determined by the plane $y=3x$.

 

[Chipset3600]

Re: Integral- cylindrical coordinates

Right. Now, what are the limits on $\theta$? Hint: it'll be partly determined by the plane $y=3x$.

Can you show me the graph of bounded area please?

 

[Ackbach]

Re: Integral- cylindrical coordinates

Can you show me the graph of bounded area please?

Alas, I do not have very good graphing or scanning capabilities where I am. The plane $y=3x$ does not depend on $z$, so it'll look the same no matter what $z$ is. It will intersect with the $z$-axis, and come out of it at an angle with respect to the $x$-axis. Can you find what that angle is?

 

[Chipset3600]

Re: Integral- cylindrical coordinates

Alas, I do not have very good graphing or scanning capabilities where I am. The plane $y=3x$ does not depend on $z$, so it'll look the same no matter what $z$ is. It will intersect with the $z$-axis, and come out of it at an angle with respect to the $x$-axis. Can you find what that angle is?

from tan^-1(3) to pi/2?

 

[Ackbach]

Re: Integral- cylindrical coordinates

Close. I think it's $0$ to $\tan^{-1}(3)$.

 

[Chipset3600]

Re: Integral- cylindrical coordinates

Close. I think it's $0$ to $\tan^{-1}(3)$.

So the integral be: $$\int_{0}^{3}\int_{0}^{\arctan 3 }\int_{0}^{\sqrt{\frac{3-z^2}{sin^2(\theta)}}} rdr d\theta dz$$ ?? this equal to Zero :s

 

[Ackbach]

Re: Integral- cylindrical coordinates

I don't think the integral will be zero. You have
\begin{align*}
\int_{0}^{3} \int_{0}^{\tan^{-1}(3)} \int_{0}^{\frac{\sqrt{3-z^{2}}}{\sin(\theta)}}
r\, dr\, d\theta\, dz&=
\int_{0}^{3} \int_{0}^{ \tan^{-1}(3)} \left( \frac{r^{2}}{2} \right) \Bigg|_{0}^{ \frac{ \sqrt{3-z^{2}}}{ \sin( \theta)}}
d\theta\, dz\\
&=\frac{1}{2}\int_{0}^{3} \int_{0}^{ \tan^{-1}(3)} \frac{3-z^{2}}{\sin^{2}( \theta)}
d\theta\, dz\\
&= \frac{1}{2} \left( \int_{0}^{3}(3-z^{2})\,dz \right) \left( \int_{0}^{\tan^{-1}(3)} \csc^{2}( \theta) \, d\theta \right).
\end{align*}
Can you finish?

 

[Ackbach]

Re: Integral- cylindrical coordinates

Hmm. Not sure I can finish, actually. The $\theta$ integral doesn't converge.

Can you write down the original problem statement, word-for-word, please?

 

[Chipset3600]

Re: Integral- cylindrical coordinates

Hmm. Not sure I can finish, actually. The $\theta$ integral doesn't converge.

Can you write down the original problem statement, word-for-word, please?

Calculate the ∭zdV
In cylindrical coordinates: where V is limited by the cylinder y^2+z^2=9 and the planes x = 0, y = 3x and z = 0 in the first octant.

 

[Opalg]

Re: Integral- cylindrical coordinates

Calculate the ∭zdV
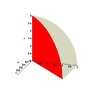
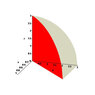
In cylindrical coordinates: where V is limited by the cylinder y^2+z^2=9 and the planes x = 0, y = 3x and z = 0 in the first octant.

I have kept away from this discussion because I could not see a convenient way of solving the problem using cylindrical coordinates. In fact, I think it is much better not to use them. Suppose instead that we look at a cross-section of $V$ at a fixed level of $z$. The cross-section looks like this:

View attachment 901

​

We must have $y$ lying between $-\sqrt{9-z^2}$ and $+\sqrt{9-z^2}$. We also need $x\geqslant0$. Next, the line $y=3x$ must mark the boundary of the cross-section. But which side of that line should $V$ be on? The wording of the problem does not make that clear, and that seems to be one of the reasons that this problem is causing so much discussion. But if $V$ is to be bounded, it is clear from the picture that it must lie to the left of the line, so that the cross-section is the pink shaded region in the diagram. This is a triangle whose height is $\sqrt{9-z^2}$ and whose width is one-third of that. Thus its area is $\frac16(9-z^2)$. Finally, since $V$ lies in the positive octant, we must have $0\leqslant z\leqslant 3$. It follows that $$\iiint z\,dV = \int_0^3 \tfrac16z(9-z^2)\,dz,$$ which is an easy integral.​

 

[Mathwebster]

Here's a 3D picture. Surfaces in 3D are hard for a lot of people to visualize. For me, I'd stick with Cartesian coords or go with a different parameterization than cylindrical polars. (Sorry it's so large - maybe someone can scale it down abit.
View attachment 902

 

[Ackbach]

Thanks much for the corrections from Opalg and the picture from Jester. So, if you must do the integral in cylindrical, the appropriate integral is
$$\int_{0}^{3} \int_{\tan^{-1}(3)}^{ \frac{ \pi}{2}} \int_{0}^{\frac{\sqrt{3-z^{2}}}{\sin(\theta)}}
r\, dr\, d\theta\, dz.$$
This time, the $\csc^{2}$ integral will converge.

 

[Chipset3600]

Thanks much for the corrections from Opalg and the picture from Jester. So, if you must do the integral in cylindrical, the appropriate integral is
$$\int_{0}^{3} \int_{\tan^{-1}(3)}^{ \frac{ \pi}{2}} \int_{0}^{\frac{\sqrt{3-z^{2}}}{\sin(\theta)}}
r\, dr\, d\theta\, dz.$$
This time, the $\csc^{2}$ integral will converge.

Now make's more sense, but the integral still equal to zero
View attachment 903

 

[Ackbach]

Oops. I'm still missing something. You were asked to compute $\iiint z \, dV$. You must insert a $z$ into the integrand.

 

[Chipset3600]

Oops. I'm still missing something. You were asked to compute $\iiint z \, dV$. You must insert a $z$ into the integrand.

Exactly... if y^2+z^2= 9 then z= sqrt(9-y^2)

Parametrizing, am i wrong if i say that z= sqrt(9-(rsin(theta))^2) ?

 

[Ackbach]

I don't think there's a need to parametrize. $z$ is an accepted variable already in cylindrical coordinates. Besides, the equation you're using is the equation of the portion of the cylinder which is enclosing the 3D region over which you are integrating. In other words, that equation does not hold over the entire region. So your integral is as follows:
\begin{align*}
\int_{0}^{3} \int_{\tan^{-1}(3)}^{ \frac{ \pi}{2}} \int_{0}^{\frac{\sqrt{3-z^{2}}}{\sin(\theta)}}
zr\, dr\, d\theta\, dz
&= \int_{0}^{3} z \int_{ \tan^{-1}(3)}^{ \frac{ \pi}{2}}
\left( \frac{r^{2}}{2} \right) \Bigg|_{0}^{ \frac{ \sqrt{3-z^{2}}}{ \sin( \theta)}} \, d \theta \, dz \\
&= \frac{1}{2} \int_{0}^{3} z \int_{ \tan^{-1}(3)}^{ \frac{ \pi}{2}}
\frac{3-z^{2}}{\sin^{2}( \theta)}\, d \theta \, dz \\
&= \frac{1}{2} \left( \int_{0}^{3}(3z-z^{3}) \, dz \right) \left( \int_{ \tan^{-1}(3)}^{ \frac{ \pi}{2}} \csc^{2}( \theta) \, d \theta \right).
\end{align*}

 

[Opalg]

Here's a 3D picture. Surfaces in 3D are hard for a lot of people to visualize. For me, I'd stick with Cartesian coords or go with a different parameterization than cylindrical polars. (Sorry it's so large - maybe someone can scale it down a bit.
View attachment 905

The question asked for a solution using cylindrical coordinates, and Jester's helpful diagram provides a clue for doing this. The usual way to transform Cartesian coordinates to cylindrical is to replace $x$ and $y$ by $r\cos\theta$ and $r\sin\theta$, and to leave $z$ alone. But this problem refers to the cylinder $y^2+z^2=9$, which has its axis in the $x$-direction, so it makes much more sense to replace $y$ and $z$ by $r\cos\theta$ and $r\sin\theta$, and to leave $x$ alone.

If we let $y = r\cos\theta$ and $z = r\sin\theta$, then it is clear from Jester's diagram that the region $V$ is given by

$0\leqslant\theta\leqslant \pi/2$,
$0\leqslant r\leqslant 3$,
$0\leqslant x\leqslant y/3 = \frac13r\cos\theta$.​

Therefore (doing the $x$-integral first) $$\begin{aligned} \iiint z\,dV &= \int_0^{\pi/2}\int_0^3\int_0^{\frac13r\cos\theta}r\sin\theta\,dx\,rdrd\theta \\ &= \int_0^{\pi/2}\int_0^3\tfrac13r^3\sin\theta\cos\theta \,drd\theta \\ &= \int_0^{\pi/2}\tfrac{27}4\sin\theta\cos\theta\,d\theta \\ &= \int_0^{\pi/2}\tfrac{27}8\sin(2\theta)\,d\theta \\ &= \biggl[-\tfrac{27}{16} \cos(2\theta) \biggr]_0^{\pi/2} = \frac{27}8.\end{aligned}$$ Happily, this agrees with with the answer obtained by my previous method.