I Properties of conditionally convergent series

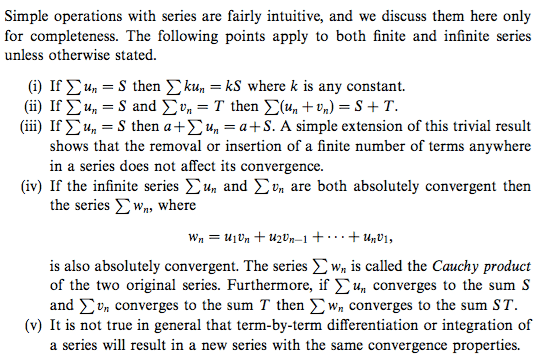
Do (i), (ii) and (iii) apply to conditionally convergent series as well? I feel like they don't. But the book seems to say that they do because it doesn't "state otherwise".

 

Yes there is. For (ii), it says ##u_1+u_2+...+v_1+v_2+...=u_1+v_1+u_2+v_2+...##.

There is no reordering in (iii). So we are left to prove that (ii) is true for conditionally convergent series.

If (ii) is true and if (i) is true for ##k=-1##, then (i) is true for all ##k##. Consider ##v_n=\frac{1}{2}u_n##, then using (ii), ##T=S-T##, so ##T=\frac{1}{2}S##. Similarly, we can prove (i) is true for all rational ##k##. Next, we use squeeze theorem to prove that (i) is true for all irrational ##k## too.

 

Is ##(u_1+u_2+...)+(v_1+v_2+...)=(u_1+v_1)+(u_2+v_2)+...## well defined? It says summing up all the ##u##'s and separately all the ##v##'s and then adding up the two sums is equal to summing up all the ##(u+v)##'s. So there is a rearrangement.

 

But the order we do the sum is different in the RHS from that in the LHS. Isn't this generally not allowed for conditionally convergent series?

 

This (elementary, common) proof of squeeze theorem does not use (i), (ii) or (iii): https://proofwiki.org/wiki/Squeeze_Theorem/Sequences/Real_Numbers

My use of squeeze theorem for proving (i) is also true for irrational ##k##:

Suppose ##k_1<k<k_2##, where ##k_1## and ##k_2## are rational and ##k## is irrational. As before, let ##S=\Sigma u_n##.

Since (i) is true for rational ##k_1##,
##\lim_{k_1\rightarrow k}\Sigma k_1u_n=kS##. (And similarly true for ##k_2##.)

Thus,
##kS=\lim_{k_1\rightarrow k}\Sigma k_1u_n\leq \Sigma ku_n\leq\lim_{k_2\rightarrow k}\Sigma k_2u_n=kS##.

By squeeze theorem (or otherwise),
##\Sigma ku_n=kS##.

 

##\lim_{k_1\rightarrow k}\Sigma k_1u_n=\lim_{k_1\rightarrow k}k_1\Sigma u_n##, since (i) is true for rational ##k_1##
##=k\Sigma u_n=kS##

 

I can't prove it directly.

Since ##\lim_{n\rightarrow\infty}\Sigma_{r=1}^nu_r=S##,

##\forall\epsilon>0, \exists N:\,\forall n>N, |\Sigma_{r=1}^nu_r-S|<\epsilon##

##|k|\,|\Sigma_{r=1}^nu_r-S|<|k|\epsilon##
##|k\,\Sigma_{r=1}^nu_r-kS|<|k|\epsilon##

but we want to show that

##|\Sigma_{r=1}^nku_r-kS|<|k|\epsilon,\,\forall n>N##.

This is true if ##k\,\Sigma_{r=1}^nu_r=\Sigma_{r=1}^nku_r,\,\forall n>N## including ##n=\infty##. But this is precisely what we want to prove.

 

##\sum_{i=0}^n k_1u_i\leq\sum_{i=0}^n k u_i\leq\sum_{i=0}^n k_2u_i##

By squeeze theorem,
##\lim_{n \to \infty} \sum_{i=0}^n k u_i=kS##

##\forall n>N## means we only need to consider finite values of ##n##?