Resultant vector field as sum of many sources

[Spinnor]

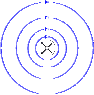
Let us have some localized density of sources, S, in a plane, each of which produces a localized circular vector field. Let us work in polar coordinates. Let the density of sources, S = Aexp(-r^2/a^2) and let each source have circular vector field whose strength is given by exp(-(r-r_i)^2/b^2) and is in a clockwise circular direction about the point r_i where r_i is the location in the plane of the ith source.

The constants a and b are the length scales in this problem, a determines how far from the origin most of the sources will be found and b tells us how localized the field of each source is, a is much larger than b. The constant A is a number density such that the integral of S over the whole plane gives the number of sources which is a very large number.

I think I know qualitatively what the resultant vector field is like, far from the origin the field goes to zero and at the origin the field goes to zero, where the density S changes the greatest in the radial direction one will find the strongest resultant field, and the resultant field is circular about the origin. But it would be nice to prove this.

I am only looking for qualitative results. Any hints on how to move forward would be appreciated. I suspect one might come up with an plausible verbal argument to show the above. Hopefully I have a well defined problem above.

Thanks for any help.

 

[mathman]

Your post is so wordy that it is very hard to read. Try to use more paragraphs at least.

 

[Spinnor]

Your post is so wordy that it is very hard to read. Try to use more paragraphs at least.

Done, hopefully more readable.

 

[mathman]

The description is too amorphous. You might better describe a particular situation and ask a defined question.

 

[Spinnor]

The description is too amorphous. You might better describe a particular situation and ask a defined question.

I know what I mean but the reader may not or may have to work too hard to try and figure out what I mean. I am sorry. What part is not clear?

Thanks.

 

[mathman]

The statement is clear enough, but the details are messy. Why do you think the field is zero at the origin? You need to clarify what a and b are supposed to do.

 

[Spinnor]

Why do you think the field is zero at the origin?

The distribution of sources is centered at the origin. If I plot the vector field of each source at the origin the vectors will point (nearly) symmetrically in all directions and their sum will be nearly zero though maybe not exactly zero, therefore the curl will be zero.

As far as the constants a and b in the problem they just gave the width of the gaussians and I wanted the width of the field of each source to be smaller than the width of the distribution of sources so we only need to consider the sources in some small region around some point of interest.

In the interim I think I have come up with an argument why the resultant field should be greatest where the density of sources changes the greatest in the radial direction. Say I am at a point where the density of sources changes the greatest in the radial direction. If I face the origin sources in front of me will have a resultant vector field pointing to my left and the sources behind me will have a resultant vector field pointing to my right. In some small region around me there are more sources in front of me then behind me so the resultant field will be to my left?

Thanks.

 

[mathman]

I won't comment on the details of what you are trying to do. However, as far as the field at the origin being zero or small, why can't you have a source at or near the origin?

 

[Spinnor]

I won't comment on the details of what you are trying to do. However, as far as the field at the origin being zero or small, why can't you have a source at or near the origin?

There are sources near the origin, because of the gaussian, S = Aexp(-r^2/a^2), a nearly uniform distribution of them. The field lines of each source circulate around each source. Something like the following but just the clockwise field lines.

(The field of each source is undefined at the center of each source so I guess I have to also set the field of each source to zero at the center of each source).

So it seems, by symmetry, the resultant field at the center of all the sources should generally cancel but not to exactly zero, how close to zero I don't know.

Thanks.

 

[Delta2]

if each infinitesimal source has finite contribution then the total field will be infinite at most points. Perhaps you meant to say that the field from each infinitesimal source $dq=S(\vec{r'})dA$ located at $\vec{r'}$ (where $dA$ the surface element of the plane), has strength $|d\vec{F(\vec{r})}|=f(\vec{r})dq=f(\vec{r})S(\vec{r'})dA$ where $f(\vec{r})=e^{-\frac{{(\vec{r}-\vec{r'})}^2}{b^2}}$? So that my point is that each infinitesimal source $dq=SdA$ must have infinitesimal contribution to the total field.

 

[Spinnor]

if each infinitesimal source has finite contribution then the total field will be infinite at most points.

The field strength of each source dies off like a gaussian, exp(-(r-r_i)^2/b^2), so depending on how quickly how the field of each source dies off, given by the length scale b, we only need consider those sources within some distance b of the point where we evaluate the field? So even though there may be many sources, as stated in the problem, the field at any point never blows up?

Thank you.

 

[Delta2]

Ok let's say this: We have to write down an integral, if we want to calculate the vector field $\vec{F}(\vec{r})$ from the source surface density $S(\vec{r'})$. The integral I have in mind is
$$\vec{F}(\vec{r})=\int_A \vec{f}(\vec{r},\vec{r'})S(\vec{r'})dA$$
$$dA=d^2\vec{r'}$$where A is the surface of integration (which is a plane in our case) and $\vec{f}(\vec{r},\vec{r'})$ is the field at point $\vec{r}$ due to an infinitesimal source located at point $\vec{r'}$. Do you agree with the above integral? If not what integral do you suggest?

 

[Spinnor]

Do you agree with the above integral?

That is marvously simple but I think that has to be it! Thank you. Does anyone disagree?

 

[Delta2]

That is marvously simple but I think that has to be it! Thank you. Does anyone disagree?

Ok I am glad we agree. But have in mind that $\vec{f}(\vec{r},\vec{r'})$ is not $e^{-\frac{{(\vec{r}-\vec{r'})}^2}{b^2}}$ for this problem, you have to find the expression for the field $\vec{f}$ that includes both the magnitude and direction, that is the full vector expression for the field from an infinitesimal source.