Silly current path thought experiment

[tim9000]

Hi, I want to varify something that I intuitively know (I think), that might not seem intuitive.

If an electrical current is circulating and then it's path splits in two, where there is a circulating current. The larger current (black) will have to pass through this loop; what I want to varify is that current A will split evenly and won't have any preference for half of the split path than the other, it won't care that half the current in the path is opposing it and half is in the same direction, Current A will take both paths evenly?

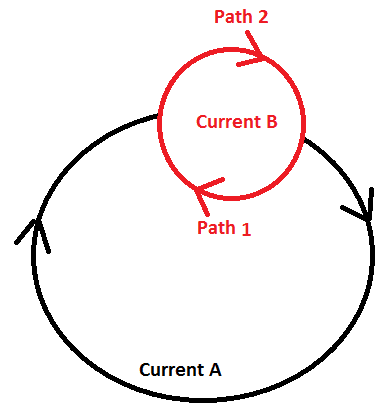
Then consider also that the circulating current in the small loop is half the magnitude of the larger current circulating through both loops. So then there is a NET current of nothing in Path 1, does this mean that half the electrons are drifting one way and half the other way, or they aren't really bias at all now? WIll Path 1 have twice the resistive heating of Path 2, or none?

Thanks

 

[jaus tail]

If a current is flowing in a circuit n you split the path in two n join them later then the current will split but both will go in same direction. depending on resistance of either half(split) the current distribution will change(more current through less resistive path). But there won't be a circulating current in the small loop. I don't think there would be a current B circulating in the inner loop.

 

[phinds]

This will depend entirely on the circuit. You'd have to have a power source (voltage or current) in the lower part of your loop for this to work at all the way you have drawn it. It would be a very particular case indeed for the circuit characteristics to be such that current A would split evenly. In fact, I'm not 100% sure you could even do it but I'm not going to try to create such a scenario since basically, I think your concept of how current works is flawed.

 

[Baluncore]

I don't think there would be a current B circulating in the inner loop.

You'd have to have a power source (voltage or current) in the lower part of your loop for this to work at all the way you have drawn it.

A circular current loop does NOT require a extra power source, an in-circuit transformer will do the job. The current will NOT always be shared equally, the ratio will be determined by the transformer turns ratio.
See the circuit here for an example.
https://www.physicsforums.com/threa...ng-number-question.803666/page-2#post-5048589

 

[tim9000]

A circular current loop does NOT require a extra power source, an in-circuit transformer will do the job. The current will NOT always be shared equally, the ratio will be determined by the transformer turns ratio.
See the circuit here for an example.
https://www.physicsforums.com/threa...ng-number-question.803666/page-2#post-5048589

The reason I actually ask this is because I know an electrical circuit is analogous to a magnetic path, what I'm wishing to determine is if you have a circulating flux (imagine all have equal reluctances, or in this case resistances) and another flux needs to circulate through them, will the flux divide evenly even though one path is adding and one is counter directional?

 

[tim9000]

If a current is flowing in a circuit n you split the path in two n join them later then the current will split but both will go in same direction. depending on resistance of either half(split) the current distribution will change(more current through less resistive path). But there won't be a circulating current in the small loop. I don't think there would be a current B circulating in the inner loop.

I know they will both go in the same direction with respect to the split of Current A, but just imagine that Current B is independantly caused and circulating, both paths are the same resistance. Its an idealised situation, take for granted that there is a specific circuit creating these conditions:
EDIT:
The current B in the diagram is separately caused, as is Current A, but in the region of Current B they overlap, so for path2 the total current in that branch is CurrentA + CurrentB

Path2 = Current A + Current B
Path1 = Current A + (-) Current B = 0

 

[phinds]

A circular current loop does NOT require a extra power source, an in-circuit transformer will do the job.

Well, yeah, but don't you think a tricky transformer loop is a bit much for what appears to be a circuits beginner? I mean, I don't think that really addresses the heart of the question he's asking, and how would that work with the circuit hooked up the way he shows? You'd have to short the output coils to the input coils and that would not go well.

 

[tim9000]

Well, yeah, but don't you think a tricky transformer loop is a bit much for what appears to be a circuits beginner? I mean, I don't think that really addresses the heart of the question he's asking, and how would that work with the circuit hooked up the way he shows? You'd have to short the output coils to the input coils and that would not go well.

Yeah, it's just an idealised situation, take for granted that there is a specific circuit creating these conditions, it needn't be a transformer.
I'm not reeeealy a beginner, I'm actually thinking about it if it were flux, but current is a fine analogy for flux. I know that if you have opposing flux then B will depend on the NET flux (will decrease compared to if they were in the same direction) that's what I'm getting at.
EDIT:
The current B in the diagram is separately caused, as is Current A, but in the region of Current B they overlap, so for path2 the total current in that branch is CurrentA + CurrentB

Path2 = Current A + Current B
Path1 = Current A + (-) Current B = 0

 

[milesyoung]

... what I want to varify is that current A will split evenly ...

Let's assume you just have three branches with some current sources and resistors. You'll have, for instance, I_A, I_B and -I_B in the current A path, path 1 and path 2, respectively.

By KCL in any node:
I_A + I_B - I_B = 0 ⇔ I_A = 0

I don't think your electrical analogy shows what you want it to.

 

[tim9000]

Let's assume you just have three branches with some current sources and resistors. You'll have, for instance, I_A, I_B and -I_B in the current A path, path 1 and path 2, respectively.

By KCL in any node:
I_A + I_B - I_B = 0 ⇔ I_A = 0

I don't think your electrical analogy shows what you want it to.

That's not right, what you wrote is according to the diagram which only shows the independent paths, good that you point that out, I should clarify that.
[KCL isn't according to the diagram] So The current B in the diagram is separately caused, as is Current A, but in the region of Current B they overlap, so for path2 the total current in that branch is CurrentA + CurrentB