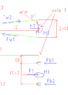
- #1
magwas
- 125
- 0
Homework Statement
In the steering arrangement below, axle 1 is a circular cross section rod.
It is hold by two bearings.
F1= 476 N
M1 = 15 Nm
Fw2 = 304.75 N, and we know that the moment imposed by Fw2 is equal to M1
l5 = 0.2 m
l8 = 0.1 m
The tensile yielding stress:
[tex]\sigma = 235 Mpa[/tex]
safety factor fs = 1.70
Calculate the radius of the rod.
https://www.physicsforums.com/attachment.php?attachmentid=23596&stc=1&d=1265629087
Homework Equations
The Attempt at a Solution
I believe I can solve it for the case when I neglect M1 and Fw2:
[tex] $ \\
$ Fb_{1} = Fb_{2} = F_{1}/2 \\
Fb_{1}=\frac{1}{2} F_{1}=238 N \\
\lvert{Fb_{1}}\rvert=238 N \\
Fb_{2}=\frac{1}{2} F_{1}=238 N \\
\lvert{Fb_{2}}\rvert=238 N \\
$ shear in axle 1 $ \\
shear = \begin{cases} 0 & \text{for}\: x < 0 \\Fb_{1} & \text{for}\: \operatorname{And}\left(0 \leq x,x < l_{5}\right) \\Fb_{1} - F_{1} & \text{for}\: \operatorname{And}\left(l_{5} \leq x,x < 2 l_{5},0 \leq x\right) \\\operatorname{And}\left(2 l_{5} \leq x,l_{5} \leq x,0 \leq x\right) & \text{for}\: Fb_{1} + Fb_{2} - F_{1} \end{cases} \\
moment = \begin{cases} 0 & \text{for}\: x < 0 \\Fb_{1} x & \text{for}\: \operatorname{And}\left(0 \leq x,x < 0.2 m\right) \\x \left(Fb_{1} - F_{1}\right) + 0.2 F_{1} m & \text{for}\: \operatorname{And}\left(0.2 m \leq x,x < 0.4 m\right) \\0.4 m \leq x & \text{for}\: x \left(Fb_{1} + Fb_{2} - F_{1}\right) + 0.2 F_{1} m - 0.4 Fb_{2} m \end{cases} \\
[/tex]
maximum bending moment in axle 1
[tex]
M_{a1}=Fb_{1} l_{5}=47.6 N m \\
\lvert{M_{a1}}\rvert=47.6 N m \\
[/tex]
Euler-Bernoulli beam equation with safety factor:
[tex]
\frac{\sigma}{fs} = \frac{M y}{Ix} \\
[/tex]
second moment of inertia in a circular beam:
[tex]
Ix = \frac{1}{4} \pi r^{4} \\
$ substituing the two equations together, $ r = y $ and $ M = M_{a1} \\
\frac{\sigma}{fs} = 4 \frac{M_{a1}}{\pi r^{3}} \\
$ solved for r :$ \\
r = \frac{2^{\frac{2}{3}} \sqrt[3]{M_{a1}} \sqrt[3]{fs} \sqrt[3]{\frac{1}{\sigma}}}{\sqrt[3]{\pi}} \\
r=\frac{2^{\frac{2}{3}} \sqrt[3]{M_{a1}} \sqrt[3]{fs} \sqrt[3]{\frac{1}{\sigma}}}{\sqrt[3]{\pi}}=0.000125670730105656 m 470^{\frac{2}{3}} \\
\lvert{r}\rvert=0.000125670730105656 m 470^{\frac{2}{3}} \\
r= 0.00759683528577m = 7.6 mm \\
[/tex]
Here is an attempt at the "M only" case:
Note that I am using the same [tex]\sigma[/tex] here. Is it okay?
torsional shearing stress with safety factor
[tex] \frac{\sigma}{fs} = \frac{M_{1} r}{J} [/tex]
polar moment of inertia for solid cylindrical shaft
[tex] J = \frac{1}{2} \pi r^{4} [/tex]
[tex]
r=\frac{\sqrt[3]{2} \sqrt[3]{M_{1}} \sqrt[3]{fs} \sqrt[3]{\frac{1}{\sigma}}}{\sqrt[3]{\pi}}=3.46762256685552 \times 10^{-5} m \sqrt[3]{30} \times 235^{\frac{2}{3}} \\
\lvert{r}\rvert=3.46762256685552 \times 10^{-5} m \sqrt[3]{30} \times 235^{\frac{2}{3}} \\
r = 0.0041031508172 m \\
[/tex]
I have no clue how to calculate with Fw2, and how to combine the whole thing together.