The Well-Ordering Principle for the Natural Numbers ....

[Math Amateur]

I am reading Ethan D. Bloch's book: The Real Numbers and Real Analysis ...

I am currently focused on Chapter 1: Construction of the Real Numbers ...

I need help/clarification with an aspect of Theorem 1.2.10 ...

Theorem 1.2.10 reads as follows:

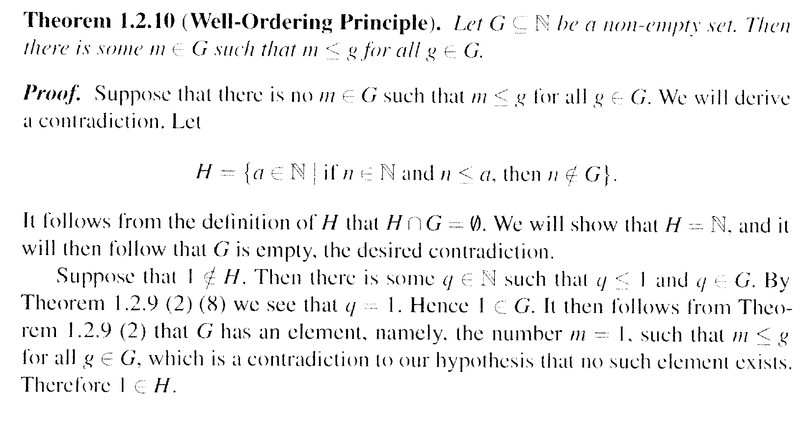
Towards the end (second last line) of the above proof by Bloch, we read the following:

" ... ... We now have a contradiction to the fact that no element such as $a + 1$ exists in $G$. ... ... "
I do not understand this remark ... as above $a + 1$ has earlier been proved to belong to $G$ ..

Can someone explain the remark "We now have a contradiction to the fact that no element such as $a + 1$ exists in $G$" in the context of the proof and explain just what is going on ... ...

Help will be much appreciated ...

Peter

 

[andrewkirk]

It is written in a very confusing way. Much too condensed, and no labelling of assumptions to allow justifications to be made clear.

The phrase 'no element such as $a+1$ exists in $G$' is particularly obscure, because we have no idea what the 'such as' means. It looks like it was intended to mean 'a minimum element', but it did not say that. Also, it should have not referred to the assumption that $G$ has no minimum as a 'fact'. It is an assumption, not a fact.

The statement about the contradiction would be much more clearly written as follows:

'Since $x$ was chosen as an arbitrary element of $G$, we conclude that every element of $G$ is greater than or equal to $a+1$, yet $a+1\in G$. That is, $a+1$ is a minimum element of $G$. That contradicts our assumption in the first line of our proof that $G$ has no minimum element. Hence we must reject the assumption that there is no minimum element.'

 

[jim mcnamara]

Just a thought. Ugh -- More than 50 years ago I took an upper level analysis class. The students and the prof all agreed that the text was impenetrable, with lots of unnecessary impediments for students. The book was dictated by the Math Department at Maryland U. The prof then put a series of texts on reserve, and gave us an under the covers syllabus using those texts. Point being: it's okay to use lots of resources, just do what you need to learn.

Consider losing that text, and find another one that clicks for you. A university library will have lots and lots of textbooks at varying levels. I know you are trying the "see what book A, book B, and book C say about theorem X you found in book Q" approach, which can work. As long as you get that one author's (proof of a) theorem may be a definition for the next writer, you will be okay. They are not always logically congruent in the eyes of a student.

 

[mathwonk]

this is another example of why you should always try prove things yourself instead of reading them. This result follows from the so called principle of complete induction. I.e. if 1 is contained in a subset S of N, and if for every n ≥ 1, the assumption that all integers from 1 to n inclusive are contained in S forces also n+1 to be contained in S, then S = N.

Using this try to prove every non empty subset T of N has a least element, as follows: first case is that 1 lies in T, then we are done and 1 is the desired east element. 2nd case is that 1 does not lie in T, and then try proof by contradiction. i.e. assume that T does not have a least element, and try to prove T is empty. This is equivalent to proving that S = N-T is equal to N. try that using complete induction.