- #1
Md. Abde Mannaf
- 20
- 1
Homework Statement
Homework Equations
The Attempt at a Solution
here is i am still stuck.
Don't use substitution, use Leibniz rule with α as the second variable.
Md. Abde Mannaf said:i know the Leibniz's rule. i solve many mathematical term . bt i cann't solve this math with Leibniz rule. i am still stuck
here. here limit is constant so 2nd and 3rd term will be zero.
See this, example 3.Zondrina said:if you are intending to use Leibniz rule. I for one have never seen an In\text{In} function before.
You can even cheat :) using wolframalpha.com.Md. Abde Mannaf said:Homework Statement
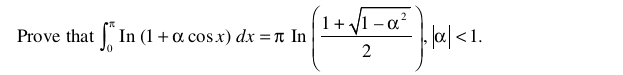
Homework Equations
The Attempt at a Solution

here is i am still stuck.
Zondrina said:I believe there are quite a few typos in the problem statement if you are intending to use Leibniz rule. I for one have never seen an ##\text{In}## function before.
The Leibniz rule in one dimension would be:
$$\frac{d}{dx} \int_{a(x)}^{b(x)} f(t) dt = f(b(x)) b'(x) - f(a(x)) a'(x)$$
EDIT: It would be more appropriate to call this the fundamental theorem.
Does no one read my posts :-)ehild said:You can even cheat :) using wolframalpha.com.
You can write your integral for ##f'(\alpha)## as ##\int_0^{\pi/2} + \int_{\pi/2}^{\pi}##, then change variables to ##x \leftarrow \pi -x## in the second integral, to getMd. Abde Mannaf said:i could not solve this math above analysis . i am trying to my best. but i am fail every time. and again try...
please see this and give me more idea to solve
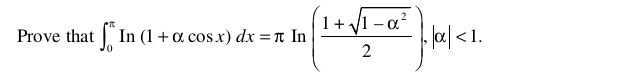
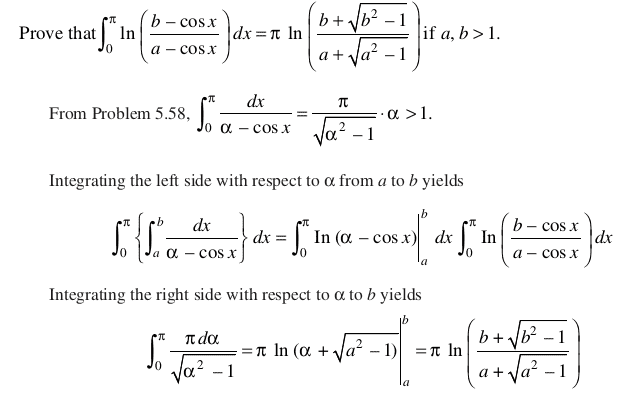
##\int \frac{1}{1+\alpha cos(x)} dx## is not that nasty.certainly said:Now let's do that daunting looking integral. Like I said before, this is a good problem.
First write ##\int_0^{\pi} \frac{1}{\alpha}-\frac{1}{(\alpha)(1+\alpha cos(x))} dx## for the original integral.
However ##\int \frac{1}{1+\alpha cos(x)} dx## itself is not nice, in-fact it's pretty nasty.
Yeah, your right. I didn't actually do the integral, I only saw the answer and thought, this might take some time.ehild said:##\int \frac{1}{1+\alpha cos(x)} dx## is not that nasty.
Use the identity ##\cos(x)=\frac{1-\tan^2(x/2)}{1+\tan^2(x/2)}##. Substitute u=tan(x/2), x=2arctan(u), ##dx=\frac{2}{1+u^2}du##. The integration limits become 0-->infinite.
Leibniz Rule, also known as the generalized product rule, is a mathematical formula used to differentiate a product of two or more functions. It can be used to find the derivative of complicated functions that cannot be easily differentiated using basic differentiation rules.
To use Leibniz Rule, follow these steps:
Leibniz Rule should be used when trying to find the derivative of a product of two or more functions. It is especially useful when working with complicated functions that cannot be easily differentiated using basic rules.
Leibniz Rule cannot be used to differentiate a product of an infinite number of functions. It also cannot be used if the functions are not differentiable or if they are not defined for all values of x.
Yes, Leibniz Rule can be extended to find higher order derivatives for products of multiple functions. The formula for finding the nth derivative is: d^n/dx^n(f(x) * g(x)) = f(x) * g^(n)(x) + f^(n)(x) * g(x) + sum(k=1 to n-1) [f^(k)(x) * g^(n-k)(x)].