Maximum Deviation Angle in Elastic Collision

[Saitama]

Homework Statement

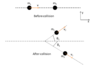
A particle of mass $m_1$ collides elastically with a stationary particle of mass $m_2$ ($m_1>m_2$). Find the maximum angle through which the striking particle may be deviated as a result of the collision.

(Ans. $\sin(\theta_{max})=m_2/m_1$)

Homework Equations

The Attempt at a Solution

I don't understand the problem. After the collision, both the particles continue the motion in the initial direction of motion. I don't see why the particles deviate. No geometry about the particles is specified.

Any help is appreciated. Thanks!

 

[TSny]

I think you're supposed to assume a glancing collision, rather than head-on.

 

[Saitama]

I think you're supposed to assume a glancing collision, rather than head-on.

Please have a look at the attachment. I have shown the situations before and after the collision.

Conserving momentum in x-direction,
$$m_1v=m_1v_1\cos(\theta_1)+m_2v_2\cos(\theta_2) (*)$$

Conserving momentum in y-direction,
$$m_1v_1\sin(\theta_1)=m_2v_2\sin(\theta_2) (**)$$

Since collision is elastic, energy conservation holds,
$$\frac{1}{2}m_1v^2=\frac{1}{2}m_1v_1^2+\frac{1}{2}m_2v_2^2 (***)$$

How do I solve this? :uhh:

EDIT:
From (**)
$$v_2=\frac{m_1v_1\sin(\theta_1)}{m_2\sin(\theta_2)}$$

Substituting this in (*)
$$m_1v=m_1v_1\cos(\theta_1)+m_2\cos(\theta_2)\frac{m_1v_1\sin(\theta_1)}{m_2\sin(\theta_2)}$$
$$\Rightarrow v=v_1\cos(\theta_1)+v\frac{v_1\sin(\theta_1)\cos(\theta_2)}{\sin(\theta_2)}=v_1\frac{\sin(\theta_1+\theta_2)}{\sin(\theta_2)}$$
$$\Rightarrow v_1=\frac{v\sin(\theta_2)}{\sin(\theta_1+\theta_2)}$$

Using this expression for $v_1$,
$$v_2=\frac{m_1}{m_2}\frac{v\sin(\theta_1)}{\sin(\theta_1+\theta_2)}$$

Substituting $v_1$ and $v_2$ in the energy equation and simplifying,
$$\sin^2(\theta_1+\theta_2)=\sin^2(\theta_2)+\frac{m_1}{m_2}\sin^2( \theta _1)$$
How do I solve after this? :(

 

[yands]

Ok initially the momentum has only x coordinates .
So you have to have zero y-component of momentum after the collision

 

[yands]

Can you try using sin(a+b) = Sin(a)cos(b)...

 

[Saitama]

Can you try using sin(a+b) = Sin(a)cos(b)...

I would rather refrain from using that here. It would lead to a very ugly expression.

And, the correct expansion is sin(a+b)=sin(a)cos(b)+cos(a)sin(b).

 

[voko]

I would approach this differently. Consider the change in momentum of the first particle. What is the square of its magnitude?

 

[Saitama]

I would approach this differently. Consider the change in momentum of the first particle. What is the square of its magnitude?

Change in momentum is $(m_1v_1\cos(\theta_1)-m_1v)\hat{i}-m_1v_1\sin(\theta_1)\hat{j}$. Square of the magnitude is,
$$m_1^2(v_1^2+v^2-2vv_1\cos(\theta_1))$$

How does this help?

 

[voko]

What is that equal to?

 

[Saitama]

What is that equal to?

Magnitude of impulse?

 

[voko]

What is that square equal to, given the conservation of momentum?

 

[Saitama]

What is that square equal to, given the conservation of momentum?

I still don't understand what you ask me here. It is equal to $m_1^2|\vec{v_1}-\vec{v}|^2$. I can't think of anything else.

 

[voko]

Conservation of momentum is $m_1 \vec {v} = m_1 \vec{v}_1 + m_2 \vec{v}_2$. You got $m_1^2 (\vec{v} - \vec{v}_1)^2$. It obviously is equal to $m_2^2 v_2^2$. Now use conservation of energy to get rid of $v_2$.

 

[Saitama]

Conservation of momentum is $m_1 \vec {v} = m_1 \vec{v}_1 + m_2 \vec{v}_2$. You got $m_1^2 (\vec{v} - \vec{v}_1)^2$. It obviously is equal to $m_2^2 v_2^2$. Now use conservation of energy to get rid of $v_2$.

I followed your suggestion and found $\vec{v_1}$ in terms of $\vec{v}$. What should I do next?

This is what I get:
$$\vec{v_1}=\vec{v}\frac{(m_1/m_2-1)}{(m_1/m_2+1)}$$

 

[voko]

Really? How did you do that?

After eliminating $v_2$, you were supposed to get a quadratic equation for $v_1$. Its analysis would solve the problem.

 

[Saitama]

Really? How did you do that?

After eliminating $v_2$, you were supposed to get a quadratic equation for $v_1$. Its analysis would solve the problem.

No, I did not get a quadratic.

Here's what I did:
I had
$$v_2^2=\frac{m_1^2(\vec{v}-\vec{v_1})^2}{m_2^2}$$
From the energy equation:
$$\frac{1}{2}m_1v^2=\frac{1}{2}m_1v_1^2+\frac{1}{2}m_2\frac{m_1^2(\vec{v}-\vec{v_1})^2}{m_2^2}$$
$$\Rightarrow v^2=v_1^2+\frac{m_1}{m_2}(\vec{v}-\vec{v_1})^2$$
$$\Rightarrow (\vec{v}-\vec{v_1})(\vec{v}+\vec{v_1})=\frac{m_1}{m_2}(\vec{v}-\vec{v_1})^2$$
$$\Rightarrow \vec{v}+\vec{v_1}=\frac{m_1}{m_2}(\vec{v}-\vec{v_1})$$
Solving this gives me the relation I posted. Is there a problem with cancelling out $(\vec{v}-\vec{v_1})$ from both the sides?

 

[voko]

Solving this gives me the relation I posted. Is there a problem with cancelling out $(\vec{v}-\vec{v_1})$ from both the sides?

You bet. You cannot cancel the square - that would mean going from scalars to vectors, and this cannot be done unambiguously. Instead, you need to expand that square, just like you did previously, and go from there.

 

[Saitama]

You bet. You cannot cancel the square - that would mean going from scalars to vectors, and this cannot be done unambiguously. Instead, you need to expand that square, just like you did previously, and go from there.

This is the quadratic I get:
$$v_1^2\left(\frac{m_1}{m_2}+1\right)-2\frac{m_1}{m_2}vv_1\cos(\theta_1)+v^2\left(\frac{m_1}{m_2}-1\right)=0$$

Since the quadratic has real roots, discriminant must be greater than or equal to zero. (is this a correct way to say it?)

Solving the inequality, I end up with
$$\sin(\theta_1) \leq \frac{m_2}{m_1}$$

Thanks voko! :)

Also, how did you come up with this method? I mean, dealing with vectors here really made the job a lot easier. How did you know that we should go with vectors? Usually in collision questions, I conserve momentum in x and y direction, dealing in vectors never hit me before.

 

[voko]

I actually prefer staying with vectors for as long as I can. In this case, additionally, you demonstrated that going component-wise results in some very nasty trigonometry. It is actually possible to continue with your original approach and massage the equation you got into something manageable, but that's too much algebra and very little physics.

Re the method I proposed, it occurred to me that the difference between the initial and final momenta will have only one angle involved, so one of the angles is eliminated early on. I cannot quite explain my train of thoughts, it somehow just dawned upon me :)

 

[Saitama]

I actually prefer staying with vectors for as long as I can. In this case, additionally, you demonstrated that going component-wise results in some very nasty trigonometry. It is actually possible to continue with your original approach and massage the equation you got into something manageable, but that's too much algebra and very little physics.

Re the method I proposed, it occurred to me that the difference between the initial and final momenta will have only one angle involved, so one of the angles is eliminated early on. I cannot quite explain my train of thoughts, it somehow just dawned upon me :)

Using vectors is a great idea, I too will try to use them as much as possible. Thanks a lot voko!