What is the area of this part of a circle?

[kaizokonpaku]

Homework Statement

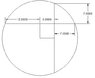
the figure is shown on the attachment. Find the area of the smallest part of the circle.

Homework Equations

area of circle, sector, segment

The Attempt at a Solution

I cannot use the said equations since the part of the circle is not with reference to the center. I cannot think also of any formulas for this kind of problem.

Please help.

 

[Saitama]

Consider that part as a part of another circle whose radius is 7.

 

[kaizokonpaku]

I already did that but the resulting answer is not the same with the given answer.

 

[Infinitum]

Consider that part as a part of another circle whose radius is 7.

Are you sure?

 

[Saitama]

I already did that but the resulting answer is not the same with the given answer.

Can you show us your steps and calculations?

Are you sure?

Not quite, but let me check it out once more.

 

[kaizokonpaku]

If that is another circle, then the area would be a quarter circle
A = (pi)(7^2) / 4 = 38.48. however, the given answer is 31.

 

[NascentOxygen]

Your first step should be to demonstrate that the corner of that square lies at the centre of the large circle.

 

[kaizokonpaku]

I do not get the first step.

 

[NascentOxygen]

the given answer is 31.

Lest anyone be misled into thinking this apparently is an easy problem you can solve in your head ...

You do need some trig and a calculator, and the answer is not an integer. I get 31.0126

 

[Curious3141]

I do not get the first step.

This can be done the hard way, or a very easy way.

Hard way: use trig to find certain angles, then prove that a geometric property of a circle (angle subtended by a chord at circumference = half angle subtended at centre). I was mucking around with this for a few minutes (and actually did it) before inspiration struck and I found the easy way.

Easy way: Reflect the figure vertically and superimpose the mirror images (getting two vertically stacked 5X5 squares). Now reflect that figure laterally, and superimpose the mirror images (to get four 5X5 squares). You will find that, with the dimensions given, one vertex of each of those squares will exactly touch the others, proving that that is the centre.

Now, after you've done that, the easiest way to find the required area is to visualise it as the sum of areas of a segment and a right triangle. Determining the angle subtended by the segment requires a little trig, but nothing too difficult.

 

[Infinitum]

This can be done the hard way, or a very easy way.

Hard way: use trig to find certain angles, then prove that a geometric property of a circle (angle subtended by a chord at circumference = half angle subtended at centre). I was mucking around with this for a few minutes (and actually did it) before inspiration struck and I found the easy way.

I did this. Couldn't post about it because I had to go out The answer doesn't come out to be exact, as NascentOxygen pointed out.

Easy way: Reflect the figure vertically and superimpose the mirror images (getting two vertically stacked 5X5 squares). Now reflect that figure laterally, and superimpose the mirror images (to get four 5X5 squares). You will find that, with the dimensions given, one vertex of each of those squares will exactly touch the others, proving that that is the centre.

Now, after you've done that, the easiest way to find the required area is to visualise it as the sum of areas of a segment and a right triangle. Determining the angle subtended by the segment requires a little trig, but nothing too difficult.

Interesting method, Curious(10^3)Pi!

 

[Curious3141]

Interesting method, Curious(10^3)Pi!

Thanks, but I only found it because I'm always trying to find the lazy way out. :tongue:

 

[kaizokonpaku]

I solved it and I got it using the easy way. Thanks for the help :)