At what angle does the normal force go to zero?

[romogirl]

Homework Statement

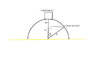
An ice cube is placed on top of an overturned spherical bowl of radius r, as indicated in the figure. If the ice cube slides downward from rest at the top of the bowl, at what angle θdoes it separate from the bowl? In other words, at what angle does the normal force between the ice cube and the bowl go to zero?

Homework Equations

∑F=ma
ΔK=-ΔU

The Attempt at a Solution

I drew a free body diagram, with the force on the ice cube from the Earth pointed straight down and the normal force perpendicular to the surface of the globe. I then summed the forces.
ΣF_y=W_y-N=ma_c
Based on trig, I know that W_y= cosθW = cosθmg.
Then: a_c= V_t²/r and the normal force is 0. Substituting all this in I get:
cosθmg=mV_t²/r
cosθ=V_t²/gr

So the next step would be to find for V_t.
ΔK =-ΔU
K_f =1/2mv²
K_O = 0
U_f = 0
U_O = mgr
1/2mv² = mgr
v² = 2gr

Plugging in this to the above:
cosθ=2gr/gr
cosθ=2
which isn't the answer.

I think I am making a mistake possibly in U_f, but I am not sure what exactly it would be. Would it be mgh, where h is the height it falls off? How would I find h then?

 

[azizlwl]

The drop should be less than r.

 

[stockzahn]

ΣF_y=W_y-N=ma_c
Based on trig, I know that W_y= cosθW = cosθmg.
Then: a_c= V_t²/r and the normal force is 0. Substituting all this in I get:
cosθmg=mV_t²/r
cosθ=V_t²/gr

So the next step would be to find for V_t.
ΔK =-ΔU
K_f =1/2mv²
K_O = 0
U_f = 0
U_O = mgr

Till this point it's correct.

1/2mv² = mgr

This equation generally is correct, but it gives you the velocity of the ice cube, when it hits the ground (as the entire potential energy is converted into kinetic energy). You have to find a connectedness between the velocity of the ice cube and the height above ground depending on the angle θ.

So instead of

1/2mv² = mgr

you need a function

1/2⋅m⋅v² = m⋅g⋅Δh(r,θ)

How could you express Δh in terms of radius r and angle θ?

 

[romogirl]

Till this point it's correct.
This equation generally is correct, but it gives you the velocity of the ice cube, when it hits the ground (as the entire potential energy is converted into kinetic energy). You have to find a connectedness between the velocity of the ice cube and the height above ground depending on the angle θ.

So instead of
you need a function

1/2⋅m⋅v² = m⋅g⋅Δh(r,θ)

How could you express Δh in terms of radius r and angle θ?

I made a right triangle, and I found that r-h=rcosθ, so then would h = r-rcosθ?

 

[romogirl]

I made a right triangle, and I found that r-h=rcosθ, so then would h = r-rcosθ?

I attached a picture of the drawing I made.

 

[stockzahn]

I made a right triangle, and I found that r-h=rcosθ, so then would h = r-rcosθ?

Yes, that's it.

 

[stockzahn]

I attached a picture of the drawing I made.

Looks good.

 

[romogirl]

Looks good.

This makes so much more sense, thank you!