Classical gas with general dispersion relation

[victor94]

i'm trying to understand the solution to this problem:

http://physweb.bgu.ac.il/COURSES/StatMechCohen/ExercisesPool/EXERCISES/ex_2065_sol_Y13.pdf
(link to the problem and the solution of it)

All my questions come from the partition function:

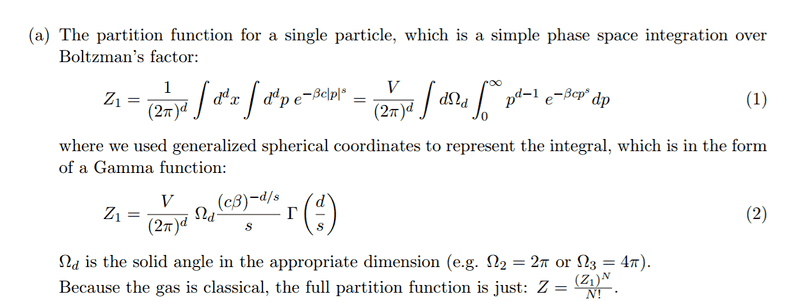
1) From where the term (2*pi)^d comes from?, I think is like a normalization factor, but I'm not sure.


2) The Volume (V) should be V^d, because is the volume of a particle of d dimension, but in the solution is just "V" , I don't understand why.3) The solid angle is used to simplify the integral and it comes from the volume of a sphere of d-dimensionm. I don't understand how to use that volume of the sphere to this specific problem.

4) Where is this problem used, or is it just a theoretical problem?

5) The last question is a conceptual one, how the phase space looks in a d-dimension, I don't understand this concept.

Any help in this questions will be appreciated.
Thanks in advance.

 

[Charles Link]

The way I learned it, (the Statistical Physics book by F. Reif), $e^{ik_x x}=e^{i k_x(x+L_x)}$ for periodic boundary conditions. This means in counting states in k-space in 3 dimensions, you get the number of states $\Delta N = \Delta^3 n=V \Delta^3 k/(2 \pi)^3$. ($k_x L_x=n_x 2 \pi$, $k_y L_y=n_y 2 \pi$, etc. from the periodicity requirement). Since $p=\hbar k$, this will also put ah $\hbar^3$ in the denominator of the $Z$ function which counts the states and multiplies by the Boltzmann factor $e^{-E/(kT)}$.