- #1
Dassinia
- 144
- 0
Hello
1. Homework Statement
Consider fk N*→N, k≥0
fk(n)=Σ jk n>0
We're looking to establish these identities, using a combinatorial argument
fk(n)=n if k=0
fk(n)= 1/(k+1) [ nk+1 + Σ ( i , k+1) (-1)k+1-ifi(n) ] if k>0
the sum is about i from i=0 to i=k-1 and (i, k+1) is the combination.
Consider the experience of k+1 drawing with handoff from a box of n balls numbered
Ej : the maximum of the values obtained on the k+1 draw is j , j=1,...,n
Fj : The drawing are made on {1,...,j} j=1,...,n
Fo=
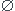
1. Find |Ω|
2. Write Ω as an operation on sets E1,...,En
3. Write Ej as an operation on sets Fj-1, Fj j=1...n
4. Find |Fj| j=0...n
5. Find |Ej| j=1...n
6. Find |Ω| through the calculation of |Ej| j=1...n and using the binomial theorem
(x+y)n=∑(k, n)xn-kyk
1. |Ω|=nk+1
2. Ω=∩ Ej for j=1,...,n
3. I just don't get what Fj represents..?
Thanks
1. Homework Statement
Consider fk N*→N, k≥0
fk(n)=Σ jk n>0
We're looking to establish these identities, using a combinatorial argument
fk(n)=n if k=0
fk(n)= 1/(k+1) [ nk+1 + Σ ( i , k+1) (-1)k+1-ifi(n) ] if k>0
the sum is about i from i=0 to i=k-1 and (i, k+1) is the combination.
Consider the experience of k+1 drawing with handoff from a box of n balls numbered
Ej : the maximum of the values obtained on the k+1 draw is j , j=1,...,n
Fj : The drawing are made on {1,...,j} j=1,...,n
Fo=
1. Find |Ω|
2. Write Ω as an operation on sets E1,...,En
3. Write Ej as an operation on sets Fj-1, Fj j=1...n
4. Find |Fj| j=0...n
5. Find |Ej| j=1...n
6. Find |Ω| through the calculation of |Ej| j=1...n and using the binomial theorem
Homework Equations
(x+y)n=∑(k, n)xn-kyk
The Attempt at a Solution
1. |Ω|=nk+1
2. Ω=∩ Ej for j=1,...,n
3. I just don't get what Fj represents..?

Thanks