Composition Series of Modules .... Remarks by Cohn

[Math Amateur]

I am reading "Introduction to Ring Theory" by P. M. Cohn (Springer Undergraduate Mathematics Series ... )

In Chapter 2: Linear Algebras and Artinian Rings, on Page 61 we find a definition of a refinement of a chain and a definition of a composition series.

The relevant text on page 61 is as follows:

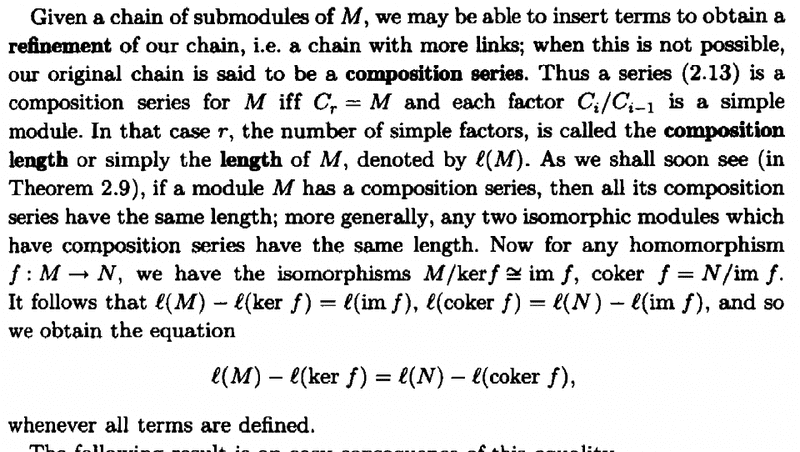
In the above text, Cohn indicates that a refinement of a chain (added links) is a composition series for a module $M$, but then goes on to to characterise a composition series for a module $M$ as a chain in which $C_r = M$ for some positive integer $r$, and for which $C_i/C_{i-1}$ is a simple module for each $i$.

So then, is Cohn saying that if a refinement is not possible, then it follows that $C_r =M$ for some $r$ and $C_i/C_{i-1}$ is a simple module for each $i$? If so, why/how is this the case?

Peter

 

[andrewkirk]

In the above text, Cohn indicates that a refinement of a chain (added links) is a composition series for a module $M$,

He's not saying that. A composition series is what you have when you have done all the refinement possible, and can do no more.

Since he says 'if a module M has a composition series...' I imagine there must be some modules for which any chain can always be further refined, so the point where no further refinement is possible can never be reached, hence there is no composition series, and no 'length'. I would guess that such modules have uncountable cardinality.

A chain for which no refinement is possible is a composition series, and the 'big' end of the chain is the full module $M$, which is $C_l$ where $l$ is the 'length' of $M$.

And $C_i/C_{i-1}$ is simple in a composition series because if it was not, there would be some submodule $C_*$ of $C_i$ such that $C_*/C_{i-1}$ was a proper, non-trivial submodule of $C_i/C_{i-1}$, so we could make a further refinement of the chain by inserting $C_*$ between $C_{i-1}$ and $C_i$.

 

[Math Amateur]

Thanks Andrew ... I really appreciate your help ...

... but, can you help a bit more ... I was trying to formulate a rigorous and formal proof of the statement, as you put it ...

" ... ... And Ci/Ci−1 is simple in a composition series because if it was not, there would be some submodule C∗ of Ci such that C∗/Ci−1 was a proper, non-trivial submodule of Ci/Ci−1, so we could make a further refinement of the chain by inserting C∗ between Ci−1 and Ci... ... "

I am unable to formulate an explicit, rigorous and formal proof of this statement ... can you help ...

Peter

 

[andrewkirk]

If $C_i/C_{i-1}$ is not simple then by definition it must contain a non-trivial, proper submodule, call it $B$. Define the following subset of $C_i$:
$$C_*\equiv\{c\in C_i\ |\ c+C_{i-1}\in B\}$$
I claim that $C_*$ is a submodule of $C_i$ that is different from both $C_i$ and $C_{i-1}$. Proving that involves three things.

1. Prove that $C_*$ is a proper subset of $C_i$. This is easy to do based on the fact that $B$ is a proper subset of $C_i/C_{i-1}$. Just pick an element $c+C_{i-1}\in C_i/C_{i-1}$ that is not in $B$ and reason in a couple of steps that $c$ cannot be in $C_*$.

2. Prove that $C_{i-1}$ is a proper subset of $C_*$. This is easy to do based on the fact that $B/C_{i-1}\neq 0$. Just pick a non-zero element $c+C_{i-1}\in B$ and observe that $c$ cannot be in $C_{i-1}$.

3. Prove that $C_*$ is a submodule. Note that most of the submodule axioms are inherited from $C_i$. The ones that need additional, explicit proof are closure under addition and ring multiplication, the existence of a zero and an additive inverse. The proofs are short and straightforward. It's mostly just a case of keeping one's notation in order. See how you go, and shout out if you get stuck.

 

[Math Amateur]

Hi Andrew ... thanks for the help ...

Will try (1) ...

Changing your notation a bit ... so there are not too many c's ... let

$D_* \equiv \{ d \in C_i \ | \ d + C_{i -1} \in B \}$

Now prove (1) $D_*$ is a proper subset of $C_i$

Choose an element $d' + C_{i -1} \in C_i / C_{i -1}$ that is not in $B$ (where $B$ is a proper submodule of $C_i / C_{i -1}$ )

Now the natural or canonical $R$-homomorphism for modules $\pi \ : \ C_i \longrightarrow C_i / C_{i -1}$ where $\pi \ : \ h \mapsto h + C_{i -1}$ for $h \in C_i$ is surjective ... ...

So, now, consider the pre-image of $d' + C_{i -1}$, namely $\pi^{-1} \{ d' + C_{i -1} \}$ ... ... (See Figure 1 below)

This pre-image contains d' among other elements of $C_i$ ... so $d' \in C_i$ ... ...

BUT ... since $d' + C_{i -1} \notin B$, then we have $d' \notin D_*$ by the definition of $D_*$ ...

Hence $D_*$ is a proper subset of $C_i$

Can you please critique my analysis above ... pointing out errors or shortcomings ...

Peter

=================================================================

Figure 1 is as follows:

*** EDIT ***

I am getting concerned (the more I think about it) that my Figure 1 implies some erroneous things ...

It implies that an element, day, where $d'_1$ also belongs to $C_i$ is possible ... ...

BUT ... ... I suspect that $d'_1 \in \pi^{-1} \{ d' + C_{i-1} \}$ is disjoint from $C_{i-1}$...

Is that correct? ... if it is what is the exact reason as I am not sure ...I also suspect that $d'_1 \in \pi^{-1} \{ d' + C_{i-1} \}$ is also disjoint from $D_*$ ... is that correct ... but why ...? (the above are only suspicions ...)

... ...

 

[fresh_42]

Firstly, there's nothing wrong in what Andrew said.
I just want to give you another approach. (Which in truth isn't one because it's just another way to state it.)

You have a composition series $0 ⊂ C_1 ⊂ ... ⊂ C_n ⊂ M$ of $M$ defined by: no further refinement possible.
To show: the quotient modules $C_i / C_{i-1}$have to be simple, i.e. they do not have a proper submodule.

Now apply the correspondence theorem 6.22 to $C_i$ (as $M$ in 6.22) and $C_{i-1}$ (as $T$ in 6.22).

Assume you have a 'subquotientmodule' $0 = C_{i-1} / C_{i-1} ⊆ S / C_{i-1} ⊆ C_i / C_{i-1}$
($S$ here is $C_*$ in Andrew's notation, $D_*$ in yours; but I do not require $S$ to be proper. I've chosen $S$ as it is in 6.22. Here $S = S'$.)
According to theorem 6.22 you get a corresponding chain $C_{i-1} ⊆ S ⊆ C_i$ of submodules. However, the series $0 ⊂ C_1 ⊂ ... ⊂ C_n ⊂ M$ cannot be refined which forces $S$ to be $S = C_{i-1} ⇔ S / C_{i-1} = 0$ or $S = C_i ⇔ S / C_{i-1} = C_i / C_{i-1}$, i.e. there is no proper submodule in $C_i / C_{i-1}$, which we call to be a simple module.

Since 6.22 constitutes a bijection this proof goes the other way round. If all quotientmodules of a series of submodules are simple, then the series cannot be refined, i.e. it's a composition series.

Theorem 6.22 also guaranties that the length of a module is well-defined, i.e. the maximal index where the composition series cannot be refined any further. It cannot have two composition series of different lengths.

P.S.: Just seen afterwards: As far as I can see, Andrew wrote you that on Friday Tea culpa.
But it fulfills me with joy to see your compassion and efforts to work it out carefully. It reminds me on my own troubles I once had getting used to the concepts. Once you are firm in it it'll appear to you far easier than you might think it is now. It's just a matter of "playing" with it. And never be afraid of asking, Peter. (You're at a pretty save place down there )

P.P.S.: Oops, just seen. Andrew from Sydney is. Not as save you are as think I was ...