Energy of parallel plate capacitor

[Pushoam]

Energy of a parallel plate capacitor with charge Q and potential difference V can be calculated in two ways:
1)Work done in bringing charge dq from infinty to the capacitor when there is potential V= q/C on the positive plate and V= 0 on the negative plate is dW = (q/C)dq
integrrating from 0 to Q gives W = (1/2)Q²/C

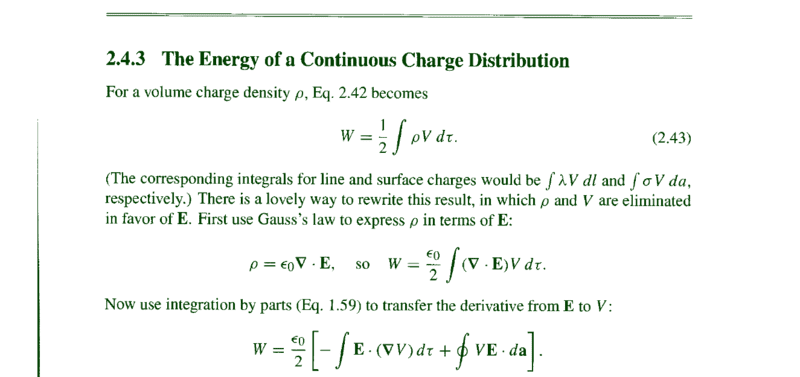
2)Using eqn.(2.43) of Griffith's Introduction to electrodynamics , 3 ed.,
W= $\frac 1 2\int_Γ ρV \, dΓ =\frac 1 2 \int_{q=0}^Q V \, dq = \frac 1 2 \int \frac q C \, dq = \frac 1 2 \frac {Q^2} {2C} = \frac 1 4 \frac {Q^2} {C}$,
where Γ is the volume over which the integration has to be done
How to decide which method is right?
Can anyone tell me why there is a factor of (1/2) in the above equation?

 

[mjc123]

You are applying 2.43 wrongly. It is not considering adding charge to the capacitor, and changing V; it is considering the fully charged state, and integrating ρV over the volume. Therefore ∫ρVdΓ is not equal to ∫Vdq; q is constant. Assuming V is constant over the volume, ∫ρVdΓ = QV = Q²/C, so W is the same as for method 1.

 

[Pushoam]

Thanks for reply,

Assuming V is constant over the volume, ∫ρVdΓ = QV = Q2/C, so W is the same as for method 1.

V is not constant over the volume.It is E which is constant.
dW = ρV dΓ is the work done by me when I bring a charge dq = ρ dΓ from a point where potential is zero to the point where potential is V.
In case of a capacitor, I am bringing a charge dq from negative plate where potential is V= q/C, where q is charge of the capacitor at this time.
Hence, ρV dΓ = q/C dq .
What is wrong with this argument?

According to what you said is,
ρ = Qδ(y)δ(z)[ δ(x-d) - δ(x)],
where the positive plate is at (d,0,0) and negative plate is (0,0,0).
Now
$\frac 1 2\int_Γ ρV \, dΓ =\frac 1 2 \int_Γ Qδ(y)δ(z)[ δ(x-d) - δ(x)] V \, d Γ= \frac 1 2 QV$

 

[mjc123]

Integrating over the volume (where ρ and V are the final charge density and potential) is not equivalent to integrating over the charge as you charge up. (And V is constant over the volume of a conductor, E is zero.)

In case of a capacitor, I am bringing a charge dq from negative plate where potential is V= q/C, where q is charge of the capacitor at this time.
Hence, ρV dΓ = q/C dq .
What is wrong with this argument?

I think what is wrong with it is that V varies with q during charging. But V is constant (the final potential) in 2.43. Perhaps it would be clearer if you used, say, v and V analogously to q and Q. So V = Q/C is the final potential at final charge Q, and v = q/C is the instantaneous potential at charge level q. Hence your equation would be ρv dΓ = q/C dq, whereas the integral in 2.43 is ρV dΓ, which is not the same thing. The factor of 1/2 arises in exactly the same way as in method 1, where ∫v(q)dq = 1/2 QV. Since ∫ρdΓ = Q, W = 1/2 ∫ρVdΓ.

 

[Pushoam]

Now, I got it.
Thank you.
I was mistaking V for v.