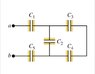
- #1
mathnoobie
- 63
- 0
Homework Statement
In the figure, C1 = C5 = 8.0 microF and C2 = C3 = C4 = 4.2 microF . The applied potential is Vab = 230V
Homework Equations
1/Ceq=1/C1+1/C2+...+1/Cn(for series connections)
Ceq=C1+C2+...+Cn
The Attempt at a Solution
The answer is 2.4x10^-6
So far I have tried to set C1 to C5 all in series, but that gives me the wrong solution.
I'm not sure what to do, could anyone hint me in the right direction?