- #1
Comeback City
- 417
- 68
Hello everyone!
Today in my BC Calculus (calc 2) class, we spent time trying to find ways to integrate the same function (all methods we're fluent with, but are reviewing for the upcoming AP test)...
(I apologize in advance that I do not know how to use LaTex, but am trying to learn)
f(x) = 1/[(e^x)+1]
Overall, we were able to integrate using simple methods such as trigonometric substitution, u-substitution, partial fraction decomposition, and with another algebraic method.
Being me, I didn't think this was enough, and attempted to solve this using a series. Below is my work...
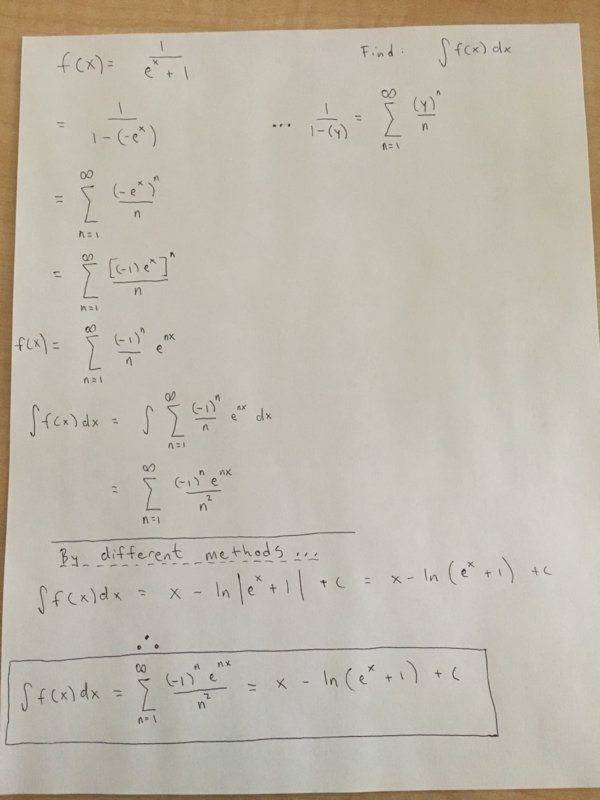
My question... Is there a way to continue this series and prove it is equivalent to the algebraic form found using the other integration methods? That is, besides just saying they come from the same integral.
Thank you in advance
Today in my BC Calculus (calc 2) class, we spent time trying to find ways to integrate the same function (all methods we're fluent with, but are reviewing for the upcoming AP test)...
(I apologize in advance that I do not know how to use LaTex, but am trying to learn)
f(x) = 1/[(e^x)+1]
Overall, we were able to integrate using simple methods such as trigonometric substitution, u-substitution, partial fraction decomposition, and with another algebraic method.
Being me, I didn't think this was enough, and attempted to solve this using a series. Below is my work...
My question... Is there a way to continue this series and prove it is equivalent to the algebraic form found using the other integration methods? That is, besides just saying they come from the same integral.
Thank you in advance


