Further Questions on Computations in Coordinates - Lee, Ch 3

[Math Amateur]

I am reading John M. Lee's book: Introduction to Smooth Manifolds ...

I am focused on Chapter 3: Tangent Vectors ...

I have some further questions concerning Lee's conversation on computations with tangent vectors and pushforwards ...

The relevant conversation in Lee is as follows:

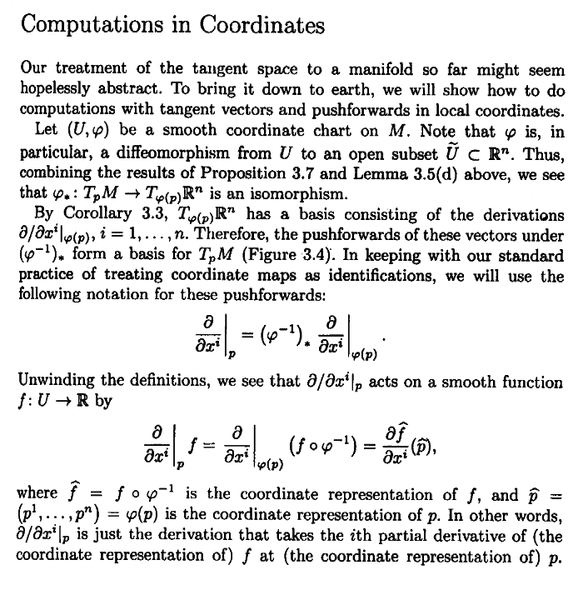
In the above text we read:

" ... ... we see that $\phi_* \ : \ T_p M \longrightarrow T_{ \phi(p) } \mathbb{R}^n$ is an isomorphism ... ... "

and then further ...

" ... ... $T_{ \phi(p) } \mathbb{R}^n$ has a basis consisting of all derivations $\frac{ \partial }{ \partial x^i } |_{\phi(p)} \ , \ i = 1, \ ... \ ... , n$. Therefore the pushforwards of these vectors under $( \phi^{-1} )_*$ form a basis for $T_p M$ ... ... "Question 1

Is $( \phi^{-1} )_*$ the inverse of $\phi_*$ and hence the isomorphism from $T_{ \phi(p) } \mathbb{R}^n$ to the tangent space $T_p M$?

Why isn't the inverse $( \phi_* )^{-1}$ ?Question 2

Since $( \phi^{-1} )_* \ : \ T_{ \phi(p) } \mathbb{R}^n \longrightarrow T_p M$ and we know that $T_{ \phi(p) } \mathbb{R}^n$ is a vector space ... then since $( \phi^{-1} )_*$ is an isomorphism ... then ... $T_p M$ is a vector space ... is that correct? ... ...

Hope someone can help ... ...

Peter

 

[lavinia]

Question 1

Is $( \phi^{-1} )_*$ the inverse of $\phi_*$ and hence the isomorphism from $T_{ \phi(p) } \mathbb{R}^n$ to the tangent space $T_p M$?

Why isn't the inverse $( \phi_* )^{-1}$ ?

They are the same. But the point is that the inverse mapping gives you the inverse differential. This is just the Chain Rule.

Question 2

Since $( \phi^{-1} )_* \ : \ T_{ \phi(p) } \mathbb{R}^n \longrightarrow T_p M$ and we know that $T_{ \phi(p) } \mathbb{R}^n$ is a vector space ... then since $( \phi^{-1} )_*$ is an isomorphism ... then ... $T_p M$ is a vector space ... is that correct? ... ...

yes but you already know that it is a vector space. Otherwise what would isomorphism mean?

 

[Math Amateur]

They are the same. But the point is that the inverse mapping gives you the inverse differential. This is just the Chain Rule.
yes but you already know that it is a vector space. Otherwise what would isomorphism mean?

Thanks Lavinia ... Needed that confirmation For confidence in what I am doing ...

Thanks again ...

Peter