- #1
FallenApple
- 566
- 61
So I'm reading the first page of Rudin. This is right after proving that there is no rational solution to x^2=2.
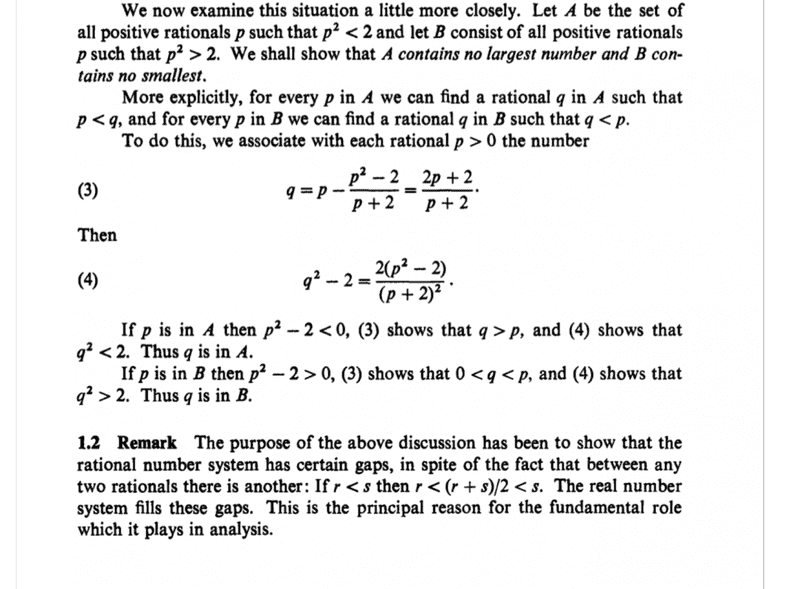 How does this show that the rational number system has gaps? All it shows is that A has no upper bound and B has no lower bound. Is it really necessary to have two sets A&B perfectly glued together? It seems to show that the real number line has gaps(if unfilled), but not the rational number line.
How does this show that the rational number system has gaps? All it shows is that A has no upper bound and B has no lower bound. Is it really necessary to have two sets A&B perfectly glued together? It seems to show that the real number line has gaps(if unfilled), but not the rational number line.
Also, proving there is no rational number x such that x^2=2 is different from saying that there exists a number x
such that I can take the square of it and get 2.
Also, proving there is no rational number x such that x^2=2 is different from saying that there exists a number x
such that I can take the square of it and get 2.
Last edited: